Samacheer Kalvi 12th Maths Guide Chapter 2 Complex Numbers Ex 2.8

Tamilnadu State Board New Syllabus Samacheer Kalvi 12th Maths Guide Pdf Chapter 2 Complex Numbers Ex 2.8 Textbook Questions and Answers, Notes.

Tamilnadu Samacheer Kalvi 12th Maths Solutions Chapter 2 Complex Numbers Ex 2.8

Question 1.
If to ω ≠ 1 is a cube root of unity, then show that

Solution:
L.H.S

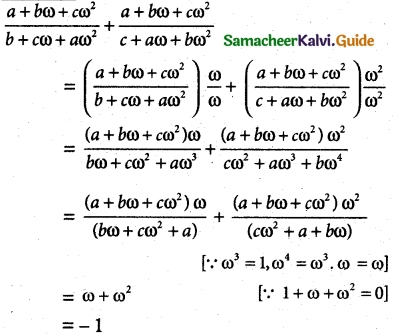
Question 2.
Show that

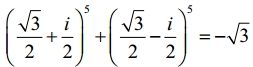
Solution:

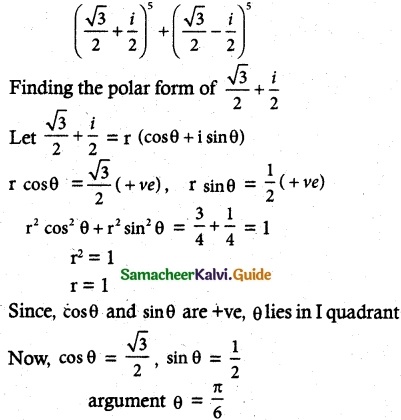
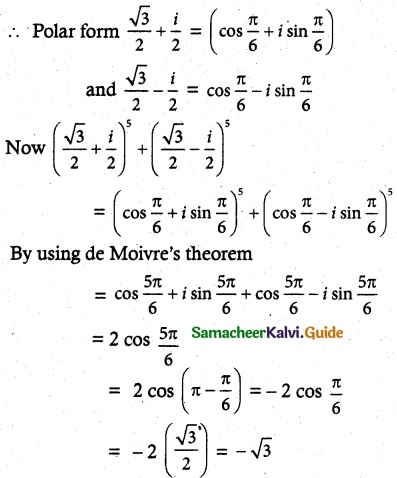
Question 3.

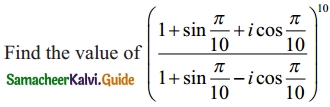
Solution:


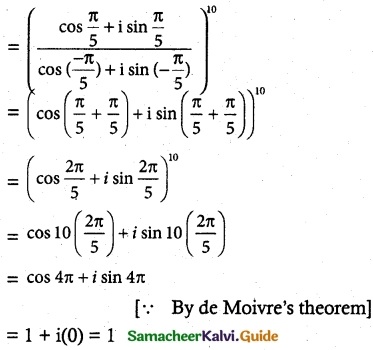
Aliter method:

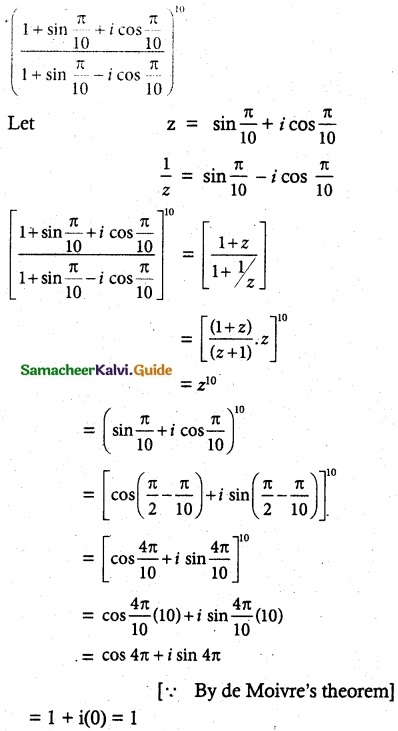
Question 4.
If 2cos α = x + \(\frac{1}{x}\) and 2 cos β = y + \(\frac{1}{x}\), show that
(i) \(\frac{x}{y}+\frac{y}{x}=2 \cos (\alpha-\beta)\)
Solution:
Given 2 cos α = x + \(\frac{1}{x}\) and cos β = y + \(\frac{1}{y}\)
simplifying x² – 2x cos α + 1 = 0

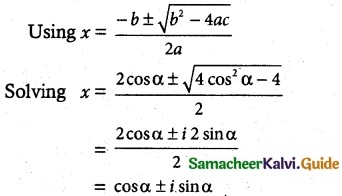
if x = cos α + i sin α, then \(\frac{1}{x}\) = cos α – i sin α
similarly y = cos β + i sin β and \(\frac{1}{y}\) = cos β – i sin β

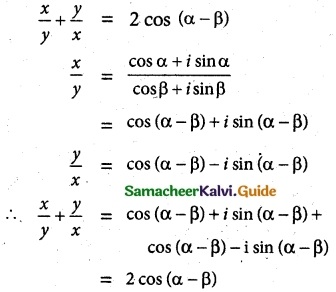
Hence proved

(ii) xy = (cos α + i sin α)(cos β + i sin β )
Solution:
xy = (cos α + i sin α) (cos β + i sin β)
= cos (α + β) + i sin (α + β)
[∵ arg (z₁z₂) = arg z₁ + arg z₂
\(\frac{1}{xy}\) = cos (α + β) – i sin (α + β)
∴ xy – \(\frac{1}{xy}\) = cos (α + β) + i sin (α + β) – cos (α + β) + i sin (α + β)
= 2i sin (α + β)
Hence proved

(iii) \(\frac{x^{m}}{y^{n}}-\frac{y^{n}}{x^{m}}\) = 2 i sin (mα – nβ)
Solution:

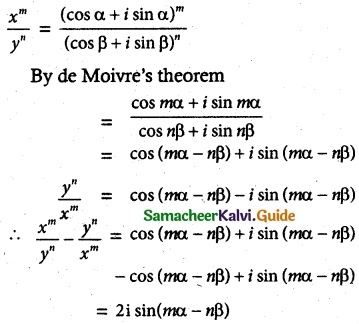
Hence Proved

(iv) x^m yⁿ + \(\frac { 1 }{ x^m y^n }\) = 2 cos(mα – nβ)
Solution:
= (cos mα + sin mα) (cos nβ + i sin nβ)
= cos (mα + nβ) + i sin (mα + nβ)

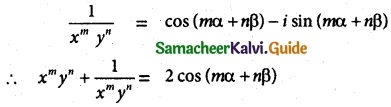
Hence proved

Question 5.
Solve the equation z³ + 27 = 0.
Solution:
z³ + 27 = 0
z³ = – 27 = 27 (-1)
= 27 [cos(π + 2kπ) + i sin(π + 2kπ)], k ∈ z
∴ z = (27)^1/3[cos (2k + 1)π + i sin (2k+1)π]^1/3 k ∈ z

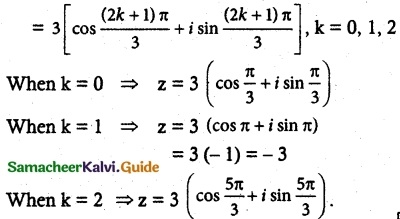
Question 6.
If ω ≠ 1 is a cube root of unity, show that the roots of the equation (z – 1)³ + 8 = 0 are -1, 1 – 2ω, 1 – 2ω².
Solution:
Given ω ≠ 1 is a active root of unity
(z – 1)³ + 8 = 0
(z- 1)³ = -8
z – 1 = (-8)^1/3 (1)^1/3
= (-2) (1, ω, ω²)
z – 1 = (-2, -2ω, -2ω²)
= z – 1 = -2
z = -2 + 1 = -1
z – 1 = -2ω
z = 1 – 2ω
z – 1 = -2ω²
z = 1 – 2ω²

Question 7.

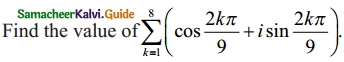
Solution:
\(\sum_{k=1}^{8}\) (cos \(\frac { 2kπ }{ 9 }\) + i sin \(\frac { 2kπ }{ 9 }\))
The sum all nth root of unity is
1 + ω + ω² + …….. + ω^n-1 = 0
From the given polar from , it is clear that the complex number is 1 + i0 (unity)

Question 8.
If ω ≠ 1 is a cube root of unity, show that
(i) (1 – ω + ω²)⁶ + (1 + ω – ω²)⁶ = 128.
Solution:
ω is a cube root of unity ω³ = 1; 1 + ω + ω² = 0
(1 – ω + ω²)⁶ + (1 + ω – ω²)⁶
= (-ω – ω)⁶ + (-ω² – ω²)⁶
= (-2ω)⁶ + (-2ω²)⁶
= (-2)⁶(ω⁶ + ω¹²)
= (64)(1 + 1)
= 128

(ii) (1 + ω) (1 + ω²) (1 + ω⁴) (1 + ω⁸)….. (1 + ω²ⁿ) = 1
Solution:
(1 + ω)(1 + ω²)(1 + ω⁴)(1 + ω⁸) …… (1 + ω²ⁿ)
= (1 + ω)(1 + ω²)(1 + ω⁴)(1 + ω⁸) ……. 2n factors
= (-ω²)(-ω)(-ω²)(-ω) …… 2n factors
= ω³. ω³
= 1
Hence proved.

Question 9.
If z = 2 – 2i, find the rotation of z by θ radians in the counterclockwise direction about the origin when
Solution:
Let 2 – 2i
Modules = |z| = \(\sqrt{2^2+2^2}\) = 2√2
Argument θ = tan^-1(\(\frac{-2}{2}\)) = tan^-1(-1) = –\(\frac{π}{4}\)
(i) when ‘z’ is rotated in the counter clockwise direction about the origin when θ = \(\frac{π}{3}\) i.,e argument of new position

(ii) θ = \(\frac{2π}{3}\)
Solution:

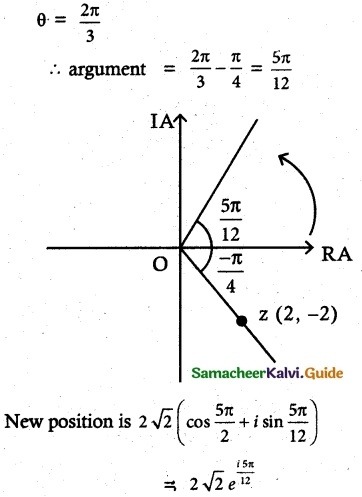
(iii) θ = \(\frac{3π}{3}\)
Solution:
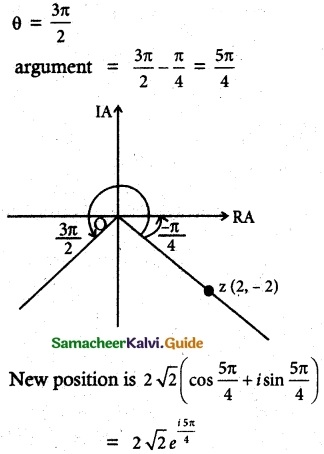