Category: Class 12

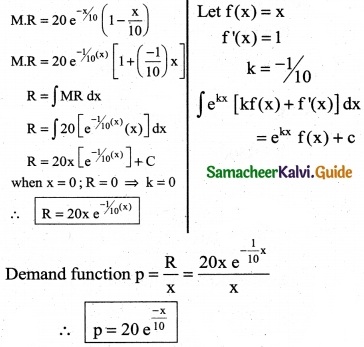
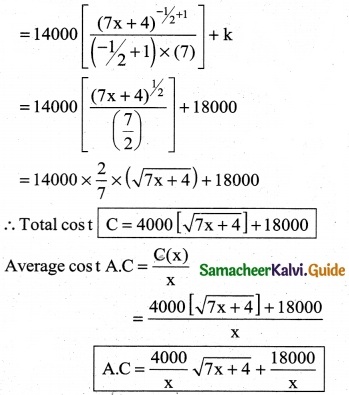
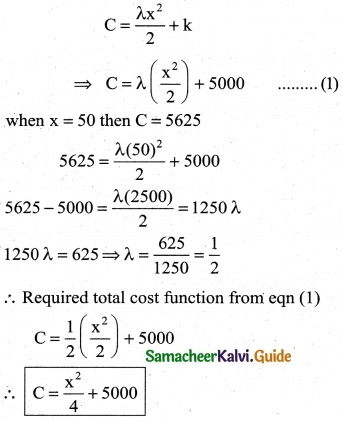
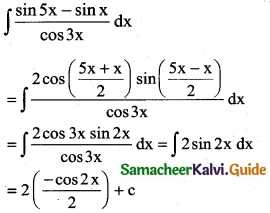Students can Download Tamil Nadu 12th Biology Model Question Paper 4 English Medium Pdf, Tamil Nadu 12th Biology Model Question Papers helps you to revise the complete Tamilnadu State Board New Syllabus, helps students complete homework assignments and to score high marks in board exams.

TN State Board 12th Biology Model Question Paper 4 English Medium

General Instructions:

1. 1. The question paper comprises of four parts. Questions for Botany and Zoology are asked separately.
   2. You are to attempt all the parts. An internal choice of questions is provided wherever applicable.
   3. All questions of Part I, II, III and IV are to be attempted separately.
   4. Question numbers 1 to 8 in Part I are Multiple Choice Questions of one mark each. These are to be answered by choosing the most suitable answer from the given four alternatives and writing the option code and the corresponding answer.
   5. Question numbers 9 to 14 in Part II are two-marks questions. These are to be answered in about one or two sentences.
   6. Question numbers 15 to 19 in Part III are three-marks questions. These are to be answered in about three to five short sentences.
   7. Question numbers 20 and 21 in Part IV are five-marks questions. These are to be answered in detail. Draw diagrams wherever necessary.

Time: 2.30 Hours
Maximum Marks: 70

Bio-Botany [Maximum Marks: 35]

Part – I

Choose the correct answer. [8 × 1 = 8]

Question 1.
A Plant called ‘X’ possesses small flower with reduced perianth and versatile anther. The probable agent for pollination would be _________.
(a) water
(b) air
(c) butterflies
(d) beetles
Answer:
(b) air

Question 2.
“Gametes are never hybrid”. This is a statement of _______.
(a) Law of dominance
(b) Law of independent assortment
(c) Law of segregation
(d) Law of random fertilization
Answer:
(c) Law of segregation

Question 3.
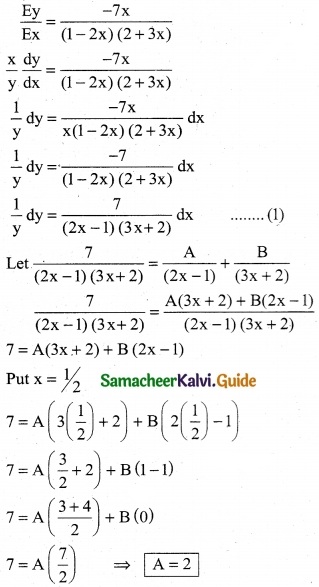
In which techniques Ethidium Bromide is used?
(a) Southern Blotting techniques
(b) Western Blotting techniques
(c) Polymerase Chain Reaction
(d) Agarose Gel Electrophoresis
Answer:
(d) Agarose Gel Electrophoresis

Question 4.
Which of the following statement is correct?
(a) Agar is not extracted from marine algae such as seaweeds
(b) Callus undergoes differentiation and produces somatic embryoids
(c) Surface sterilization of explants is done by using mercuric bromide
(d) pH of the culture medium is 5.0 to 6.0
Answer:
(d) pH of the culture medium is 5.0 to 6.0

Question 5.
The term pedogenesis is related to _______.
(a) Fossils
(b) Water
(c) Population
(d) Soil
Answer:
(d) Soil

Question 6.
Depletion of which gas in the atmosphere can lead to an increased incidence of skin cancer?
(a) Ammonia
(b) Methane
(c) Nitrous oxide
(d) Ozone
Answer:
(d) Ozone

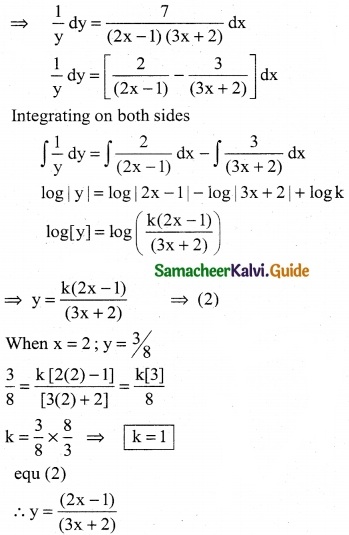
Question 7.
A wheat variety, Atlas 66 which has been used as a donor for improving cultivated wheat is rich in _______.
(a) iron
(b) carbohydrates
(c) proteins
(d) vitamins
Answer:
(c) proteins

Question 8.
Statement A: Coffee contains caffeine.
Statement B: Drinking coffee enhances cancer.
(a) A is correct, B is wrong
(b) A and B – Both are correct
(c) A is wrong, B is correct
(d) A and B – Both are wrong
Answer:
(b) A and B – Both are correct

Part – II

Answer any four of the following questions. [4 × 2 = 8]

Question 9.
Draw and label the structure of a typical pollen grain.
Answer:

Question 10.
What is test cross? Why it is done?
Answer:
Test cross is crossing an individual of unknown genotype with a homozygous recessive. Test cross is used to identify whether an individual is homozygous or heterozygous for dominant character.

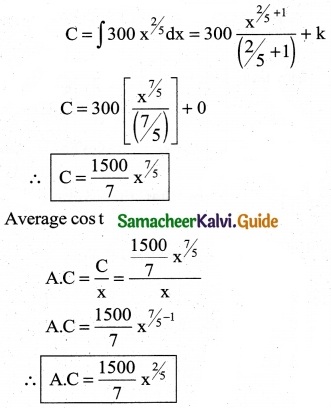
Question 11.
You are working in a biotechnology lab with a bacterium namely E.coli. How will you cut the nucleotide sequence? Explain it.
Answer:
The DNA nucleotide sequence can be cut using Restriction endonucleases (RE). Restriction

Question 12.
What is ecological hierarchy? Name the levels of ecological hierarchy.
Answer:
The interaction of organisms with their environment results in the establishment of grouping of organisms which is called ecological hierarchy.

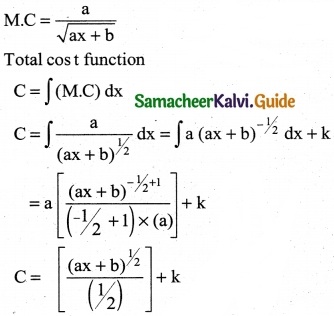
Question 13.
Mutagens are the substances that induces mutation. Name any two physical and chemical mutagens.
Answer:
UV short waves, X-rays – Physical mutagens.
Nitromethyl, Urea – Chemical mutagens.

Question 14.
What is pseudo cereal? Give an example.
Answer:
The term pseudo-cereal is used to describe foods that are prepared and eaten as a whole grain, but are botanical outliers from grasses. Example: quinoa. It is actually a seed from the Chenopodium quinoa plant, belongs to the family Amaranthaceae.

Part – III

Answer any three questions in which question number 19 is compulsory. [3 × 3 = 9]

Question 15.
“Endothecium is associated with dehiscence of anther” Justify the statement.
Answer:
The inner tangential wall develops bands (sometimes radial walls also) of α cellulose (sometimes also slightly lignified). The cells are hygroscopic. The cells along the junction of the two sporangia of an anther lobe lack these thickenings. This region is called stomium. This region along with the hygroscopic nature of endothecium helps in the dehiscence of anther at maturity.

Question 16.
What is gene mapping? Write its uses.
Answer:
The diagrammatic representation of position of genes and related distances between the adjacent genes is called genetic mapping. It is directly proportional to the frequency of recombination between them. It is also called as linkage map.

Uses of genetic mapping:

• It is used to determine gene order, identify the locus of a gene and calculate the distances between genes.
• They are useful in predicting results of dihybrid and trihybrid crosses.
• It allows the geneticists to understand the overall genetic complexity of particular organism.

Question 17.
How synthetic seeds are developed?
Answer:
Artificial seeds or synthetic seeds (synseeds) are produced by using embryoids (somatic embryos) obtained through in vitro culture. They may even be derived from single cells from any part of the plant that later divide to form cell mass containing dense cytoplasm, large nucleus, starch grains, proteins, and oils, etc. To prepare the artificial seeds different inert materials are used for coating the somatic embryoids like agrose and sodium alginate.

Question 18.
Discuss the three zones of a lentic ecosystem.
Answer:
There are three zones, littoral, limnetic and profundal. The littoral zone, which is closest to the shore with shallow water region, allows easy penetration of light. It is warm and occupied by rooted plant species. The limnetic zone refers the open water of the pond with an effective penetration of light and domination of planktons.

The deeper region of a pond below the limnetic zone is called profundal zone with no effective light penetration and predominance of heterotrophs. The bottom zone of a pond is termed benthic and is occupied by a community of organisms called benthos (usually decomposers).

Question 19.
Write a short note on clean development mechanism.
Answer:
Clean Development Mechanism (CDM) is defined in the Kyoto protocol (2007) which provides project based mechanisms with two objectives to prevent dangerous climate change and to reduce green house gas emissions. CDM projects helps the countries to reduce or limit emission and stimulate sustainable development.

An example for CDM project activity, is replacement of conventional electrification projects with solar panels or other energy efficient boilers. Such projects can earn Certified Emission Reduction (CER) with credits / scores, each equivalent to one tonne of CO₂, which can be counted towards meeting Kyoto targets.

Part – IV

Answer all the questions. [2 × 5 = 10]

Question 20.
(a) Describe dominant epistasis with an example.
Answer:

Dominant Epistasis – It is a gene interaction in which two alleles of a gene at one locus interfere and suppress or mask the phenotypic expression of a different pair of alleles of another gene at another locus. The gene that suppresses or masks the phenotypic expression of a gene at another locus is known as epistatic. The gene whose expression is interfered by non-allelic genes and prevents from exhibiting its character is known as hypostatic. When both the genes are present together, the phenotype is determined by the epistatic gene and not by the hypostatic gene.

In the summer squash the fruit colour locus has a dominant allele ‘W’ for white colour and a recessive allele ‘w’ for coloured fruit. ‘W’ allele is dominant that masks the expression of any colour. In another locus hypostatic allele ‘G’ is for yellow fruit and its recessive allele ‘g’ for green fruit. In the first locus the white is dominant to colour where as in the second locus yellow is dominant to green. When the white fruit with genotype WWgg is crossed with yellow fruit with genotype wwGG, the F₁ plants have white fruit and are heterozygous (WwGg). When F₁ heterozygous plants are crossed they give rise to F₂ with the phenotypic ratio of 12 white : 3 yellow : 1 green.

Since W is epistatic to the alleles ‘G’ and ‘g’ the white which is dominant, masks the effect of yellow or green. Homozygous recessive ww genotypes only can give the coloured fruits (4/16). Double recessive ‘wwgg’ will give green fruit (1/16). The Plants having only ‘G’ in its genotype (wwGg or wwGG) will give the yellow fruit(3/16).

[OR]

(b) Point out the significance of plant succession.
Answer:
Significance of Plant Succession:

• Succession is a dynamic process. Hence an ecologist can access and study the seral stages of a plant community found in a particular area.
• The knowledge of ecological succession helps to understand the controlled growth of one or more species in a forest.
• Utilizing the knowledge of succession, even dams can be protected by preventing siltation.
• It gives information about the techniques to be used during reforestation and afforestation.
• It helps in the maintenance of pastures.
• Plant succession helps to maintain species diversity in an ecosystem.
• Patterns of diversity during succession are influenced by resource availability and disturbance by various factors.
• Primary succession involves the colonization of habitat of an area devoid of life.
• Secondary succession involves the re-establishment of a plant community in disturbed area or habitat.
• Forests and vegetation that we come across all over the world are the result of plant succession.

Question 21.
(a) Compare the various types of Blotting techniques.
Answer:

[OR]

(b) Explain different types of hybridization.
Answer:
Types of Hybridization:
According to the relationship between plants, the hybridization is divided into.
1. Intravarietal hybridization – The cross between the plants of same variety. Such crosses are Useful only in the self-pollinated crops.

2.. Intervarietal hybridization – The cross between the plants belonging to two different varieties of the same species and is also known as intraspecific hybridization. This technique has been the basis of improving self-pollinated as well as cross pollinated crops.

3. Interspecific hybridization – The cross between the plants belonging to different species belonging to the same genus is also called intragenic hybridization. It is commonly used for transferring the genes of disease, insect, pest and drought resistance from one species to another.
Example: Gossypium hirsutum x Gossypium arboreum – Deviraj.

4. Intergeneric hybridization – The crosses are made between the plants belonging to two different genera. The disadvantages are hybrid sterility, time consuming and expensive procedure. Example: Raphanobrassica and Triticale.

Bio-Zoology [Maximum Marks: 35]

Part – I

Choose the correct answer. [8 × 1 = 8]

Question 1.
Select the correct production site and action site of Relaxin.
(a) Hypothalamus and pituitary gland
(b) Pituitary gland and Pelvic joints and cervix
(c) Placenta and pelvic joint and cervix
(d) Hypothalamus and placenta
Answer:
(c) Placenta and pelvic joint and cervix

Question 2.
Fusion of young individuals produced immediately after the mitotic division of adult parent cell is called _______.
(a) Merogamy
(b) Anisogamy
(c) Hologamy
(d) Paedogamy
Answer:
(d) Paedogamy

Question 3.
Mangolism is a genetic disorder which is caused by the presence of an extra chromosome number ______.
(a) 20
(b) 21
(c) 23
(d) 19
Answer:
(b) 21

Question 4.
DNA finger printing techniques was developed by _______.
(a) Jacob and Monod
(b) Alec Jeffreys
(c) Frederick Sanger
(d) Hershey and Chase
Answer:
(b) Alec Jeffreys

Question 5.
Identify the correct sequence of periods from oldest to youngest
(a) Cambrian → Permian → Devonian → Silurian → Ordovician
(b) Permian → Silurian → Devonian → Ordovician → Cambrian
(c) Permian → Devonian → Silurian → Cambrian → Ordovician
(d) Cambrian → Ordovician → Silurian → Devonian → Permian
Answer:
(d) Cambrian → Ordovician → Silurian → Devonian → Permian

Question 6.
Spread of cancerous cells to distant sites is termed as _________.
(a) Metastasis
(b) Oncogenes
(c) Proto-oncogenes
(d) Malignant neoplasm
Answer:
(a) Metastasis

Question 7.
Assertion (A): Streptomycin is an antibiotic.
Reason (R): Antibiotic are microbial chemicals inhibits the growth of pathogenic microbe.
(a) A is right R is wrong
(b) R explains A
(c) A and R are wrong
(d) A is wrong but R is right
Answer:
(b) R explains A

Question 8.
World Ozone Day was observed on _________.
(a) September 16^th
(b) October 12^th
(c) December 1^th
(d) August 18^th
Answer:
(a) September 16^th

Part – II

Answer any four of the following questions. [4 × 2 = 8]

Question 9.
Name the active chemical found in the medicinal plant Rauwolfia vomitoria. What type of diversity does it belongs to?
Answer:
Rauwolfia vomitoria can be cited as an example for genetic diversity. Reserpine is an active chemical extracted from Rauwolfia vomitoria.

Question 10.
State any two unique features of ELISA test.
Answer:
ELISA is highly sensitive and can detect antigen even in nanograms.
ELISA test does not require radioisotopes or radiation counting apparatus.

Question 11.
Define Anaphylaxis.
Answer:
Anaphylaxis is the classical immediate hypersensitivity reaction. It is a sudden, systematic, severe and immediate hypersensitivity reaction occurring as a result of rapid generalized mast-cell degranulation

Question 12.
Who is Cro-Magnon?
Answer:
Cro-Magnon was one of the most talked forms of modem human found from the rocks of Cro-Magnon, France and is considered as the ancestor of modem Europeans. They were not only adapted to various environmental conditions, but were also known for their cave paintings, figures on floors and walls.

Question 13.
What is S – D sequence?
Answer:
The 5′ end of the mRNA of prokaryotes has a special sequence which precedes the initial AUG start codon of mRNA. This ribosome binding site is called the Shine – Dalgamo sequence or S-D sequence. This sequences base-pairs with a region of the 16Sr RNA of the small ribosomal subunit facilitating initiation.

Question 14.
Expand (a) GIFT (b) ICSI
Answer:
GIFT – Gamete Intra – Fallopian Transfer
ICSI – Intra-cytoplasmic sperm injection

Part – III

Answer any three questions in which question number 19 is compulsory. [3 × 3 = 9]

Question 15.
Write a short note on encystment in amoeba.
Answer:
During unfavorable conditions (increase or decrease in temperature and scarcity of food) Amoeba withdraws its pseudopodia and secretes a three-layered, protective, chitinous cyst wall around it and becomes inactive. This phenomenon is called encystment. When conditions become favourable, the encysted Amoeba divides by multiple fission and produces many minute amoebae called pseudopodiospore or amoebulae.

The cyst wall absorbs water and breaks off liberating the young pseudopodiospores, each with a fine pseudopodia. They feed and grow rapidly to lead an independent life.

Question 16.
Draw a schematic representation of human oogenesis.
Answer:

Question 17.
Comment on the methods of Eugenics.
Answer:
Eugenics refers to the study of the possibility of improving the qualities of human population. Methods of Eugenics:

• Sex-education in school and public forums.
• Promoting the uses of contraception.
• Compulsory sterilization for mentally retarded and criminals.
• Egg donation.
• Artificial insemination by donors.
• Prenatal diagnosis of genetic disorders and performing MTP.
• Gene therapy.
• Cloning.
• Egg/sperm donation of healthy individuals.

Question 18.
Both strands of DNA are not copied during transcription. Give reason.
Answer:
Both the strands of DNA are not copied during transcription for two reasons.
1. If both the strands act as a template, they would code for RNA with different sequences. This in turn would code for proteins with different amino acid sequences. This would result in one segment of DNA coding for two different proteins, hence complicate the genetic information transfer machinery.

2. If two RNA molecules were produced simultaneously, double stranded RNA complementary to each other would be formed. This would prevent RNA from being translated into proteins.

Question 19.
What is Q₁₀ value? How it is calculated?
Answer:
The effect of temperature on the rate of reaction is expressed in terms of temperature coefficient or Q₁₀ value. The Q₁₀ values are estimated taking the ratio between the rate of reaction at X°C and rate of reaction at (X-10°C).

Part – IV

Answer all the questions. [2 × 5 = 10]

Question 20.
(a) Differentiate between r-selected species and k-selected species.
Answer:
r – selected species:

• Smaller sized organisms
• Produce many offspring
• Mature early
• Short life expectancy
• Each individual reproduces only once or few times in their life time
• Only few reach adulthood
• Unstable environment, density independent

k – seleced species:

• Larger sized organisms
• Produce few offspring
• Late maturity with extended parental care
• Long life expectancy
• Can reproduce more than once in lifetime
• Most individuals reach maximum life span
• Stable environment, density dependent

[OR]

(b) Give a detailed account on ethanol production by microbes and the uses of ethanol.
Answer:
Ethanol production:
Saccharomyces cerevisiae (Yeast) is the major product of ethanol.

Since ethanol is used for industrial, laboratory and fuel proposes, it is called as industrial alcohol.

Organism used: Saccharomyces cerevisiae, bacteria like Zymomonas mobilis and Sarcina ventriculi.
Substances used: Molasses, Com, Potatoes, wood waste.

Process of ethanol production:
Step-1: Milling of fees stock.
Step-2: Adding fungal (Aspergillus) amylase to break down starch into sugar.
Step-3: Yeast is added to convert sugar into ethanol.
Step-4: Distillation yield 96% concentrated ethanol.

Uses of Ethanol:
Ethanol and bio-diesel are the two commonly used first generation bio-fuels.
Ethanol is used as fuel, mainly as bio-fuel additive for gasoline.

Question 21.
(a) How DNA is packed in an eukaryotic cell?
Answer:
In eukaryotes, organization is more complex. Chromatin is formed by a series of repeating units called nucleosomes. Komberg proposed a model for the nucleosome, in which 2 molecules of the four histone proteins H2A, H2B, H3 and H4 are organized to form a unit of eight molecules called histone octamere. The negatively charged DNA is wrapped around the positively charged histone octamere to form a structure called nucleosome. A typical nucleosome contains 200 bp of DNA helix.

The histone octameres are in close contact and DNA is coiled on the outside of nucleosome. Neighbouring nucleosomes are connected by linker DNA (H1) that is exposed to enzymes. The DNA makes two complete turns around the histone octameres and the two turns are sealed off by an H1 molecule. Chromatin lacking H1 has a beads-on-a-string appearance in which DNA enters and leaves the nucleosomes at random places. H1 of one nucleosome can interact with H1 of the neighbouring nucleosomes resulting in the further folding of the fibre.

The chromatin fiber in interphase nuclei and mitotic chromosomes have a diameter that vary between 200-300 nm and represents inactive chromatin. 30 nm fibre arises from the folding of nucleosome, chains into a solenoid structure having six nucleosomes per turn. This structure is stabilized by interaction between different H1 molecules. DNA is a solenoid and packed about 40 folds. The hierarchical nature of chromosome structure is illustrated.

Additional set of proteins are required for packing of chromatin at higher level and are referred to as non-histone chromosomal proteins (NHC). In a typical nucleus, some regions of chromatin are loosely packed (lightly stained) and are referred to as euchromatin. The chromatin that is tightly packed (stained darkly) is called heterochromatin. Euchromatin is transcriptionally active and heterochromatin is transcriptionally inactive.

[OR]

(b) Explain Oparin – Haldane hypothesis on evolution.
Answer:
According to the theory of chemical evolution primitive organisms in the primordial environment of the Earth evolved spontaneously from inorganic substances and physical forces such as lightning, UV radiations, volcanic activities, etc. Oparin (1924) suggested that the organic compounds could have undergone a series of reactions leading to more complex molecules. He proposed that the molecules formed colloidal aggregates or ‘coacervates’ in an aqueous environment.

The coacervates were able to absorb and assimilate organic compounds from the environment. Haldane (1929) proposed that the primordial sea served as a vast chemical laboratory powered by solar energy. The atmosphere was oxygen free and the combination of CO₂, NH₃ and UV radiations gave rise to organic compounds. The sea became a ‘hot’ dilute soup containing large populations of organic monomers and polymers.

They envisaged that groups of monomers and polymers acquired lipid membranes and further developed into the first living cell. Haldane coined the term prebiotic soup and this became the powerful symbol of the Oparin-Haldane view on the origin of life (1924-1929). Oparin and Haldane independently suggested that if the primitive ‘ atmosphere was reducing and if there was appropriate supply of energy such as lightning or UV light then a wide range of organic compounds can be synthesized.

Read More:

TRENT Pivot Point Calculator

Tamilnadu State Board New Syllabus Samacheer Kalvi 12th Business Maths Guide Pdf Chapter 2 Integral Calculus I Ex 2.8 Text Book Back Questions and Answers, Notes.

Tamilnadu Samacheer Kalvi 12th Business Maths Solutions Chapter 2 Integral Calculus I Ex 2.8

Using second fundamental theorem, evaluate the following:

Question 1.
\(\int_{0}^{1}\) e^2x dx
Solution:

Question 2.
\(\int_{0}^{1/4}\) \(\sqrt { 1 -4x}\) dx
Solution:

Question 3.
\(\int_{0}^{1}\) \(\frac { xdx }{x^2+1}\)
Solution:

Question 4.
\(\int_{0}^{3}\) \(\frac { e^xdx }{1+e^x}\)
Solution:
\(\int_{0}^{3}\) \(\frac { e^xdx }{1+e^x}\)
= {log |1 + ex|}\(_{0}^{3}\)
= log |1 + e³| – log |1 + e°|
= log |1 + e³| – log |1 + 1|
= log |1 + e³| – log |2|
= log |\(\frac { 1+e^3 }{2}\)|

Question 5.
\(\int_{0}^{1}\) xe^x² dx
Solution:

Question 6.
\(\int_{1}^{e}\) \(\frac { dx }{x(1+logx)^3}\)
Solution:

Question 7.
\(\int_{-1}^{1}\) \(\frac { 2x+3 }{x^2+3x+7}\) dx
Solution:

Question 8.
\(\int_{0}^{π/2}\) \(\sqrt { 1 +cosx} \) dx
Solution:

Question 9.
\(\int_{1}^{2}\) \(\frac { x-1 }{x^2}\) dx
Solution:

Evaluate the following

Question 10.
\(\int_{1}^{4}\) f(x) dx where f(x) = \(\left\{\begin{array}{l}
4 x+3,1 \leq x \leq 2 \\
3 x+5,2 \end{array}\right.\)
Solution:
\(\int_{1}^{4}\) f(x) dx
= \(\int_{1}^{2}\) f(x) dx + \(\int_{2}^{4}\) f(x) dx
= \(\int_{1}^{2}\) (4x + 3) dx + \(\int_{2}^{4}\) (3x + 5) dx

(8 + 6) – [5] + [24 + 20] – [6 + 10]
= 14 – 5 + 44 – 16
= 58 – 21
= 37

Question 11.
\(\int_{0}^{2}\) f(x) dx where f(x) = \(\left\{\begin{array}{ll}
3-2 x-x^{2}, & x \leq 1 \\
x^{2}+2 x-3, & 1<x \leq 2
\end{array}\right.\)
Solution:
\(\int_{0}^{2}\) f(x) dx
= \(\int_{0}^{1}\) f(x) dx + \(\int_{1}^{2}\) f(x) dx
= \(\int_{0}^{1}\) (3 – 2x – x²) dx + \(\int_{1}^{2}\) (x² + 2x – 3) dx

Question 12.
\(\int_{-1}^{1}\) f(x) dx where f(x) = \(\left\{\begin{array}{ll}
x, & x \geq 0 \\
-x, & x<0
\end{array}\right.\)
Solution:
\(\int_{-1}^{1}\) f(x) dx
\(\int_{-1}^{0}\) f(x) dx + \(\int_{0}^{1}\) f(x) dx
= \(\int_{-1}^{0}\) (-x) dx + \(\int_{0}^{1}\) x dx

Question 13.
f(x) = \(\left\{\begin{array}{l}
c x, \quad 0<x<1 \\
0, \text { otherwise }
\end{array}\right.\) find ‘c’ if \(\int_{0}^{1}\) f(x) dx = 2
Solution:
Given
f(x) = \(\left\{\begin{array}{l}
c x, \quad 0<x<1 \\
0, \text { otherwise }
\end{array}\right.\)
⇒ \(\int_{0}^{1}\) f(x) dx = 2
⇒ \(\int_{0}^{1}\) cx dx = 2
c[ \(\frac { x^2 }{ 2 }\) ]\(_{0}^{1}\) = 2
c[ \(\frac { 1 }{ 2 }\) – 0 ] = 2
\(\frac { 1 }{ 2 }\) = 2
⇒ c = 4

Must Read:

MARICO Pivot Point Calculator

Tamilnadu State Board New Syllabus Samacheer Kalvi 12th Business Maths Guide Pdf Chapter 2 Integral Calculus I Ex 2.9 Text Book Back Questions and Answers, Notes.

Tamilnadu Samacheer Kalvi 12th Business Maths Solutions Chapter 2 Integral Calculus I Ex 2.9

Evaluate the following using properties of definite integrals:

Question 1.
\(\int_{-π/4}^{π/4}\) x³ cos³ x dx
Solution:
Let f(x) = x³cos³x
f(-x) = (-x)³ cos³(-x)
= -x³ cos³x
f(-x) = -f(x)
⇒ f(x) is an odd function
∴ \(\int_{-π/4}^{π/4}\) x³ cos³ x dx = 0

Question 2.
\(\int_{-π/2}^{π/2}\) sin² θ dθ
Solution:
Let f(θ)= sin² θ
f(-θ) = sin² (-θ) = [sin (-θ)]²
= [-sin θ]² = sin² θ
f(-θ) = f(θ)
∴ f(θ) is an even function

Question 3.
\(\int_{-1}^{1}\) log(\(\frac { 2-x }{2+x}\)) dx
Solution:

Question 4.
\(\int_{0}^{π/2}\) \(\frac { sin^7x }{sin^7x+cos^7x}\) dx
Solution:
Using the property
\(\int_{0}^{a}\) f(x) dx = \(\int_{0}^{a}\) f(a – x) dx

Question 5.
\(\int_{0}^{1}\) log (\(\frac { 1 }{x}\) – 1) dx
Solution:

Question 6.
\(\int_{0}^{1}\) \(\frac { x }{(1-x)^{3/4}}\) dx
Solution:
Let I = \(\int_{0}^{1}\) log \(\frac { x }{(1-x)^{3/4}}\) dx
Using the property
\(\int_{0}^{a}\) f(x) dx = \(\int_{0}^{a}\) f(a – x) dx

Read More:

CUB Pivot Point Calculator

Tamilnadu State Board New Syllabus Samacheer Kalvi 12th Business Maths Guide Pdf Chapter 2 Integral Calculus I Ex 2.10 Text Book Back Questions and Answers, Notes.

Tamilnadu Samacheer Kalvi 12th Business Maths Solutions Chapter 2 Integral Calculus I Ex 2.10

Evaluate the following:

Question 1.
(i) \(\Upsilon\) (4)
Solution:
Γ(4) = Γ(3 + 1) = 3! = 6

(ii) \(\Upsilon\) (\(\frac { 9 }{2}\))
Solution:

(iii) \(\int_{0}^{∞}\) e^-mx x⁶ dx
Solution:
W.K.T \(\int_{0}^{∞}\) xⁿ e^-ax dx = \(\frac { n! }{a^{n+1}}\)
∴ \(\int_{0}^{∞}\) e^-mx x⁶ dx = \(\frac { 6! }{3^{6+1}}\) = \(\frac { 6! }{m^7}\)

(iv) \(\int_{0}^{∞}\) e^-4x x⁴ dx
Solution:

(v) \(\int_{0}^{∞}\) e^-x/2 x⁵ dx
Solution:

Question 2.
If f(x) = \(\left\{\begin{array}{l}
x^{2} e^{-2 x}, x \geq 0 \\
0, \text { otherwise }
\end{array}\right.\), then evaluate \(\int_{0}^{∞}\) f(x) dx
Solution:
Given

Read More:

• ABCAPITAL Pivot Point Calculator

Tamilnadu State Board New Syllabus Samacheer Kalvi 12th Business Maths Guide Pdf Chapter 3 Integral Calculus II Ex 3.4 Text Book Back Questions and Answers, Notes.

Tamilnadu Samacheer Kalvi 12th Business Maths Solutions Chapter 3 Integral Calculus II Ex 3.4

Choose the most suitable answer from the given four alternatives:

Question 1.
Area bounded by the curve y = x (4 – x) between the limits 0 and 4 with x-axis is
(a) \(\frac { 30 }{3}\) sq.unit
(b) \(\frac { 31 }{2}\) sq.unit
(c) \(\frac { 32 }{3}\) sq.unit
(d) \(\frac { 15 }{3}\) sq.unit
Solution:
(c) \(\frac { 32 }{3}\) sq.unit
Hint:

Question 2.
Area bounded by the curve y = e^-2x between the limits 0 < x < ∞ is
(a) 1 sq.units
(b) \(\frac { 1 }{2}\) sq.units
(c) 5 sq.units
(d) 2 sq.units
Solution:
(b) \(\frac { 1 }{2}\) sq.units
Hint:

Question 3.
Area bounded by the curve y = \(\frac { 1 }{x}\) between the limits 1 and 2 is
(a) log 2 sq.units
(b) log 5 sq.units
(c) log 3 sq.units
(d) log 4 sq.units
Solution:
(a) log 2 sq.units
Hint:
Area = \(\int_{1}^{2} \frac{1}{x} d x\)
= \((\log x)_{1}^{2}\)
= log 2 – log 1
= log 2 (Since log 1 = 0)

Question 4.
If the marginal revenue function of a firm is MR = e^{\(\frac { -x }{10}\)} then revenue is
(a) 1 – e^-x/10
(b) e^-x/10 + 10
(c) 10(1 – e^-x/10)
(d) -10e^-x/10
Solution:
(c) 10(1 – e^-x/10)
Hint:
MR = e^{\(\frac { -x }{10}\)} then R = ∫MR dx
R = ∫e^-x/10 dx = \(\frac { e^{-x/10} }{(-1/10)}\) + k
R = -10e^-x/10 + k when x = 0, R = 0
⇒ 0 = -10e⁰ + k
0 = -10(1) + k
∴ k = 10
R = -10e^-x/10 + 10 = 10(1 – e^-x/10)

Question 5.
If MR and MC denotes the marginal revenue and marginal cost functions, then the profit functions is
(a) P = ∫(MR – MC) dx + k
(b) P = ∫(R – C) dx + k
(c) P = ∫(MR + MC)dx + k
(d) P = ∫(MR) (MC) dx + k
Solution:
(a) P = ∫(MR – MC) dx + k
Hint:
Profit = Revenue – Cost

Question 6.
The demand and supply functions are given by D(x) = 16 – x² and S(x) = 2x² + 4 are under perfect competition, then the equilibrium price x is
(a) 2
(b) 3
(c) 4
(d) 5
Solution:
(a) 2
Hint:
D(x) =16 – x² and S(x) = 2x² + 4
Under perfect competition D(x) = S(x)
16 – x² = 2x² + 4; 16 – 4 = 2x² + x²
3x² = 12 ⇒ x² = \(\frac { 12 }{3}\) = 4
∴ x = ± 2, x cannot be in negative
∴ x = 2

Question 7.
The marginal revenue and marginal coast functions of a company are MR = 30 – 6x and MC = -24 + 3x where x is the product, profit function is
(a) 9x² + 54x
(b) 9x² – 54x
(c) 54x – \(\frac { 9x^2 }{2}\)
(d) 54x – \(\frac { 9x^2 }{2}\) + k
Solution:
(d) 54x – \(\frac { 9x^2 }{2}\) + k
Hint:
Profit = ∫(MR – MC) dx + k
= ∫(30 – 60) – (-24 + 3x) dx + k
= ∫(54 – 9x) dx + k
= 54x – \(\frac{9 x^{2}}{2}\) + k

Question 8.
The given demand and supply function are given by D(x) = 20 – 5x and S(x) = 4x + 8 if they are under perfect competition then the equilibrium demand is
(a) 40
(b) \(\frac { 41 }{2}\)
(c) \(\frac { 40 }{3}\)
(d) \(\frac { 41 }{5}\)
Solution:
(c) \(\frac { 40 }{3}\)
Hint:
Under perfect competition D(x) = S(x)
20 – 5x = 4x + 8
20 – 8 = 4x + 5x ⇒ 9x = 12
x = \(\frac { 4 }{3}\)
when x = \(\frac { 4 }{3}\); D(x) = 20 – 5(\(\frac { 4 }{3}\)) = 20 – \(\frac { 20 }{3}\)
= \(\frac { 40 }{3}\)

Question 9.
If the marginal revenue MR = 35 +7x – 3x², then the average revenue AR is.
(a) 35x + \(\frac { 7x^2 }{2}\) – x³
(b) 35x + \(\frac { 7x }{2}\) – x²
(c) 35x + \(\frac { 7x }{2}\) + x²
(d) 35x + 7x + x²
Solution:
(c) \(\frac { 40 }{3}\)
Hint:
R = ∫MR dx = ∫(35 + 7x – 3x²) dx

Question 10.
The profit of a function p(x) is maximum when
(a) MC – MR = 0
(b) MC = 0
(c) MR = 0
(d) MC + MR = 0
Solution:
(a) MC – MR = 0
Hint:
P = Revenue – Cost
P is maximum when \(\frac{d p}{d x}\) = 0
\(\frac{d p}{d x}\) = R'(x) – C'(x) = MR – MC = 0

Question 11.
For the demand function p(x), the elasticity of demand with respect to price is unity then.
(a) revenue is constant
(b) a cost function is constant
(c) profit is constant
(d) none of these
Solution:
(a) Revenue is constant

Question 12.
The demand function for the marginal function MR = 100 – 9x² is
(a) 100 – 3x²
(b) 100x – 3x²
(c) 100x – 9x²
(d) 100 + 9x²
Solution:
(a) 100 – 3x²
Hint:
R = ∫(MR) dx + c₁
R = ∫(100 – 9x²) dx + c₁
R = 100x – 3x³ + c₁
When R = 0, x = 0, c₁ = 0
R = 100x – 3x³
Demand function is \(\frac{R}{x}\) = 100 – 3x²

Question 13.
When x₀ = 5 and p₀ = 3 the consumer’s surplus for the demand function p_d = 28 – x²
(a) 250 units
(b) \(\frac { 250 }{3}\) units
(c) \(\frac { 251 }{2}\) units
(d) \(\frac { 251 }{3}\) units
Solution:
(b) \(\frac { 250 }{3}\) units
Hint:

Question 14.
When x₀ = 2 and P₀ = 12 the producer’s surplus for the supply function P₀ = 2x² + 4 is
(a) \(\frac { 31 }{5}\) units
(b) \(\frac { 31 }{2}\) units
(c) \(\frac { 32 }{2}\) units
(d) \(\frac { 30 }{7}\) units
Solution:
(c) \(\frac { 32 }{2}\) units
Hint:
Producer’s Surplus

Question 15.
Area bounded by y = x between the lines y = 1, y = 2 with y = axis is
(a) \(\frac { 1 }{2}\) sq units
(b) \(\frac { 5 }{2}\) sq units
(c) \(\frac { 3 }{2}\) sq units
(d) 1 sq units
Solution:
(c) \(\frac { 3 }{2}\) sq units
Hint:

Question 16.
The producer’s surplus when supply the function for a commodity is p = 3 + x and x₀ = 3 is
(a) \(\frac { 1 }{2}\)
(b) \(\frac { 9 }{2}\)
(c) \(\frac { 3 }{2}\)
(d) \(\frac { 7 }{2}\)
Solution:
(b) \(\frac { 9 }{2}\)
Hint:
p = 3 + x and x₀ = 3
then p₀ = 3 + 3 = 6

Question 17.
The marginal cost function is MC = 100√x find AC given that TC = 0 when the out put is zero is
(a) \(\frac { 200 }{3}\) x^1/2
(b) \(\frac { 200 }{3}\) x^3/2
(c) \(\frac { 200 }{3x^{3/2}}\)
(d) \(\frac { 200 }{3x^{1/2}}\)
Solution:
(a) \(\frac { 200 }{3}\) x^1/2
Hint:
TC = ∫MC dx = ∫100√x dx = 100 ∫(x)^1/2 dx

Question 18.
The demand and supply function of a commodity are P(x) = (x – 5)² and S(x) = x² + x + 3 then the equilibrium quantity x₀ is
(a) 5
(b) 2
(c) 3
(d) 10
Solution:
(b) 2
Hint:
At equilibrium, P(x) = S(x)
⇒ (x – 5)² = x² + x + 3
⇒ x² – 10x + 25 = x² + x + 3
⇒ 11x = 22
⇒ x = 2

Question 19.
The demand and supply function of a commodity are D(x) = 25 – 2x and S(x) = \(\frac { 10+x }{2}\) then the equilibrium price P₀ is
(a) 2
(b) 2
(c) 3
(d) 10
Solution:
(a) 2
Hint:
At equilibrium, D(x) = S(x)
25 – 2x = \(\frac{10+x}{4}\)
⇒ 100 – 8x = 10 + x
⇒ x = 10
That is x₀ = 10
P₀ = 25 – 2(x₀) = 25 – 20 = 5

Question 20.
If MR and MC denote the marginal revenue and marginal cost and MR – MC = 36x – 3x² – 81, then maximum profit at x equal to
(a) 3
(b) 6
(c) 9
(d) 10
Solution
(c) 9
Hint:
Profit P = ∫(MR – MC) dx = ∫(36x – 3x² – 81) dx
P = [\(\frac { 36x^2 }{2}\) – \(\frac { 3x^3 }{3}\) – 81x] = 18x² – x³ – 81x
when p = 0; 18x² – x³ – 81x = 0 ⇒ x² – 18x + 81 = 0
(x – 9)² = 0 ⇒ x – 9 = 0
∴ x = 9

Question 21.
If the marginal revenue of a firm is constant, then the demand function is
(a) MR
(b) MC
(c) C(x)
(d) AC
Solution:
(a) MR
Hint:
MR = k (constant)
Revenue function R = ∫(MR) dx + c₁
= ∫kdx + c₁
= kx + c₁
When R = 0, x = 0, ⇒ c₁ = 0
R = kx
Demand function p = \(\frac{R}{x}=\frac{k x}{x}\) = k constant
⇒ p = MR

Question 22.
For a demand function p, if ∫\(\frac { dp }{p}\) = k ∫\(\frac { dx }{x}\) then k is equal to
(a) n_d
(b) -n_d
(c) \(\frac { -1 }{n_d}\)
(d) \(\frac { 1 }{n_d}\)
Solution:
(c) \(\frac { -1 }{n_d}\)

Question 23.
The area bounded by y = e^x between the limits 0 to 1 is
(a) (e – 1) sq.units
(b) (e + 1) sq.units
(c) (1 – \(\frac { 1 }{e}\)) sq.units
(d) (1 + \(\frac { 1 }{e}\)) sq.units
Solution:
(a) (e – 1) sq.units
Hint:
Area A = \(\int_{a}^{b}\)ydx = \(\int_{0}^{1}\)e^xdx = [e^x]\(_{0}^{1}\)
= [e^x – e°] = [e – 1]

Question 24.
The area bounded by the parabola y² = 4x bounded by its latus rectum is
(a) \(\frac { 16 }{3}\) sq units
(b) \(\frac { 8 }{3}\) sq units
(c) \(\frac { 72 }{3}\) sq units
(d) \(\frac { 1 }{3}\) sq units
Solution:
(b) \(\frac { 8 }{3}\) sq units
Hint:

y² = 4x ⇒ y = \(\sqrt { 4x}\) 2√x = 2(x)^1/2
In this parabola 4a = 4 ⇒ a = 1 and vertex V(0, 0)

Question 25.
The area bounded by y = |x| between the limits 0 and 2 is
(a) 1 sq.units
(b) 3 sq.units
(c) 2 sq.units
(d) 4 sq.units
Solution:
(c) 2 sq.units
Hint:
Area A = \(\int_{a}^{b}\)ydx = \(\int_{0}^{2}\)x dx = [ \(\frac { x^2 }{2}\) ]\(_{0}^{2}\)
= \(\frac { (2)^2 }{2}\) – (0) = \(\frac { 4 }{2}\) = 2

Must Read:

Icici Bank Pivot Point

Tamilnadu State Board New Syllabus Samacheer Kalvi 12th Business Maths Guide Pdf Chapter 3 Integral Calculus II Ex 3.3 Text Book Back Questions and Answers, Notes.

Tamilnadu Samacheer Kalvi 12th Business Maths Solutions Chapter 3 Integral Calculus II Ex 3.3

Question 1.
Calculate consumer’s surplus if the demand function p = 50 – 2x and x = 20
Solution:
Demand function p = 50 – 2x and x = 20
when x = 20, p = 50 – 2(20)
p = 50 – 40 = 10
∴ p₀ = 10
CS = \(\int _{0}^{x}\) (demand function) dx – (Price × quantity demanded)
= \(\int _{0}^{20}\) (50 – 2x)dx – (10 × 20)
= [50x – 2(\(\frac { x^2 }{2}\))]\( _{0}^{20}\) – 200
= [50x – x²]\( _{0}^{20}\) – 200
= {50(20) – (20)² – [0]} – 200
= (1000 – 400) – 200
= 600 – 200
∴ C.S = 400 units

Question 2.
Calculate consumer’s surplus if the demand function p = 122 – 5x – 2x² and x = 6.
Solution:
Demand function p = 122 – 5x – 2x² and x = 6
when x = 6; p = 122 – 5(6) – 2(6)²
= 122 – 30 – 2 (36)
= 122 – 102 = 20
∴ p₀ = 20
C.S = \(\int _{0}^{x}\) (demand function) dx – (Price × quantity demanded)
= \(\int _{0}^{6}\)(122 – 5x – 2x²) dx – (20 × 6)

[732 – 5(18) – 2(72)] – 120
= 732 – 90 – 144 – 120
= 732 – 354 = 378
∴ CS = 378 units

Question 3.
The demand function p = 85 – 5x and supply function p = 3x – 3. Calculate the equilibrium price and quantity demanded. Also calculate consumer’s surplus.
Solution:
Demand function p = 85 – 5x
Supply function p = 3x – 35
W.K.T. at equilibrium prices p_d = p_s
85 – 5x = 3x – 35
85 + 35 = 3x + 5x
120 = 8x ⇒ x = \(\frac { 120 }{8}\)
∴ x = 15
when x = 15 p₀ = 85 – 5(15) = 85 – 75 = 10
C.S = \(\int _{0}^{x}\) f(x) dx – x₀p₀
= \(\int _{0}^{x}\) (85 – 5x) dx – (15)(10)

= 1275 – \(\frac { 1125 }{2}\) – 150
= 1275 – 562.50 – 150
= 1275 – 712.50
∴ CS = 562.50 units

Question 4.
The demand function for a commodity is p = e^-x. Find the consumer’s surplus when p = 0.5
Solution:
The demand function p = e^-x
when p = 0.5 ⇒ 0.5 = e^-x
\(\frac { 1 }{2}\) = \(\frac { 1 }{e^x}\) ⇒ e^x = 2
∴ x = log 2
∴ Consumer’s surplus
C.S = \(\int _{0}^{x}\) (demand function) dx – (Price × quantity demanded)

Question 5.
Calculate the producer’s surplus at x = 5 for the supply function p = 7 + x
Solution:
The supply function p = 7 + x
when x = 5 ⇒ p = 7 + 5 = 12
∴ x₀ = 5 and p₀ = 12
Producer’s surplus

Question 6.
If the supply function for a product is p = 3x + 5x². Find the producer’s surplus when x = 4
Solution:
The supply function p = 3x + 5x²
when x = 4 ⇒ p = 3(4) + 5(4)²
p = 12 + 5(16)= 12 + 80
p = 92
∴ x₀ = 4 and p₀ = 92
Producer’s Surplus

Question 7.
The demand function for a commodity is p = \(\frac { 36 }{x+4}\) Find the producer’s surplus when the prevailing market price is Rs 6.
Solution:
The demand function for a commodity
p = \(\frac { 36 }{x+4}\)
when p = 6 ⇒ 6 = \(\frac { 36 }{x+4}\)
x + 4 = \(\frac { 36 }{6}\) ⇒ x + 4 = 6
x = 2
∴ p₀ = 6 and x₀ = 2
The consumer’s surplus
C.S = \(\int _{0}^{x}\) f(x) dx – x₀p₀
= \(\int _{0}^{2}\) (\(\frac { 36 }{x+4}\)) dx – 2(6)
= 36 [log (x + 4)]\(_{0}^{2}\) – 12
= 36 [log (2 + 4) – log (0 + 4)] – 12
= 36 [log6 – log4] – 12
= 36[log(\(\frac { 6 }{4}\))] – 12
∴ CS = 36 log(\(\frac { 6 }{4}\)) – 12 units

Question 8.
The demand and supply functions under perfect competition are pd = 1600 – x² and p_s = 2x² + 400 respectively, find the producer’s surplus.
Solution:
pd = 1600 – x² and p_s = 2x² + 400
Under the perfect competition p_d = p_s
1600 – x² = 2x² + 400
1600 – 400 = 2x² + x² ⇒ 1200 = 3x²
⇒ x² – 400 ⇒ x = 20 or -20
The value of x cannot be negative, x = 20 when x₀ = 20;
p₀ = 1600 – (20)² = 1600 – 400
P₀ = 1200
PS = x₀p₀ – \(\int _{0}^{x_0}\) g(x) dx

Question 9.
Under perfect competition for a commodity the demand and supply laws are P_d = \(\frac { 8 }{x+1}\) – 2 and Ps = \(\frac { x+3 }{2}\) respectively. Find the consumer’s and producer’s surplus.
Solution:

16 – (x² + 3x + x + 3) = 2 [2(x + 1)]
16 – (x² + 4x + 3) = 4(x + 1)
16 – x² – 4x – 3 = 4x + 4
x² + 4x + 4x + 4 + 3 – 16 = 0
x² + 8x – 9 = 0
(x + 9) (x – 1) = 0 ⇒ x = -9 (or) x = 1
The value of x cannot be negative x = 1 when x₀ = 1
p₀ = \(\frac { 8 }{1+1}\) – 2 ⇒ p₀ = \(\frac { 8 }{2}\) – 2
p₀ = 4 – 2 ⇒ p₀ = 2
CS = \(\int _{0}^{x}\) f(x) dx – x₀p₀
= \(\int _{0}^{1}\) (\(\frac { 8 }{x+1}\) – 2) dx – (1) (2)
= {8[log(x + 1)] – 2x}\(\int _{0}^{1}\) – 2
= 8 {[log (1 + 1) – 2(1)] – 8 [log (0 + 1) – 2(0)]} – 2
= [8 log (2) – 2 – 8 log1] – 2
= 8 log(\(\frac { 8 }{2}\)) – 2 – 2
C.S = (8 log 2 – 4) units
P.S = x₀p₀ – \(\int _{0}^{x_0}\) g(x) dx

Question 10.
The demand equation for a products is x = \(\sqrt {100-p}\) and the supply equation is x = \(\frac {p}{2}\) – 10. Determine the consumer’s surplus and producer’s, under market equilibrium.
Solution:
p_d = \(\sqrt {100-p}\) and p_s = \(\sqrt {100-p}\)
Under market equilibrium, p_d = p_s
\(\sqrt {100-p}\) = \(\frac {p}{2}\) – 10
Squaring on both sides

36p = p² ⇒ p² – 36 p = 0
p (p – 36) = 0 ⇒ p = 0 or p = 36
The value of p cannot be zero, ∴p₀ = 36 when p₀ = 36
x₀ = \(\sqrt {100-36}\) = \(\sqrt {64}\)
∴ x₀ = 8

= 288 – [x² + 20x]\( _{0}^{8}\)
= 288 – { [(8)² + 20(8)] – [0]}
= 288 – [64 + 160]
= 288 – 224 = 64
PS = 64 Units

Question 11.
Find the consumer’s surplus and producer’s surplus for the demand function pd = 25 – 3x and supply function ps = 5 + 2x
Solution:
p_d = 25 – 3x and p_s = 5 + 2x
Under market equilibrium, p_d = p_s
25 – 3x = 5 + 2x
25 – 5 = 2x + 3x ⇒ 5x = 20
∴ x = 4
when x = 4
P₀ = 25 – 3(4)
= 25 – 12 = 13
p₀ = 13

= 52 – [5x + x²]\( _{0}^{4}\)
= 52 – (5(4) + (4)²) – (0)}
= 52 – [20 + 16]
= 52 – 36
∴ PS = 16 units

Try More:

What Is Nr7 Candle

Tamilnadu State Board New Syllabus Samacheer Kalvi 12th Business Maths Guide Pdf Chapter 3 Integral Calculus II Ex 3.2 Text Book Back Questions and Answers, Notes.

Tamilnadu Samacheer Kalvi 12th Business Maths Solutions Chapter 3 Integral Calculus II Ex 3.2

Question 1.
The cost of an overhaul of an engine is Rs 10,000 The Operating cost per hour is at the rate of 2x-240 where the engine has run x km. find out the total cost of the engine run for 300 hours after overhaul.
Solution:
Given that the overhaul cost is Rs. 10,000.
The marginal cost is 2x – 240
MC = 2x – 240
C = ∫ MC dx + k
C = x² – 240x + k
k is the overhaul cost
⇒ k = 10,000
So C = x² – 240x + 10,000
When x = 300 hours, total cost is
C = (300)² – 240(300) + 10,000
⇒ C = 90,000 – 72000 + 10,000
⇒ C = 28,000
So the total cost of the engine run for 300 hours after the overhaul is ₹ 28,000.

Question 2.
Elasticity of a function \(\frac { Ey }{Ex}\) is given by \(\frac { Ey }{Ex}\) = \(\frac { -7x }{(1-2x)(2+3x)}\). Find the function when x = 2, y = \(\frac { 3 }{8}\)
Solution:

Put x = 0
7 = A (3(0) + 2) + B (2(0) – 1)
7 = A (2) + B (-1)
7 = (2) (2) – B
B = 4 – 7
B = -3

Question 3.
The Elasticity of demand with respect to price for a commodity is given by where \(\frac { (4-x)}{x}\) p is the price when demand is x. find the demand function when the price is 4 and the demand is 2. Also, find the revenue function
Solution:
The elasticity at the demand

Integrating on both sides
∫\(\frac { 1}{(x-4)}\) = ∫\(\frac { 1}{p}\) dp
log |x – 4| = log |p| + log k
log |x – 4| = log |pk| ⇒ (x – 4) = pk ……… (1)
when p = 4 and x = 2
(2 – 4) = 4k ⇒ -2 = 4k
k = -1/2
Eqn (1) ⇒ (x – 4) = p(-1/2)
-2 (x – 4) = p ⇒ p = 8 – 2x
Revenue function R = px = (8 – 2x)x
R = 8x – 2x²

Question 4.
A company receives a shipment of 500 scooters every 30 days. From experience it is known that the inventory on hand is related to the number of days x. Since the shipment, I (x) = 500 – 0.03 x², the daily holding cost per scooter is Rs 0.3. Determine the total cost for maintaining inventory for 30 days
Solution:
Here I (x) = 500 – 0.03 x²
C¹ = Rs 0.3
T = 30
Total inventory carrying cost
= C¹ \(\int _{0}^{T}\) I(x) dx
= 0.3 \(\int _{0}^{30}\) (500 – 0.03 x²)dx
= 0.3 [500 x – 0.03(\(\frac { x^3 }{3}\))]\( _{0}^{30}\)
= 0.3 [ 500 x – 0.01 x³]\( _{0}^{30}\)
= 0.3 [500(30) – 0.01 (30)³] – [0]
= 0.3 [15000 – 0.01 (27000)]
= 0.3 [15000 – 270] = 0.3 [14730]
= Rs 4,419

Question 5.
An account fetches interest at the rate of 5% per annum compounded continuously an individual deposits Rs 1000 each year in his account. how much will be in the account after 5 years (e^0.25 = 1.284)
Solution:
P = 1000
r = \(\frac { 5 }{1000}\) = 0.05
N = 5
Annuity = \(\int _{0}^{5}\) 1000 e^0.05t dt
= 1000 [ \(\frac { e^{0.05t} }{0.05}\) ] \(_{0}^{5}\)
= \(\frac { 1000 }{0.05}\) [e^0.05(5) – e⁰]
= 20000 [e^0.25 – 1]
= 20000 [1.284 – 1]
= 20000 [0.284]
= Rs 5680

Question 6.
The marginal cost function of a product is given by \(\frac { dc }{dx}\) = 100 – 10x + 0.1 x² where x is the output. Obtain the total and average cost function of the firm under the assumption, that its fixed cost is t 500
Solution:
\(\frac { dc }{dx}\) = 100 – 10x + 0.1 x² and k = Rs 500
dc = (100 – 10x + 0.1 x²) dx
Integrating on both sides,

Question 7.
The marginal cost function is M.C = 300 x^2/5 and the fixed cost is zero. Find the total cost as a function of x
Solution:
M.C = 300 x^2/5 and fixed cost K = 0
Total cos t = ∫M.C dx

Question 8.
If the marginal cost function of x units of output is \(\frac { a }{\sqrt {ax+b}}\) and if the cost of output is zero. Find the total cost as a function of x.
Solution:

∴ C(x) = 2(ax + b)^1/2 + k …….. (1)
When x = 0
eqn (1) ⇒ 0 = 2 [a(0) + b]^1/2 + k
k = -2(b)^1/2 ⇒ k = -2√b
Required cost function
C(x) = 2(ax + b)^1/2 – 2√b
∴ C = 2\(\sqrt { ax + b}\) – 2√b

Question 9.
Determine the cost of producing 200 air conditioners if the marginal cost (is per unit) is C'(x) = \(\frac { x^2 }{200}\) + 4
Solution:

= 13333.33 + 800
∴ Cost of producing 200 air conditioners
= Rs 14133.33

Question 10.
The marginal revenue (in thousands of Rupees) function for a particular commodity is 5 + 3 e^-0.03x where x denotes the number of units sold. Determine the total revenue from the sale of 100 units (given e^-3 = approximately)
Solution:
The marginal Revenue (in thousands of Rupees) function
M.R = 5 + 3^-0.03x
Total Revenue from sale of 100 units is
Total Revenue T.R = \(\int _{0}^{ 100}\) M.R dx

= [500 – 100 e^-3] – [0 – 100 e°]
= [500 -100 (0.05)] – [-100 (1)]
= [500 – 5]+ 100
= 495 + 100 = 595 thousands
= 595 × 1000
∴ Revenue R = Rs 595000

Question 11.
If the marginal revenue function for a commodity is MR = 9 – 4x². Find the demand function.
Solution:
Marginal Revenue function MR = 9 – 4x²
Revenue function R = ∫MR dx

Question 12.
Given marginal revenue function \(\frac { 4 }{(2x+3)^2}\) -1, show that the average revenue function is P = \(\frac { 4 }{6x+9}\) -1
Solution:
M.R = \(\frac { 4 }{(2x+3)^2}\) -1
Total Revenue R = ∫M.R dx

Question 13.
A firms marginal revenue functions is M.R = 20 e^-x/10 Find the corresponding demand function.
Solution:

Question 14.
The marginal cost of production of a firm is given by C’ (x) = 5 + 0.13 x, the marginal revenue is given by R’ (x) = 18 and the fixed cost is Rs 120. Find the profit function.
Solution:
MC = C'(x) = 5 + 0.13x
C(x) = ∫C'(x) dx + k₁
= ∫(5 + 0.13x) dx + k₁
= 5x + \(\frac{0.13}{2} x^{2}\) + k₁
When quantity produced is zero, fixed cost is 120
(i.e) When x = 0, C = 120 ⇒ k₁ = 120
Cost function is 5x + 0.065x² + 120
Now given MR = R'(x) = 18
R(x) = ∫18 dx + k₂ = 18x + k₂
When x = 0, R = 0 ⇒ k₂ = 0
Revenue = 18x
Profit P = Total Revenue – Total cost = 18x – (5x + 0.065x² + 120)
Profit function = 13x – 0.065x² – 120

Question 15.
If the marginal revenue function is R'(x) = 1500 – 4x – 3x². Find the revenue function and average revenue function.
Solution:
Given marginal revenue function
MR = R’(x)= 1500 – 4x – 3x²
Revenue function R(x) = ∫R'(x) dx + c
R = ∫(1500 – 4x – 3x²) dx + c
R = 1500x – 2x² – x³ + c
When x = 0, R = 0 ⇒ c = 0
So R = 1500x – 2x² – x³
Average revenue function P = \(\frac{R}{x}\) ⇒ 1500 – 2x – x²

Question 16.
Find the revenue function and the demand function if the marginal revenue for x units MR = 10 + 3x – x
Solution:
The marginal revenue function
MR = 10 + 3x – x²
The Revenue function
R = ∫(MR) dx
= ∫(10 + 3x – x²)dx

Question 17.
The marginal cost function of a commodity is given by M_c = \(\frac { 14000 }{\sqrt{7x+4}}\) and the fixed cost is Rs 18,000. Find the total cost average cost.
Solution:
The marginal cost function of a commodity
M_c = \(\frac { 14000 }{\sqrt{7x+4}}\) = 14000 (7x + 4)^-1/2
Fixed cost k = Rs 18,000
Total cost function C = ∫(M.C) dx
= ∫14000 (7x + 4)^-1/2 dx

Question 18.
If the marginal cost (MC) of production of the company is directly proportional to the number of units (x) produced, then find the total cost function, when the fixed cost is Rs 5,000 and the cost of producing 50 units is Rs 5,625.
Solution:
M.C αx
M.C = λx
fixed cost k = Rs 5000
Cost function C = ∫(M.C) dx
= ∫λx dx

Question 19.
If MR = 20 – 5x + 3x², Find total revenue function
Solution:
MR = 20 – 5x + 3x²
Total Revenue function
R = ∫(MR) dx = ∫(20 – 5x + 3x²) dx
R = 20x – \(\frac { 5x^2 }{2}\) + \(\frac {3x^3 }{3}\) + k
when x = 0; R = 0 ⇒ k = 0
∴ R = 20x – \(\frac { 5 }{2}\) x² + x³

Question 20.
If MR = 14 – 6x + 9x², Find the demand function.
Solution:
MR = 14 – 6x + 9x²
R = ∫(14 – 6x + 9x²) dx + k
= 14x – 3x² + 3x³ + k
Since R = 0, when x = 0, k = 0
So revenue function R = 14x – 3x² + 3x³
Demand function P = \(\frac{R}{x}\) = 14 – 3x + 3x²

Must Try:

Nifty Resistance Support Levels Calculator

Tamilnadu State Board New Syllabus Samacheer Kalvi 12th Business Maths Guide Pdf Chapter 2 Integral Calculus I Ex 2.12 Text Book Back Questions and Answers, Notes.

Tamilnadu Samacheer Kalvi 12th Business Maths Solutions Chapter 2 Integral Calculus I Ex 2.12

Choose the most suitable answer from the given four alternatives:

Question 1.
∫\(\frac { 1 }{x^3}\) dx is
(a) \(\frac { -3 }{x^2}\) + c
(b) \(\frac { -1 }{2x^2}\) + c
(c) \(\frac { -1 }{3x^2}\) + c
(d) \(\frac { -2 }{x^2}\) + c
Solution:
(b) \(\frac { -1 }{2x^2}\) + c
Hint:
∫\(\frac { 1 }{x^3}\) dx = ∫x^-3 dx = [ \(\frac { x^{-3+1} }{-3+1}\) ] + c
= (\(\frac { x^{-2} }{-2}\)) + c = \(\frac { -1 }{2x^2}\) + c

Question 2.
∫2^x dx is
(a) 2^x log 2 + c
(b) 2^x + c
(c) \(\frac { 2^x }{log 2}\) + c
(d) \(\frac { log 2 }{2^x}\) + c
Solution:
(c) \(\frac { 2^x }{log 2}\) + c
Hint:
∫2^x dx = ∫a^x dx = \(\frac { a^x }{log a}\) + c

Question 3.
∫\(\frac { sin 2x }{2 sin x}\) dx is
(a) sin x + c
(b) \(\frac { 1 }{2}\) sin x + c
(c) cos x + c
(d) \(\frac { 1 }{2}\) cos x + c
Solution:
(a) sin x + c
Hint:
∫\(\frac { sin 2x }{2 sin x}\) dx = ∫\(\frac { 2sin x cos x }{2 sin x}\) dx
= ∫cos x dx
= sin x + c

Question 4.
∫\(\frac { sin 5x-sin x }{cos 3x}\) dx is
(a) -cos 2x + c
(b) -cos 2x – c
(c) –\(\frac { 1 }{4}\) cos 2x + c
(d) -4 cos 2x + c
Solution:
(a) -cos 2x + c
Hint:

Question 5.
∫\(\frac { log x}{x}\) dx, x > 0 is
(a) \(\frac { 1 }{2}\) (log x)² + c
(b) –\(\frac { 1 }{2}\) (log x)²
(c) \(\frac { 2 }{x^2}\) + c
(d) \(\frac { 2 }{x^2}\) – c
Solution:
(a) \(\frac { 1 }{2}\) (log x)² + c
Hint:
∫\(\frac { log x}{x}\) dx, x > 0
∫ tdt = [ \(\frac { t^2 }{2}\) ] + c
= \(\frac { (log x)^2 }{2}\) + c
let t = log x
\(\frac { dt }{dx}\) = \(\frac { 1 }{x}\)
dt = \(\frac { 1 }{x}\) dx

Question 6.
∫\(\frac { e^x }{\sqrt{1+e^x}}\) dx is
(a) \(\frac { e^x }{\sqrt{1+e^x}}\) + c
(b) 2\(\sqrt{1+e^x}\) + c
(c) \(\sqrt{1+e^x}\) + c
(d) e^x\(\sqrt{1+e^x}\) + c
Solution:
(b) 2\(\sqrt{1+e^x}\) + c
Hint:

Question 7.
∫\(\sqrt { e^x}\) dx is
(a) \(\sqrt { e^x}\) + c
(b) 2\(\sqrt { e^x}\) + c
(c) \(\frac { 1 }{2}\) \(\sqrt { e^x}\) + c
(d) \(\frac { 1 }{2\sqrt { e^x}}\) + c
Solution:
(b) 2\(\sqrt { e^x}\) + c
Hint:
∫\(\sqrt { e^x}\) dx
= ∫\(\sqrt { e^x}\) dx = ∫(e^x)^1/2 dx = ∫ e^x/2 dx
= \(\frac { e^{x/2} }{1/2}\) + c = 2e^x/2 + c
= 2(e^x)^1/2 + c = 2\(\sqrt { e^x}\) + c

Question 8.
∫e^2x [2x² + 2x] dx
(a) e^2x x² + c
(b) xe^2x + c
(c) 2x²e² + c
(d) \(\frac { x^2e^x }{2}\) + c
Solution:
(a) e^2x x² + c
Hint:
∫e^2x (2x² + 2x) dx
Let f(x) = x²; f'(x) = 2x and a = 2
= ∫e^ax [af(x),+ f ’(x)] = e^ax f(x) + c
= ∫e^2x (2x² + 2x) dx = e^2x (x²) + c

Question 9.
\(\frac { e^x }{e^x+1}\) dx is
(a) log |\(\frac { e^x }{e^x+1}\)| + c
(b) log |\(\frac { e^x+1 }{e^x}\)| + c
(c) log |e^x| + c
(d) log |e^x + 1| + c
Solution:
(d) log |e^x + 1| + c
Hint:
∫\(\frac { e^x }{e^x+1}\) dx
= ∫\(\frac { dt }{t}\)
= log |t| + c
= log |e^x + 1| + c
take t = e^x + 1
\(\frac { dt }{dx}\) = e^x
dt = e^x dx

Question 10.
∫\(\frac { 9 }{x-3}-\frac { 1 }{x+1}\) dx is
(a) log |x – 3| – log|x + 1| + c
(b) log|x – 3| + log|x + 1| + c
(c) 9 log |x – 3| – log |x + 1| + c
(d) 9 log |x – 3| + log |x + 1| + c
Solution:
(c) 9 log |x – 3| – log |x + 1| + c
Hint:

Question 11.
∫\(\frac { 2x^3 }{4+x^4}\) dx is
(a) log |4 + x⁴| + c
(b) \(\frac { 1 }{2}\) log |4 + x⁴| + c
(c) \(\frac { 1 }{2}\) log |4 + x⁴| + c
(d) log |\(\frac { 2x^3 }{4+x^4}\) + c
Solution:
(b) \(\frac { 1 }{2}\) log |4 + x⁴| + c
Hint:

Question 12.
∫\(\frac { dx }{\sqrt{x^2-36}}\) is
(a) \(\sqrt{x^2-36}\) + c
(b) log |x + \(\sqrt{x^2-36}\)| + c
(c) log |x – \(\sqrt{x^2-36}\)| + c
(d) log |x² + \(\sqrt{x^2-36}\)| + c
Solution:
(b) log |x + \(\sqrt{x^2-36}\)| + c
Hint:

Question 13.
∫\(\frac { 2x+3 }{\sqrt{x^2+3x+2}}\) dx is
(a) \(\sqrt{x^2+3x+2}\) + c
(b) 2\(\sqrt{x^2+3x+2}\) + c
(c) \(\sqrt{x^2+3x+2}\) + c
(d) \(\frac { 2 }{3}\) (x² + 3x + 2) + c
Solution:
(b) 2\(\sqrt{x^2+3x+2}\) + c
Hint:

Question 14.
\(\int_{0}^{4}\) (2x + 1) dx is
(a) 1
(b) 2
(c) 3
(d) 4
Solution:
(b) 2
Hint:
\(\int_{0}^{4}\) (2x + 1) dx
= [2(\(\frac { x^2 }{2}\)) + x]\(_{0}^{1}\) = [x² + x]\(_{0}^{1}\)
= [(1)² + (1)] – [0] = 2

Question 15.
\(\int_{2}^{4}\) \(\frac { dx }{x}\) is
(a) log 4
(b) 0
(c) log 2
(d) log 8
Solution:
(c) log 2
Hint:
\(\int_{2}^{4}\) \(\frac { dx }{x}\)
\(\int_{2}^{4}\) \(\frac { dx }{x}\) = [log |x|]\(_{0}^{1}\) = log |4| – log |2|
= log[ \(\frac { 4}{2}\) ] = log 2

Question 16.
\(\int_{0}^{∞}\) e^-2x dx is
(a) 0
(b) 1
(c) 2
(d) \(\frac { 1 }{2}\)
Solution:
(d) \(\frac { 1 }{2}\)
Hint:

Question 17.
\(\int_{-1}^{1}\) x³ e^x4 dx is
(a) 1
(b) 2\(\int_{0}^{1}\) x³ e^x4
(c) 0
(d) e^x4
Solution:
(c) 0
Hint:
\(\int_{-1}^{1}\) x³ e^x4 dx
Let f (x) = x³e^x4
f(-x) = (-x)² e^(-x)4
= -x² e^x4
f(-x) = -f(x)
⇒ f(x) is an odd function
∴ \(\int_{-1}^{1}\) x³ e^x4 dx = 0

Question 18.
If f(x) is a continuous function and a < c < b, then \(\int_{a}^{c}\) f(x) dx + \(\int_{c}^{b}\) f(x) dx is
(a) \(\int_{a}^{b}\) f(x) dx – \(\int_{a}^{c}\) f(x) dx
(b) \(\int_{a}^{c}\) f(x) dx – \(\int_{a}^{b}\) f(x) dx
(c) \(\int_{a}^{b}\) f(x) dx
(d) 0
Solution:
(c) \(\int_{a}^{b}\) f(x) dx

Question 19.
The value of \(\int_{-π/2}^{π/2}\) cos x dx is
(a) 0
(b) 2
(c) 1
(d) 4
Solution:
(b) 2
Hint:
\(\int_{-π/2}^{π/2}\) cos x dx
Let f(x) = cos x
f(-x) = cos (-x) = cos (x) = f(x)
∴ f(x) is an even function
\(\int_{-π/2}^{π/2}\) cos x dx = 2 × \(\int_{0}^{π/2}\) cos x dx
= 2 × [sin x]\(_{0}^{-π/2}\) = 2 [sin π/2 – sin 0]
= 2 [1 – 0] = 2

Question 20.
\(\int_{-π/2}^{π/2}\) \(\sqrt {x^4(1-x)^2}\) dx
(a) \(\frac { 1 }{12}\)
(b) \(\frac { -7 }{12}\)
(c) \(\frac { 7 }{12}\)
(d) \(\frac { -1 }{12}\)
Solution:
(a) \(\frac { 1 }{12}\)
Hint:

Question 21.
If \(\int_{0}^{1}\) f(x) dx = 1, \(\int_{0}^{1}\) x f(x) dx = a and \(\int_{0}^{1}\) x² f(x) dx = a², then \(\int_{0}^{1}\) (a – x)² f(x) dx is
(a) 4a²
(b) 0
(c) a²
(d) 1
Solution:
(b) 0
Hint:
\(\int_{0}^{1}\) (a – x)² f(x) dx
= \(\int_{0}^{1}\) [a² +x² – 2ax] f(x) dx
= \(\int_{0}^{1}\) a² + f (x) dx + \(\int_{0}^{1}\) x² f (x) dx – 2a\(\int_{0}^{1}\) x f(x) dx
= a²(1) + a² – 2a(a) – 2a² – 2a² = 0

Question 22.
The value of \(\int_{2}^{3}\) f(5 – x) dx – \(\int_{2}^{3}\) f(x) dx is
(a) 1
(b) 0
(c) -1
(d) 5
Solution:
(b) 0
Hint:
\(\int_{2}^{3}\) f(5 – x) dx – \(\int_{2}^{3}\) f(x) dx
Using the property
= \(\int_{2}^{3}\) f(x) dx = \(\int_{a}^{b}\) f(a + b – x) dx
= \(\int_{2}^{3}\) f (5 – x) – \(\int_{2}^{3}\) f (5 – x) dx
= 0

Question 23.
\(\int_{0}^{4}\) (√x + \(\frac { 1 }{√x}\)), dx is
(a) \(\frac { 20 }{3}\)
(b) \(\frac { 21 }{3}\)
(c) \(\frac { 28 }{3}\)
(d) \(\frac { 1 }{3}\)
Solution:
(c) \(\frac { 28 }{3}\)
Hint:

Question 24.
\(\int_{0}^{π/3}\) tan x dx is
(a) log 2
(b) 0
(c) log √2
(d) 2 log 2
Solution:
(a) log 2
Hint:
\(\int_{0}^{π/3}\) tan x dx
= ∫tan x dx
= ∫\(\frac { sin x }{cos x}\) dx
= -∫\(\frac { -sin x }{cos x}\) dx
= -log |cos x| + c
= log sec x + c
= [log (sec x)]\(_{0}^{π/3}\)
= log [(sec π/3) – log (sec 0)]
= log (2) – log (1)
= log 2 – (0) = log 2

Question 25.
Using the factorial representation of the gamma function, which of the following is the solution for the gamma function Γ(n) when n = 8
(a) 5040
(b) 5400
(c) 4500
(d) 5540
Solution:
(a) 5040
Hint:
\(\Upsilon\) (8) = 7! = 7 × 6 × 5 × 4 × 3 × 2 × 1 = 5040

Question 26.
Γ(n) is
(a) (n – 1)!
(b) n!
(c) n Γ (n)
(d) (n – 1) Γ(n)
Solution:
(a) (n – 1)!
Hint:
Γ(n) = Γ(n – 1) + 1 = (n – 1)!

Question 27.
Γ(1) is
(a) 0
(b) 1
(c) n
(d) n!
Solution:
(b) 1
Hint:
\(\Upsilon\) (1) = 0! = 1

Question 28.
If n > 0, then Γ(n) is
(a) \(\int_{0}^{1}\) e^-x x^n-1 dx
(b) \(\int_{0}^{1}\) e^-x xⁿ dx
(c) \(\int_{0}^{∞}\) e^x x^-n dx
(d) \(\int_{0}^{∞}\) e^-x x^n-1 dx
Solution:
(d) \(\int_{0}^{∞}\) e^-x x^n-1 dx

Question 29.
Γ(\(\frac { 3 }{2}\))
(a) √π
(b) \(\frac { √π }{2}\)
(c) 2√π
(d) \(\frac { 3 }{2}\)
Solution:
(b) \(\frac { √π }{2}\)
Hint:
\(\Upsilon\) (3/2) = \(\frac { 2 }{2}\) \(\Upsilon\) [ \(\frac { 3 }{2}\) ]
= \(\frac { 3 }{2}\) √π

Question 30.
\(\int_{0}^{∞}\) x⁴ e^-x dx is
(a) 12
(b) 4
(c) 4!
(d) 64
Solution:
(b) \(\frac { √π }{2}\)
Hint:
\(\int_{0}^{∞}\) x⁴ e^-x dx
= ∫xⁿ e^-ax dx = \(\frac { n! }{a{n+1}}\)
= \(\frac { 4! }{(1)^{n+1}}\)
= \(\frac { 4! }{(1)^5}\)
= 4!

Must Read:

Gann Square Calculator

Tamilnadu State Board New Syllabus Samacheer Kalvi 12th Business Maths Guide Pdf Chapter 2 Integral Calculus I Miscellaneous Problems Text Book Back Questions and Answers, Notes.

Tamilnadu Samacheer Kalvi 12th Business Maths Solutions Chapter 2 Integral Calculus I Miscellaneous Problems

Question 1.
∫\(\frac { 1 }{\sqrt{x-1}-\sqrt{x+3}}\) dx
Solution:
∫\(\frac { 1 }{\sqrt{x+2}-\sqrt{x+3}}\) dx
Conjugating the Denominator

Question 2.
∫\(\frac { dx }{2-3x-2x^2}\)
Solution:

Question 3.
∫\(\frac { dx }{e^x+6+5e^{-x}}\)
Solution:

Question 4.
∫\(\sqrt { 2x^2-3}\) dx
Solution:

Question 5.
∫\(\sqrt { 9x^2+12x+3}\) dx
Solution:

Question 6.
∫(x + 1)² log x dx
Solution:
∫udv = uv – ∫vdu
∫(x + 1)² log x dx

Question 7.
∫log (x – \(\sqrt { x^2-1}\)) dx
Solution:

Question 8.
\(\int_{0}^{1}\) \(\sqrt { x(x-1)}\) dx
Solution:

Question 9.
\(\int_{-1}^{1}\) x² e^-2x dx
Solution:

Question 10.
\(\int_{0}^{3}\) \(\frac { xdx }{\sqrt {x+1} + \sqrt{5x+1}}\)
Solution:

Try More:

MARICO Pivot Point Calculator

Tamilnadu State Board New Syllabus Samacheer Kalvi 12th Business Maths Guide Pdf Chapter 2 Integral Calculus I Ex 2.11 Text Book Back Questions and Answers, Notes.

Tamilnadu Samacheer Kalvi 12th Business Maths Solutions Chapter 2 Integral Calculus I Ex 2.11

Evaluate the following integrals as the limit of the sum:

Question 1.
\(\int_{0}^{1}\) (x + 4) dx
Solution:

Question 2.
\(\int_{1}^{3}\) x dx
Solution:

Question 3.
\(\int_{1}^{3}\) (2x + 3) dx
Solution:

Question 4.
\(\int_{0}^{1}\) x² dx
Solution:

Try More:

MANAPPURAM Pivot Point Calculator