Students can download Maths Chapter 2 Real Numbers Ex 2.5 Questions and Answers, Notes, Samacheer Kalvi 9th Maths Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus, helps students complete homework assignments and to score high marks in board exams.
Tamilnadu Samacheer Kalvi 9th Maths Solutions Chapter 2 Real Numbers Ex 2.5
Question 1.
Write the following in the form of \(5^n\):
(i) 625
(ii) \(\frac{1}{5}\)
(iii) \(\sqrt{5}\)
(iv) \(\sqrt{125}\)
Solution:
(i) 625 = 54
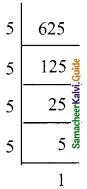
(ii) \(\frac{1}{5}\) = 5-1
(iii) \(\sqrt{5}\) = \(5^\frac{1}{2}\)
(iv) \(\sqrt{125}\) = \(\sqrt{5^3}\) = \((5^3)^\frac{1}{2} = 5^\frac{3}{2}\)
![]()
Question 2.
Write the following in the form of \(4^n\):
(i) 16
(ii) 8
(iii) 32
Solution:
(i) 16
= 4 × 4
= 4²
(ii) 8
= 4 × 2
= 4 × \(\left(2^{2}\right)^{\frac{1}{2}} \)
= 4 \(\times 4^{\frac{1}{2}} \)
= 4\(^{1+\frac{1}{2}} \)
= 4\(^{\frac{2+1}{2}} \)
= 4\(^{3 / 2}\)
(iii) 32
= 4 × 4 × 2
= 4² × \(\left(2^{2}\right)^{\frac{1}{2}} \)
= 4\(^{2} \times 4^{\frac{1}{2}} \)
= 4\(^{2+\frac{1}{2}} \)
= 4\(^{\frac{4+1}{2}} \)
= 4\(^{\frac{5}{2}} \)
![]()
Question 3.
Find the value of
(i) (49)\(^\frac{1}{2}\)
(ii) (243)\(^\frac{2}{5}\)
(iii) (9)\(^\frac{-3}{2}\)
(iv) \((\frac{64}{125})^\frac{-2}{3}\)
Solution:
(i) 49\(^\frac{1}{2}\) = \((7^2)^\frac{1}{2}\) = 7\(^{2 × \frac{1}{2}}\) = 7
(ii) (243)\(^\frac{2}{5}\) = \((3^5)^\frac{2}{5}\) = 3\(^{5 × \frac{2}{5}}\) = 3² = 9

(iii) \(9^{\frac{-3}{2}}=\left(3^{2}\right)^{\frac{-3}{2}}=3^{2 \times \frac{-3}{2}}=3^{-3}=\frac{1}{3^{3}}=\frac{1}{27}\)
(iv) \(\left(\frac{64}{125}\right)^{\frac{-2}{3}}=\left(\frac{4^{3}}{5^{3}}\right)^{\frac{-2}{3}}=\left[\left(\frac{4}{5}\right)^{3}\right]^{\frac{-2}{3}}=\left(\frac{4}{5}\right)^{3 \times \frac{-2}{3}}=\left(\frac{4}{5}\right)^{-2}=\frac{4^{-2}}{5^{-2}}=\frac{5^{2}}{4^{2}}=\frac{25}{16} \)
![]()
Question 4.
Use a fractional index to write:
(i) \(\sqrt{5}\)
(ii) \(\sqrt[2]{7}\)
(iii) (\(\sqrt[3]{49})^{5}\)
(iv) \((\frac{1}{\sqrt[3]{100}})^{7}\)
Solution:
(i) \(\sqrt{5}\) = (5)\(^\frac{1}{2}\)
(ii) \(\sqrt[2]{7}\) = 7\(^\frac{1}{2}\)
(iii) \((\sqrt[3]{49})^{5}=\left[(49)^{\frac{1}{3}}\right]^{5}=\left[\left(7^{2}\right)^{\frac{1}{3}}\right]^{5}=\left(7^{\frac{2}{3}}\right)^{5}=7^{\frac{2}{3} \times 5}=7^{\frac{10}{3}}\)
(iv) \(\left(\frac{1}{\sqrt[3]{100}}\right)^{7}=\left[\frac{1}{\sqrt[3]{10^{2}}}\right]^{7}=\left[\frac{1}{\left(10^{2}\right)^{1 / 3}}\right]^{7}=\left[\frac{1}{10^{2 / 3}}\right]^{7}=\left(10^{\frac{-2}{3}}\right)^{7}=10^{\frac{-2}{3} \times 7}=10^{\frac{-14}{3}}\)
![]()
Question 5.
Find the 5th root of:
(i) 32
(ii) 243
(iii) 100000
(iv) \(\frac{1024}{3125}\)
Solution:
(i) \(\sqrt[5]{32}=(32)^{\frac{1}{5}}=\left(2^{5}\right)^{\frac{1}{5}}=2^{5 \times \frac{1}{5}} \) = 2
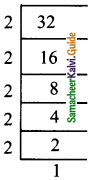
(ii) \(\sqrt[5]{243}=(243)^{\frac{1}{5}}=\left(3^{5}\right)^{\frac{1}{5}}=3^{5 \times \frac{1}{5}}\) = 3
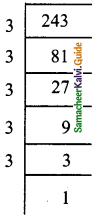
(iii) \(\sqrt[5]{100000}=(100000)^{\frac{1}{5}}=\left(10^{5}\right)^{\frac{1}{5}}\)
= \(10^{5}\times{\frac{1}{5}}\)
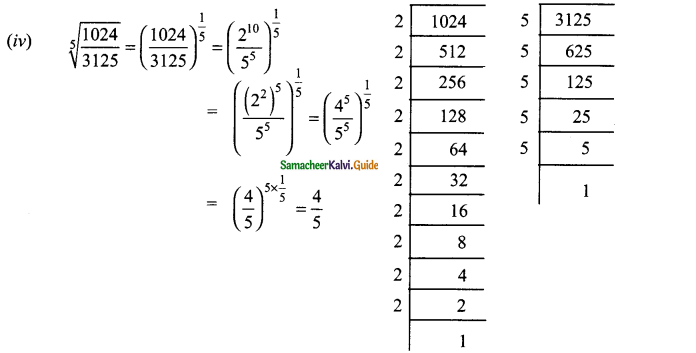
![]()