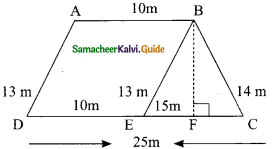
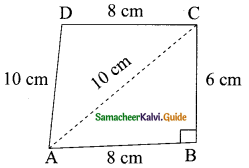
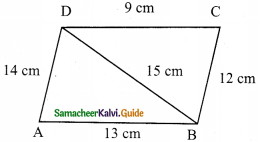
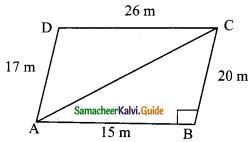
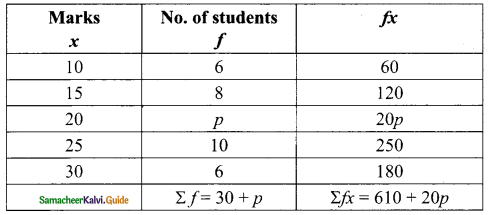
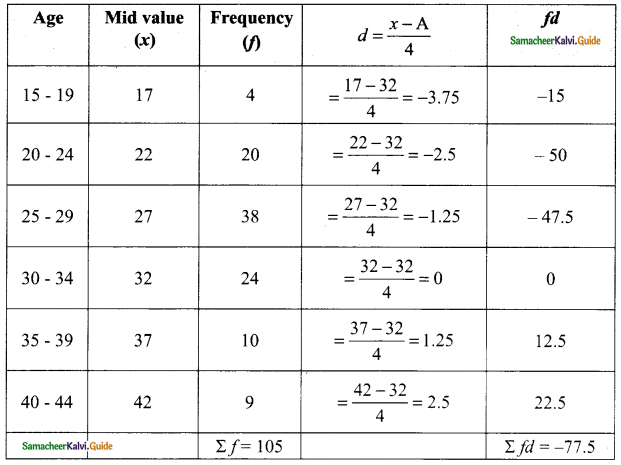
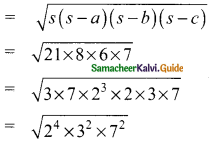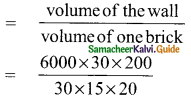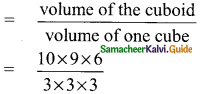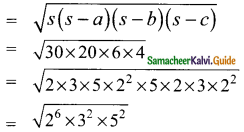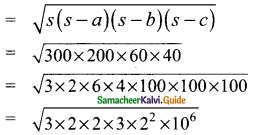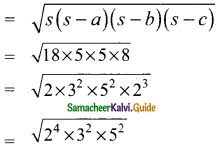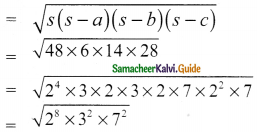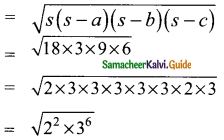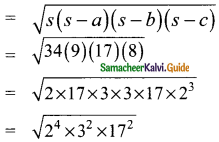Class 9 – Samacheer Kalvi

Samacheer Kalvi 9th Tamil Guide Book Back Answers Solutions

December 1, 2025

Subject Matter Experts at SamacheerKalvi.Guide have created Tamil Nadu State Board Samacheer Kalvi 9th Tamil Book Answers Solutions Guide Pdf Free Download are part of Samacheer Kalvi 9th Books Solutions.

Let us look at these TN State Board New Syllabus Samacheer Kalvi 9th Std Tamil Guide Pdf of Text Book Back Questions and Answers Term 1, 2, 3, Chapter Wise Important Questions, Study Material, Question Bank, Notes, and revise our understanding of the subject.

Samacheer Kalvi 9th Tamil Book Solutions Guide Pdf Free Download

Tamilnadu State Board Samacheer Kalvi 9th Tamil Book Back Answers Solutions Guide Term 1, 2, 3.

Samacheer Kalvi 9th Tamil Book Back Answers

We hope these Tamilnadu State Board Samacheer Kalvi Class 9th Tamil Book Solutions Answers Guide Pdf Free Download will help you get through your subjective questions in the exam.

Let us know if you have any concerns regarding TN State Board New Syllabus Samacheer Kalvi 9th Standard Tamil Guide Pdf of Text Book Back Questions and Answers, Chapter Wise Important Questions, Study Material, Question Bank, Notes, drop a comment below and we will get back to you as soon as possible.

Samacheer Kalvi 9th English Guide Book Back Answers Solutions

December 1, 2025

Subject Matter Experts at SamacheerKalvi.Guide have created Tamil Nadu State Board Samacheer Kalvi 9th English Book Answers Solutions Guide Pdf Free Download are part of Samacheer Kalvi 9th Books Solutions.

Let us look at these TN State Board New Syllabus Samacheer Kalvi 9th Std English Guide Pdf of Text Book Back Questions and Answers, Chapter Wise Important Questions, Study Material, Question Bank, Notes, and revise our understanding of the subject.

Samacheer Kalvi 9th English Book Solutions Guide Pdf Free Download

Samacheer Kalvi 9th English Book Back Answers

Tamilnadu State Board Samacheer Kalvi 9th English Book Back Answers Solutions Guide.

Samacheer Kalvi 9th English Book Solutions Prose

Samacheer Kalvi 9th English Book Solutions Poem

Samacheer Kalvi 9th English Book Solutions Supplementary

We hope these Tamilnadu State Board Samacheer Kalvi Class 9th English Book Solutions Answers Guide Pdf Free Download will help you get through your subjective questions in the exam.

Let us know if you have any concerns regarding TN State Board New Syllabus Samacheer Kalvi 9th Standard English Guide Pdf of Text Book Back Questions and Answers, Chapter Wise Important Questions, Study Material, Question Bank, Notes, drop a comment below and we will get back to you as soon as possible.

Samacheer Kalvi 9th Books Solutions Guide

December 1, 2025

Subject Matter Experts at SamacheerKalvi.Guide have created Tamil Nadu State Board Samacheer Kalvi 9th Books Answers Solutions Guide Pdf Free Download in English Medium and Tamil Medium are part of Samacheer Kalvi Books Solutions.

Let us look at these TN State Board New Syllabus Samacheer Kalvi 9th Std Guide Pdf of Text Book Back Questions and Answers Term 1, 2, 3, Chapter Wise Important Questions, Study Material, Question Bank, Notes, Formulas and revise our understanding of the subject.

Tamilnadu Samacheer Kalvi 9th Guide Text Book Back Answers Solutions Pdf Free Download

TN Samacheer Kalvi 9th Book Back Answers Solutions Guide

We hope these Tamilnadu State Board Samacheer Kalvi Class 9th Std Books Solutions Answers Guide Pdf Free Download in English Medium and Tamil Medium will help you get through your subjective questions in the exam.

Let us know if you have any concerns regarding TN State Board New Syllabus Samacheer Kalvi 9th Standard Guides Pdf of Text Book Back Questions and Answers Term 1, 2, 3, Chapter Wise Important Questions, Study Material, Question Bank, Notes, Formulas, drop a comment below and we will get back to you as soon as possible.

Samacheer Kalvi 9th Social Science Guide Book Back Answers Solutions

December 1, 2025

Subject Matter Experts at SamacheerKalvi.Guide have created Tamil Nadu State Board Samacheer Kalvi 9th Social Science Book Answers Solutions Guide Pdf Free Download of of History, Geography, Civics, Economics in English Medium and Tamil Medium are part of Samacheer Kalvi 9th Books Solutions.

Let us look at these TN State Board New Syllabus Samacheer Kalvi 9th Std Social Science Guide Pdf of Text Book Back Questions and Answers Volume 1, 2, Chapter Wise Important Questions, Study Material, Question Bank, Notes, Formulas and revise our understanding of the subject.

Samacheer Kalvi 9th Social Science Book Solutions Guide Pdf Free Download

Samacheer Kalvi 9th Social Science Book Back Answers

Tamilnadu State Board Samacheer Kalvi 9th Social Science Book Back Answers Solutions Guide.

Samacheer Kalvi 9th Social Science History Book Solutions

Samacheer Kalvi 9th Social Science Geography Book Answers

Samacheer Kalvi 9th Social Science Guide Civics

Samacheer Kalvi 9th Social Science Guide Economics

9th Social Science Guide Book Back Questions Tamil Medium with Answers

9th Social Science Book Back Questions Tamil Medium with Answers, 9th Std Social Science Guide in Tamil Medium Pdf Free Download 2021-2022, 9th New Social Science Book Back Answers in Tamil Medium.

TN 9th Social Guide History

9th Std Social Science Book Back Answers Geography

9th Std Social Science Guide Civics

9th Standard Social Science Guide Economics

We hope these Tamilnadu State Board Samacheer Kalvi Class 9th Social Science Book Solutions Answers Guide Pdf Free Download of History, Geography, Civics, Economics in English Medium and Tamil Medium will help you get through your subjective questions in the exam.

Let us know if you have any concerns regarding TN State Board New Syllabus Samacheer Kalvi 9th Standard Social Science Guide Pdf of Text Book Back Questions and Answers Volume 1, 2, Chapter Wise Important Questions, Study Material, Question Bank, Notes, Formulas, drop a comment below and we will get back to you as soon as possible.

Samacheer Kalvi 9th Maths Guide Book Back Answers Solutions

December 1, 2025

Subject Matter Experts at SamacheerKalvi.Guide have created Tamilnadu State Board Samacheer Kalvi 9th Maths Book Answers and Solutions Guide Pdf Free Download in English Medium and Tamil Medium are part of Samacheer Kalvi 9th Books Solutions.

Let us look at these TN State Board New Syllabus Samacheer Kalvi 9th Std Maths Guide Pdf of Book Back Questions and Answers Term 1, 2, 3, Chapter Wise Important Questions, Study Material, Question Bank, Notes, Formulas and revise our understanding of the subject.

Samacheer Kalvi 9th Maths Book Solutions Guide Pdf Free Download

Tamilnadu State Board Samacheer Kalvi 9th Maths Book Back Answers Solutions Guide Term 1, 2, 3.

Samacheer Kalvi 9th Maths Book Back Answers

Samacheer Kalvi 9th Maths Book with Answers Chapter 1 Set Language

Samacheer Kalvi 9th Maths Book Solutions Chapter 2 Real Numbers

TN 10th Maths Guide Samacheer Kalvi Pdf Free Download Chapter 3 Algebra

Samacheer Kalvi 9th Maths Guide Pdf Free Download Chapter 4 Geometry

Samacheer Kalvi 9th Maths Book English Medium Chapter 5 Coordinate Geometry

9th Standard Samacheer Kalvi Maths Guide Free Download Chapter 6 Trigonometry

Samacheer Kalvi 9th Maths Guide Online Chapter 7 Mensuration

Tamilnadu Board Class 9 Maths Chapter 8 Statistics

Tamil Nadu State Board 9th Std Maths Chapter 9 Probability

9th Standard Maths Guide Tamil Medium Pdf Free Download

Samacheer Kalvi 9th Maths Guide Tamil Medium Pdf Free Download, 9th Maths Guide in Tamil Medium Pdf Download 2020-2021.

Samacheer Kalvi 9th Maths Book Back Answers Chapter 1 கண மொழி

Tamilnadu State Board 9th Maths Samacheer Solutions Chapter 2 மெய்யெண்கள்

Samacheer Kalvi Maths Guide For 9th Std Chapter 3 இயற்கணிதம்

9th Standard Maths Answer Key Chapter 4 வடிவியல்

9th Class Maths Guide State Syllabus Pdf ஆயத்தொலை வடிவியல்

9th Samacheer Maths Solution Book Chapter 6 முக்கோணவியல்

Samacheer 9th Maths Guide Tamil Medium Pdf Free Download Chapter 7 அளவியல்

Samacheer Kalvi Class 9 Maths Solutions Chapter 8 புள்ளியியல்

9th Standard Samacheer Kalvi Maths Guide Chapter 9 நிகழ்தகவு

We hope these Tamilnadu State Board Samacheer Kalvi Class 9th Maths Book Solutions and Answers Pdf Free Download in English Medium and Tamil Medium will help you get through your subjective questions in the exam.

Let us know if you have any concerns regarding TN State Board New Syllabus Samacheer Kalvi 9th Standard Maths Guide Pdf of Book Back Questions and Answers Term 1, 2, 3, Chapter Wise Important Questions, Study Material, Question Bank, Notes, Formulas drop a comment below and we will get back to you as soon as possible.

Samacheer Kalvi 9th Science Guide Book Back Answers Solutions

December 1, 2025

Subject Matter Experts at SamacheerKalvi.Guide have created Tamil Nadu State Board Samacheer Kalvi 9th Science Book Answers Solutions Guide Pdf Free Download in English Medium and Tamil Medium are part of Samacheer Kalvi 9th Books Solutions.

Let us look at these TN State Board New Syllabus Samacheer Kalvi 9th Std Science Guide Pdf of Text Book Back Questions and Answers Term 1, 2, 3, Chapter Wise Important Questions, Study Material, Question Bank, Notes, and revise our understanding of the subject.

Samacheer Kalvi 9th Science Book Solutions Guide Pdf Free Download

Samacheer Kalvi 9th Science Book Back Answers

Tamilnadu State Board Samacheer Kalvi 9th Science Book Back Answers Solutions Guide Term 1, 2, 3.

Samacheer Kalvi 9th Science Physics Book Solutions

Samacheer Kalvi 9th Science Chemistry Book Solutions

Samacheer Kalvi 9th Science Biology Book Solutions

Samacheer Kalvi 9th Science Computer Science Book Solutions

9th New Science Book Back Questions Tamil Medium with Answers

9th Science Book Back Questions Tamil Medium with Answers, 9th Std Science Guide Pdf Download 2021 Tamil Medium, 9th New Science Book Back Answers in Tamil 2021-2022.

Samacheer Kalvi 9th Science Physics Book Solutions

Samacheer Kalvi 9th Science Chemistry Book Solutions

Samacheer Kalvi 9th Science Biology Book Solutions

Samacheer Kalvi 9th Science Computer Science

We hope these Tamilnadu State Board Samacheer Kalvi Class 9th Science Book Solutions Answers Guide Pdf Free Download in English Medium and Tamil Medium will help you get through your subjective questions in the exam.

Let us know if you have any concerns regarding TN State Board New Syllabus Samacheer Kalvi 9th Standard Science Guide Pdf of Text Book Back Questions and Answers Term 1, 2, 3, Chapter Wise Important Questions, Study Material, Question Bank, Notes, drop a comment below and we will get back to you as soon as possible.

Samacheer Kalvi 9th Maths Guide Chapter 1 Set Language Ex 1.4

May 23, 2024

Students can download Maths Chapter 1 Set Language Ex 1.4 Questions and Answers, Notes, Samacheer Kalvi 9th Maths Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus, helps students complete homework assignments and to score high marks in board exams.

Tamilnadu Samacheer Kalvi 9th Maths Solutions Chapter 1 Set Language Ex 1.4

Question 1.
If P = {1, 2, 5, 7, 9}, Q = {2, 3, 5, 9, 11}, R = {3, 4, 5, 7, 9} and S = {2, 3, 4, 5, 8} then find
(i) (P∪Q)∪R
(ii) (P∩Q)∩S
(m) (Q∩S)∩R
Solution:
P = {1, 2, 5, 7, 9}; Q = {2, 3, 5, 9, 11}; R = {3, 4, 5, 7, 9} and S = {2, 3, 4, 5, 8}
(i) P∪Q = {1, 2, 5, 7, 9} ∪ {2, 3, 5, 9, 11}
= {1, 2, 3, 5, 7, 9, 11}
(P∪Q)∪R = {1, 2, 3, 5, 7, 9, 11} ∪ {3, 4, 5, 7, 9}
= {1, 2, 3, 4, 5, 7, 9, 11}

(ii) P∩Q = {1, 2, 5, 7, 9} ∩ {2, 3, 5, 9, 11}
= {2, 5, 9}
(P∩Q)∩S = {2, 5, 9} ∩ {2, 3, 4, 5, 8}
= (2, 5}

(iii) Q∩S = {2, 3, 5, 9, 11} ∩ {2, 3, 4, 5, 8}
= {2, 3, 5}
(Q∩S)∩R = {2, 3, 5} ∩ {3, 4, 5, 7, 9}
= {3, 5}

Question 2.
Test for the commutative property of union and intersection of the sets
P = {x : x is a real number between 2 and 7} and
Q = {x : x is an irrational number between 2 and 7}
Solution:
P is a real number set
Q is a set of irrational number
∴ Q⊂P
P∪Q= Q∪P = P
∴ Union of sets is commutative.
P∩Q = Q∩P = Q
∴ Intersection of sets is commutative.

Question 3.
If A = {p, q, r, s}, B = {m, n, q, s, t} and C = {m, n, p, q, s}, then verify the associative property of union of sets.
Solution:
When union of sets is associative
A∪(B∪C) = (A∪B)∪C
(B∪C) = {m, n, q, s, t) ∪ {m, n, p, q, s}
= {m, n, p, q, s, t}
A∪(B∪C) = {p, q, r, s} ∪ {m, n, p, q, s, t}
= {m, n, p, q, r, s, t} ……..(1)
(A∪B) = {p, q, r, s} ∪ {m, n, q, s, t}
= {m, n, p, q, r, s, t}
(A∪B)∪C = {m, n, p, q, r, s, t} ∪ {m, n, p, q, s}
= {m, n, p, q, r, s, t} ……….(2)
From (1) and (2) it is verified that A∪(B∪C) = (A∪B)∪C

Question 4.
Verify the associative property of intersection of sets for A = {-11, √2, √5, 7},
B = {√3, √5, 6, 13} and C = {√2, √3, √5, 9}.
Solution:
When intersection of sets is associative
A∩(B∩C) = (A∩B)∩C
(B∩C) = {√3, √5, 6, 13} ∩ {√2, √3, √5, 9}
= {√3, √5}
A∩(B∩C) = {-11, √2, √5, 7} ∩ {√3, √5}
{√5} ………(i)
(A∩B) = {-11, √2, √5, 7} ∩ {√3, √5 ,6, 13}
= {√5}
(A∩B)∩C = {√5} n {√2, √3, √5, 9}
= {√5}……..(2)
From (1) and (2) it is verified that A∩(B∩C) = (A∩B)∩C

Question 5.
If A={ x : x = 2ⁿ, n ∈ W and n < 4}, B = {x : x = 2n, n ∈ N and n ≤ 4} and C = {0, 1, 2, 5, 6}, then verify the associative property of intersection of sets.
Solution:
A = {x : x = 2ⁿ, n ∈ W and n < 4}
A = {1, 2, 4, 8}
B = {x : x = 2n, n ∈ N and n ≤ 4}
B = {2, 4, 6, 8}
C ={0, 1, 2, 5, 6}
When intersection of sets is associative
A∩(B∩C) = (A∩B)∩C
(B∩C) = {2, 4, 6, 8} ∩ {0, 1, 2, 5, 6}
= {2, 6}
A∩(B∩C)= {1 ,2, 4, 8} ∩ {2, 6}
= {2}……….(1)
(A∩B) = {1, 2, 4, 8} ∩ {2, 4, 6, 8}
= {2, 4, 8}
(A∩B)∩c= {2, 4, 8} n {0, 1, 2, 5, 6}
= {2}………..(2)
From (1) and (2) we get A∩(B∩C) = (A∩B)∩C

శ్రీ సాయి కాకడ ఆరతి

Samacheer Kalvi 9th Maths Guide Chapter 9 Probability Additional Questions

October 27, 2023

Students can download Maths Chapter 9 Probability Additional Questions and Answers, Notes, Samacheer Kalvi 9th Maths Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus, helps students complete homework assignments and to score high marks in board exams.

Tamilnadu Samacheer Kalvi 9th Maths Solutions Chapter 9 Probability Additional Questions

I. Choose the Correct Answer

Question 1.
Which of the following cannot be taken as probability of an event?
(a) 0
(b) 0.35
(c) \(\frac{7}{20}\)
(d) –\(\frac{7}{20}\)
Solution:
(d) –\(\frac{7}{20}\)

Question 2.
A letter is chosen at random from the word “MATHEMATICS” the probability of getting a vowel is ……..
(a) \(\frac{2}{11}\)
(b) \(\frac{3}{11}\)
(c) \(\frac{4}{11}\)
(d) \(\frac{5}{11}\)
Solution:
(c) \(\frac{4}{11}\)

Question 3.
If P(A) =\(\frac{1}{3}\) then P(A)’ is ………
(a) \(\frac{1}{3}\)
(b) \(\frac{2}{3}\)
(c) \(\frac{3}{2}\)
(d) 1
Solution:
(b) \(\frac{2}{3}\)

Question 4.
An integer is chosen from the first twenty natural number, the probability that it is a prime number is ……..
(a) \(\frac{1}{5}\)
(b) \(\frac{2}{5}\)
(c) \(\frac{3}{5}\)
(d) \(\frac{4}{5}\)
Solution:
(b) \(\frac{2}{5}\)

Question 5.
From a well shuffled pack of 52 cards one card is drawn at random. The probability of getting not a king is ………
(a) \(\frac{12}{13}\)
(b) \(\frac{1}{13}\)
(c) \(\frac{4}{13}\)
(d) \(\frac{2}{13}\)
Solution:
(a) \(\frac{12}{13}\)

II. Answer the Following Questions

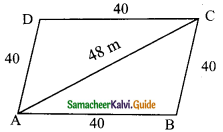
Question 6.
1500 families with 2 children were selected randomly and the following data were recorded.
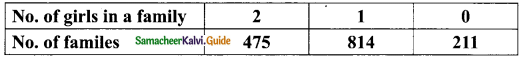
Samacheer Kalvi 9th Maths Guide Chapter 9 Probability Additional Questions 1
Compute the probability of a family chosen at random having one girl.
Solution:
Total number of families = 1500
∴ n(S) = 1500
Let E be the event of getting one girl
n(E) = 814
P(E) = \(\frac{n(E)}{n(S)}\)
= \(\frac{814}{1500}\)
= \(\frac{407}{750}\)

Question 7.
The record of weather station shows that out of the part 250 consecutive days its whether forecast were correct 175 time. What is the probability that it was not correct on a given data?
Solution:
n(S) = 250
Let E be the event of getting whether forecast were correct
n(E) = 175
P(E) = \(\frac{n(E)}{n(S)}\)
= \(\frac{175}{250}\)
= \(\frac{7}{10}\)
Forecast was not correct on a given day = 1 – P(E)
= 1 – \(\frac{7}{10}\)
= \(\frac{10-7}{10}\)
= \(\frac{3}{10}\)

Question 8.
If A coin is tossed 200 times and is found that a tail comes up for 120 times. Find the probability of getting a tail.
Solution:
Number of trials = 200
n(S) = 200
Let E be the event of getting a tail
n(E) = 120
p(E) = \(\frac{n(E)}{n(S)}\)
= \(\frac{120}{200}\)
= \(\frac{12}{20}\)
= \(\frac{3}{5}\)

Question 9.
A bag contains 5 red balls and some blue balls. If the probability of drawing a blue ball from the bag is thrice that of drawing a red ball, then find the number of blue balls in the bag.
Solution:
Let the number of blue balls be “x”
Total number of balls = 5 + x
∴ n(S) = 5 + x
Let B be the event of drawing a blue ball and R be the event of drawing a red ball
Given P(B) = 3P(R)
Samacheer Kalvi 9th Maths Guide Chapter 9 Probability Additional Questions 2
∴ x = 15
Number of blue balls = 15

Question 10.
Find the probability that a non leap year selected at random will have 53 fridays.
Solution:
No. of days in a non leap year = 365 days
This year contain 52 weeks and one day
Sample space = {Sun, Mon, Tue, Wed, Thu, Fri, Sat}
n(S) = 7
Let A be the event of getting a friday
n(A) = 1
P(A) = \(\frac{n(A)}{n(S)}\)
= \(\frac{1}{7}\)

Samacheer Kalvi 9th Maths Guide Chapter 7 Mensuration Additional Questions

October 25, 2023

Students can download Maths Chapter 7 Mensuration Additional Questions and Answers, Notes, Samacheer Kalvi 9th Maths Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus, helps students complete homework assignments and to score high marks in board exams.

Tamilnadu Samacheer Kalvi 9th Maths Solutions Chapter 7 Mensuration Additional Questions

I. Choose the Correct Answer

Question 1.
If the sides of a triangles are 5 cm, 6 cm and 7 cm then the area is ……..
(a) 18 cm²
(b) 6 √2 cm²
(c) 6 √6 cm²
(d) 6 √3 cm²
Solution:
(c) 6 √6 cm²

Question 2.
The perimeter of an equilateral triangle is 60 cm then the area is ………
(a) 60 √3 cm²
(b) 20 √3 cm²
(c) 50 √3 cm²
(d) 100 √3 cm²
Solution:
(d) 100 √3 cm²

Question 3.
The total surface area of the cuboid with dimension 20 cm × 30 cm × 15 cm is ………
(a) 2700 cm²
(b) 1500 cm²
(c) 2500 cm²
(d) 3000 cm²
Solution:
(a) 2700 cm²

Question 4.
The number of bricks each measuring 70 cm × 80 cm × 40 cm that will be required to build a wall whose dimensions are 7 m × 8 m × 4 m is ……..
(a) 4000
(b) 3000
(c) 2000
(d) 1000
Solution:
(d) 1000

Question 5.
The volume of a cube is 4913 m² then the length of its side is ……..
(a) 13 m
(b) 17 m
(c) 34 m
(d) 27 m
Solution:
(b) 17 m

II. Answer the Following Questions

Question 6.
A field is in the shape of a trapezium whose parallel sides are 25 m and 10 m. The non parallel sides are 14 m and 13 m. Find the area of the field.
Solution:
The non parallel sides are 13 m and 14 m. Draw BE || AD. Such that BE = 13 m
∴ ABED is a parallelogram
Samacheer Kalvi 9th Maths Guide Chapter 7 Mensuration Additional Questions 1
To find Area of a ΔBCE
a = 13 m, b = 15 m and c = 14 m
s = \(\frac{a+b+c}{2}\)
= \(\frac{13+15+14}{2}\)
= \(\frac{42}{2}\)
= 21 m
s – a = 21 – 13 = 8 m
s – b = 21 – 15 = 6 m
s – c = 21 – 14 = 7 m
Area of a ΔBCE
Samacheer Kalvi 9th Maths Guide Chapter 7 Mensuration Additional Questions 2
= 2² × 3 × 7
= 84 m²
Let the height of the triangle BF be x
Area of the ΔBEC = 84 m²
= \(\frac{1}{2}\) × b × h = 84
= \(\frac{1}{2}\) × 15 × h = 84
x = \(\frac{84×2}{15}\)
= \(\frac{56}{5}\) m
= 11.2 m
Area of parallelogram ABED = base × height sq. units
= 10 × 11.2 m²
= 112 m²
∴ Area of the field = Area of ΔBCE + Area of parallelogram ABED
= 84 m² + 112 m²
= 196 m²
(OR)
Area of the field = Area of the trapezium ABCD
= \(\frac{1}{2}\) h (a + b)
= \(\frac{1}{2}\) × 11.2 (25 + 10)
= \(\frac{1}{2}\) × 11.2 (35)
= 196 m²

Question 7.
Find the area of a quadrilateral ABCD in which AB = 8 cm, BC = 6 cm, CD = 8 cm, DA = 10 cm and AC = 10 cm and ⌊B = 90°.
Solution:
Samacheer Kalvi 9th Maths Guide Chapter 7 Mensuration Additional Questions 3
In ΔABC, ⌊B = 90°
∴ ABC is a right angle triangle
Area of the right ΔABC = \(\frac{1}{2}\) × AB × BC sq.units
= \(\frac{1}{2}\) × 8 × 6 cm²
= 24 cm²
In ΔACD a = 10 cm, b = 8 cm and c = 10 cm
s = \(\frac{a+b+c}{2}\)
= \(\frac{10+8+10}{2}\)
= \(\frac{28}{2}\)
= 14 cm
s – a = 14 – 10 = 4 cm
s – b = 14 – 8 = 6 cm
s – c = 14 – 10 = 4 cm
Area of ΔACD = \(\sqrt{s(s-a)(s-b)(s-c)}\)
= \(\sqrt{14×4×6×4}\)
= \(\sqrt{2×7×4×2×3×4}\)
= 4 × 2 \(\sqrt{21}\) cm²
= 8\(\sqrt{21}\) cm²
= 8 × 4.58
= 36.64 cm²
Area of the quadrilateral ABCD
= Area of ΔABC + Area of ΔACD
= 24 cm² + 36.64 cm²
= 60.64 cm²
Area of the quadrilateral = 60.64 cm²

Question 8.
The length, breadth and height of a room are 5 m, 4 m and 3 m respectively. Find the cost of white washing the walls of the room and the ceiling at the rate of Rs 7.50 per m².
Solution:
Length of the room (l) = 5 m
Breadth of the room (b) = 4 m
Height of the room (h) = 3 m
Area for white washing = Lateral surface area of four walls + Area of the ceiling
= 2(l + b) × h + (l × b)
= 2(5 + 4) × 3 + (5 × 4) m²
= (2 × 9 × 3 + 20) m²
= (54 + 20) m²
= 74 m²
Cost of white washing for one m² = Rs 7.50
Cost of white washing for 74 m² = Rs 74 × 7.50
= Rs 555
The required cost = Rs 555

Question 9.
How many hollow blocks of size 30 cm × 15 cm × 20 cm are needed to construct a wall 60 m in length 0.3 m in breadth and 2 m in height.
Solution:
Length of a wall = 60 m = 6000 cm
Breadth of a wall = 0.3 m = 30 cm
Height of a wall = 2 m = 200 cm
Volume of the wall = l × b × h sq. unit
= 6000 × 30 × 200 cm³
For hollow block
l = 30 cm, b = 15 cm, h = 20 cm
Volume of one hollow block = l × b × h
= 30 × 15 × 20 cm²
Number of hollow blocks required
Samacheer Kalvi 9th Maths Guide Chapter 7 Mensuration Additional Questions 4
= 4000
∴ Number of bricks = 4000

Question 10.
Find the number of cubes of side 3 cm that can be cut from a cuboid of dimensions 10 cm × 9 cm × 6 cm.
Solution:
Side of a cube = 3 cm
Volume of a cube = a³ cm
= 3 × 3 × 3 cm³
Length of the cuboid (l) = 10 cm
Breadth of the cuboid (b) = 9 cm
Height of the cuboid (h) = 6 cm
Volume of the cuboid = l × b × h cu. unit
= 10 × 9 × 6 cm
Number of cubes
Samacheer Kalvi 9th Maths Guide Chapter 7 Mensuration Additional Questions 5
∴ Number of cubes = 20

Samacheer Kalvi 9th Maths Guide Chapter 6 Trigonometry Additional Questions

October 25, 2023

Students can download Maths Chapter 6 Trigonometry Additional Questions and Answers, Notes, Samacheer Kalvi 9th Maths Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus, helps students complete homework assignments and to score high marks in board exams.

Tamilnadu Samacheer Kalvi 9th Maths Solutions Chapter 6 Trigonometry Additional Questions

I. Choose the Correct Answer

Question 1.
The value of cosec² 60 – 1 is equal to ……..
(a) cos² 60
(b) cot² 60
(c) sec² 60
(d) tan² 60
Solution:
(b) cot² 60

Question 2.
The value of cos 60° cos 30° – sin 60° sin 30° is equal is ……..
(a) cosec 90°
(b) tan 90°
(c) sin 30° + cos 30°
(d) cos 90°
Solution:
(d) cos 90°

Question 3.
The value of \(\frac{sin 57°}{cos 33°}\) is …….
(a) cot 63°
(b) tan 27°
(c) 1
(d) 0
Solution:
(c) 1

Question 4.
If 3 cosec 36° = sec 54° then the value of x is ……..
(a) 0
(b) 1
(c) \(\frac{1}{3}\)
(d) \(\frac{3}{4}\)
Solution:
(c) \(\frac{1}{3}\)

Question 5.
If cos A cos 30° = \(\frac{√3}{4}\), then the measures of A is ……..
(a) 90°
(b) 60°
(c) 45°
(d) 30°
Solution:
(b) 60°

II. Answer the Following Question

Question 1.
Given Sec θ = \(\frac{13}{12}\). Calculate all other trigonometric ratios.
Solution:
In the right triangle ABC
Samacheer Kalvi 9th Maths Guide Chapter 6 Trigonometry Additional Questions 1
BC² = AC² – AB²
= 13² – 12²
= 169 – 144
= 25
∴ BC = \(\sqrt{25}\)
= 5

Question 2.
If 3 cot A = 4 check weather \(\frac{1- tan²A}{1+ tan²A}\) = cos² A – sin² A or not?
Solution:
3 cot A = 4
cot A = \(\frac{4}{3}\)
In the right ΔABC
Samacheer Kalvi 9th Maths Guide Chapter 6 Trigonometry Additional Questions 3
AC² = AB² + BC²
= 4² + 3²
= 16 + 9
= 25
= \(\sqrt{25}\)
= 5

Hence \(\frac{1- tan²A}{1+ tan²A}\) = cos² A – sin² A
R.H.S = cos² A – sin² A

L.H.S = R.H.S

Question 3.
Evaluate \(\frac{sin 30° + tan 45° – cosec 60°}{sec 30° + cos 60° + cot 45°}\)
Solution:
sin 30° = \(\frac{1}{2}\); tan 45° = 1; cosec 60° = \(\frac{2}{√3}\); sec 30° = \(\frac{2}{√3}\); cos 60° = \(\frac{1}{2}\); cot 45° = 1
\(\frac{sin 30° + tan 45° – cosec 60°}{sec 30° + cos 60° + cot 45°}\)
Samacheer Kalvi 9th Maths Guide Chapter 6 Trigonometry Additional Questions 6
The value is \(\frac{43-24√3}{11}\)

Question 4.
Find A if sin 20° tan A sec 70° = √3
Solution:
sin 20° . tan A . sec 70° = √3
sin 20° . sec 70° . tan A = √3
sin (90° – 70°). sec 70° . tan A = √3
cos 70° × latex]\frac{1}{cos 70°}[/latex] tan A = √3
tan A = √3
tan A = tan 60°
∴ ∠A = 60°

Question 5.
Find the area of the right triangle with hypotenuse 8 cm and one of the acute angles is 57°
Solution:
In the ΔABC
Samacheer Kalvi 9th Maths Guide Chapter 6 Trigonometry Additional Questions 7
sin C = \(\frac{AB}{AC}\)
Sin 57° = \(\frac{AB}{8}\)
0.8387 = \(\frac{AB}{8}\)
∴ AB = 0.8387 × 8
= 0.71 cm
In the ΔABC
cos C = \(\frac{BC}{AC}\)
cos 57° = \(\frac{BC}{8}\)
0.5446 = \(\frac{BC}{8}\)
BC = 0.5446 × 8
= 4.36
Area of the right ΔABC
= \(\frac{1}{2}\) × AB × BC sq. units
= \(\frac{1}{2}\) × 6.71 × 4.36 cm²
= 14.62 cm²
Area of the Δ = 14.62 cm²

Category: Class 9

Samacheer Kalvi 9th Tamil Book Solutions Guide Pdf Free Download

Samacheer Kalvi 9th English Book Solutions Guide Pdf Free Download

Tamilnadu Samacheer Kalvi 9th Guide Text Book Back Answers Solutions Pdf Free Download

Samacheer Kalvi 9th Social Science Book Solutions Guide Pdf Free Download

Samacheer Kalvi 9th Social Science History Book Solutions

Samacheer Kalvi 9th Social Science Geography Book Answers

Samacheer Kalvi 9th Social Science Guide Civics

Samacheer Kalvi 9th Social Science Guide Economics

9th Social Science Guide Book Back Questions Tamil Medium with Answers

Samacheer Kalvi 9th Maths Book Solutions Guide Pdf Free Download

9th Standard Maths Guide Tamil Medium Pdf Free Download

Samacheer Kalvi 9th Science Book Solutions Guide Pdf Free Download

9th New Science Book Back Questions Tamil Medium with Answers

Tamilnadu Samacheer Kalvi 9th Maths Solutions Chapter 1 Set Language Ex 1.4

Tamilnadu Samacheer Kalvi 9th Maths Solutions Chapter 9 Probability Additional Questions

Tamilnadu Samacheer Kalvi 9th Maths Solutions Chapter 7 Mensuration Additional Questions

Tamilnadu Samacheer Kalvi 9th Maths Solutions Chapter 6 Trigonometry Additional Questions