Samacheer Kalvi 10th Maths Guide Chapter 4 Geometry Additional Questions

Students can download Maths Chapter 4 Geometry Additional Questions and Answers, Notes, Samacheer Kalvi 10th Maths Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus, helps students complete homework assignments and to score high marks in board exams.

Tamilnadu Samacheer Kalvi 10th Maths Solutions Chapter 4 Geometry Additional Questions

I. Multiple choice questions

Question 1.
If a straight line intersects the sides AB and AC of a ∆ABC at D and E respectively and is parallel to BC, then \(\frac { AE }{ AC } \) = …………
(1) \(\frac { AD }{ DB } \)
(2) \(\frac { AD }{ DB } \)
(3) \(\frac { DE }{ BC } \)
(4) \(\frac { AD }{ EC } \)
Answer:
(2) \(\frac { AD }{ DB } \)

Hint:
By BPT theorem,
\(\frac { AE }{ AC } \) = \(\frac { AD }{ AB } \)

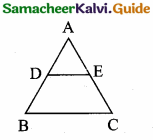
Question 2.
In ∆ABC, DE is || to BC, meeting AB and AC at D and E. If AD = 3 cm, DB = 2 cm and AE = 2.7 cm, then AC is equal to ……..
(1) 6.5 cm
(2) 4.5 cm
(3) 3.5 cm
(4) 3.5 cm
Answer:
(2) 4.5 cm
Hint:
By BPT theorem,

\(\frac { AD }{ DB } \) = \(\frac { AE }{ EC } \)
\(\frac { 3 }{ 2 } \) = \(\frac { 2.7 }{ EC } \)
∴ EC = \(\frac{2.7 \times 2}{3}\) = 1.8 CM
AC = AE + EC
= 2.7 + 1.8 = 4.5 cm

Question 3.
In ∆PQR, RS is the bisector of ∠R. If PQ = 6 cm, QR = 8 cm, RP = 4 cm then PS is equal to …………..
(1) 2 cm
(2) 4 cm
(3) 3 cm
(4) 6 cm
Answer:
(2) 2 cm
Hint:

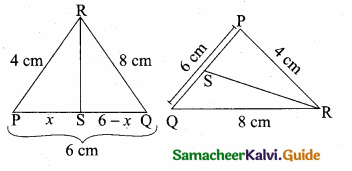
By ABT theorem,
\(\frac { PS }{ SQ } \) = \(\frac { PR }{ QR } \) ⇒ \(\frac { x }{ 6-x } \) = \(\frac { 4 }{ 8 } \)
24 – 4x = 8x ⇒ 24 = 12x
x = 2
PS = 2 cm

Question 4.
In figure, if \(\frac { AB }{ AC } \) = \(\frac { BD }{ DC } \), ∠B = 40° and ∠C = 60°, then ∠BAD = ……………
(1) 30°
(2) 50°
(3) 80°
(4) 40°
Answer:
(4) 40°
Hint:
\(\frac { AB }{ AC } \) = \(\frac { BD }{ DC } \)

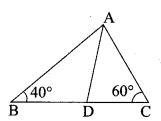
AD is the internal bisector of ∠BAC
∠A + ∠B + ∠C = 180°
∠A + 40° + 60° = 180° ⇒ ∠A = 80°
∴ ∠BAC = \(\frac { 80 }{ 2 } \) = 40°

Question 5.
In the figure, the value x is equal to …………
(1) 4.2
(2) 3.2
(3) 0.8
(4) 0.4

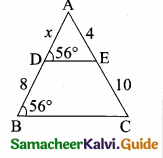
Answer:
(2) 3.2
Hint:
By Thales theorem
(DE || BC)
\(\frac { AD }{ BD } \) = \(\frac { AE }{ EC } \)
\(\frac { x }{ 8 } \) = \(\frac { 4 }{ 10 } \) ⇒ x = \(\frac{8 \times 4}{10}\) = 3.2

Question 6.
In triangles ABC and DEF, ∠B = ∠E, ∠C = ∠F, then ………….
(1) \(\frac { AB }{ DE } \) = \(\frac { CA }{ EF } \)
(2) \(\frac { BC }{ EF } \) = \(\frac { AB }{ FD } \)
(3) \(\frac { AB }{ DE } \) = \(\frac { BC }{ EF } \)
(4) \(\frac { CA }{ FD } \) = \(\frac { AB }{ EF } \)
Answer:
(3) \(\frac { AB }{ DE } \) = \(\frac { BC }{ EF } \)
Hint:

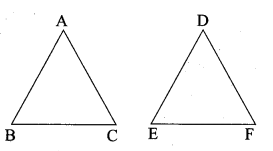
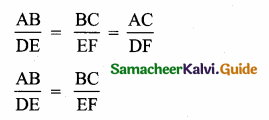
Question 7.
From the given figure, identify the wrong statement.

(1) ∆ADB ~ ∆ABC
(2) ∆ABD ~ ∆ABC
(3) ∆BDC ~ ∆ABC
(4) ∆ADB ~ ∆BDC
Answer:
(2) ∆ ABD ~ ∆ ABC

Question 8.
If a vertical stick 12 m long casts a shadow 8 m long on the ground and at the same time a tower casts a shadow 40 m long on the ground, then the height of the tower is …………..
(1) 40 m
(2) 50 m
(3) 75 m
(4) 60 m
Answer:
(4) 60 m
Hint:

\(\frac { AB }{ DE } \) = \(\frac { BC }{ EF } \)
\(\frac { 12 }{ h } \) = \(\frac { 8 }{ 40 } \); h = \(\frac{40 \times 12}{8}\) = 60

Question 9.
The sides of two similar triangles are in the ratio 2:3, then their areas are in the ratio ………….
(1) 9 : 4
(2) 4 : 9
(3) 2 : 3
(4) 3 : 2
Answer:
(2) 4 : 9
Hint:
Ratio of the Area of two similar triangle
= 2² : 3² = 4 : 9
[squares of their corresponding sides]

Question 10.
Triangles ABC and DEF are similar. If their areas are 100 cm² and 49 cm² respectively and BC is 8.2 cm then EF = …………….
(1) 5.47 cm
(2) 5.74 cm
(3) 6.47 cm
(4) 6.74 cm
Answer:
(2) 5.74 cm
Hint:

Question 11.
The perimeters of two similar triangles are 24 cm and 18 cm respectively.
If one side of the first triangle is 8 cm, then the corresponding side of the other triangle is ………
(1) 4 cm
(2) 3 cm
(3) 9 cm
(4) 6 cm
Answer:
(4) 6 cm
Hint:

Question 12.
A point P is 26 cm away from the centre O of a circle and PT is the tangent drawn from P to the circle 10 cm, then OT is equal to …………
(1) 36 cm
(2) 20 cm
(3) 18 cm
(4) 24 cm
Answer:
(4) 24 cm
Hint:

OT² = OP² – PT²
= 26² – 10²
= (26 + 10) (26 – 10)
OT² = 36 × 16
OT = 6 × 4 = 24 cm

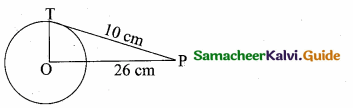
Question 13.
In the figure, if ∠PAB = 120° then ∠BPT = ……….

(1) 120°
(2) 30°
(3) 40°
(4) 60°
Answer:
(4) 60°
Hint:
∠BCP + ∠PAB = 180°
(sum of the opposite angles of a cyclic quadrilateral)
∠BCP = 180° – 120° = 60°
∠BPT = 60°
(By tangent chord theorem)

Question 14.
If the tangents PA and PB from an external point P to circle with centre O are inclined to each other at an angle of 40°, then ∠POA = ………………
(1) 70°
(2) 80°
(3) 50°
(4) 60°
Answer:
(1) 70°
Hint:
∠OPA = \(\frac { 40 }{ 2 } \) = 20°
In ∆OAP.
∠POA + ∠OAP + ∠APO = 180°
(sum of the angles of a triangle)

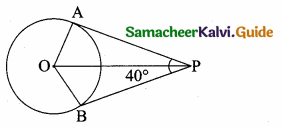
∠POA + 90° + 20° = 180°
∠POA = 180° – 110° = 70°

Question 15.
In the figure, PA and PB are tangents to the circle drawn from an external point P. Also CD is a tangent to the circle at Q. If PA = 8 cm and CQ = 3 cm, then PC is equal to ……….

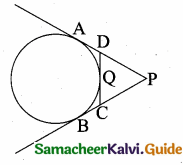
(1) 11 cm
(2) 5 cm
(3) 24 cm
(4) 38 cm
Answer:
(2) 5 cm
Hint:
PA = PB (tangent of a circle)
PB = 8 cm
PC + BC = 8
PA + QC = (BC = QC tangent)
PC + 3 = 8
∴ PC = 8 cm – 3 cm = 5 cm

Question 16.
∆ABC is a right angled triangle where ∠B = 90° and BD ⊥ AC. If BD = 8 cm, AD = 4 cm, then CD is …………
(1) 24 cm
(2) 16 cm
(3) 32 cm
(4) 8 cm
Answer:
(2) 16 cm
Hint:
∆DCB ~ ∆DBA
\(\frac { DC }{ DB } \) = \(\frac { DB }{ DA } \)

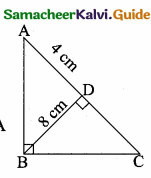
DB² = DC × DA
8² = DC × 4
DC = \(\frac { 64 }{ 4 } \) = 16 cm

Question 17.
The areas of two similar triangles are 16 cm2 and 36 cm2 respectively. If the altitude of the first triangle is 3 cm, then the corresponding altitude of the other triangle is
(1) 6.5 cm
(2) 6 cm
(3) 4 cm
(4) 4.5 cm
Answer:
(4) 4.5 cm
Hint:

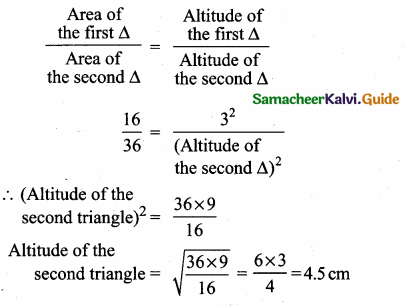
Question 18.
The perimeter of two similar triangles ∆ABC and ∆DEF are 36 cm and 24 cm respectively. If DE =10 cm, then AB is …………
(1) 12 cm
(2) 20 cm
(3) 15 cm
(4) 18 cm
Answer:
(3) 15 cm
Hint:
Perimeter of

Question 19.
In the given diagram θ is ………….
(1) 15°
(2) 30°
(3) 45°
(4) 60°

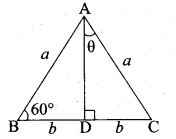
Answer:
(2) 30°
Hint:
∠BAD = 180° – 150° = 30°
= 180° – 150° = 30°
∠DAC = θ = 30°

Question 20.
If AD is the bisector of ∠A then AC is …………

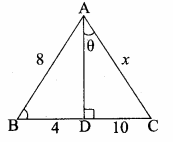
(1) 12
(2) 16
(3) 18
(4) 20
Answer:
(4) 20
Hint. In ∆ABC, AD is the internal bisector of ∠A
\(\frac { BD }{ DC } \) = \(\frac { AB }{ AC } \)
\(\frac { 4 }{ 10 } \) = \(\frac { 8 }{ x } \)
4x = 10 × 8
x = \(\frac{10 \times 8}{4}\) = 20 cm

Question 21.
In ∆ABC and ∆DEF, ∠A = ∠E and ∠B = ∠F. Then AB : AC is ………….
(1) DE : DF
(2) DE : EF
(3) EF : ED
(4) DF : EF
Answer:
(3) EF : ED
Hint:

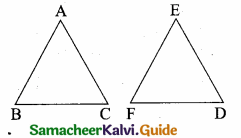
\(\frac { AB }{ EF } \) = \(\frac { BC }{ FD } \) = \(\frac { AC }{ ED } \)

Question 22.
Two circles of radius 8.2 cm and 3.6 cm touch each other externally, the distance between their centres is ………….
(1) 1.8 cm
(2) 4.1 cm
(3) 4.6 cm
(4) 11.8 cm
Answer:
(4) 11.8 cm
Hint:
Distance between the two centres

= r₁ + r₂
= 8.2 + 3.6
= 11.8 cm

Question 23.
In the given diagram PA and PB are tangents drawn from P to a circle with centre O. ∠OPA = 35° then a and b is …………

(1) a = 30°, b = 60°
(2) a = 35°, b = 55°
(3) a = 40°, b = 50°
(4) a = 45°, b = 45°
Answer:
(2) a = 35°, b = 55°
Hint:
∠OAP = 90° (tangent of the circle)
∠AOP + ∠OPA + ∠PAO = 180°
b + 35° + 90° = 180°
b = 180° – 125°
= 55°
OP is the angle bisector of ∠P
∴ a = 35°

II. Answer the following questions

Question 1.
The image of a man of height 1.8 m, is of length 1.5 cm on the film of a camera. If the film is 3 cm from the lens of the camera, how far is the man from the camera?
Solution:
Let AB be the height of the man, CD be the height of the image of the man of height 1.8 m (180 cm). LM be the distance between man and lens. LN be the distance between Lens and the film.

Given, AB = 1.8 m (180 cm)
CD = 1.5 cm and LN = 3 cm
Consider ∆ LAB and ∆ LCD
∠ALB = ∠DLC (vertically opposite angles)
∠LAB = ∠LDC (alternate angles) (AB || CD)
∴ ∆ LAB ~ ∆ LDC (AA similarity)
∴ \(\frac { AB }{ CD } \) = \(\frac { LM }{ LN } \) ⇒ \(\frac { 180 }{ 1.5 } \) = \(\frac { x }{ 3 } \) ⇒ 1.5x = 180 × 3
x = \(\frac{180 \times 3}{1.5}\) = \(\frac{180 \times 3 \times 10}{15}\)
x = 360 cm (or) 3.6 m
∴ The distance between the man and the camera = 3.6 cm

Question 2.
A girl of height 120 cm is walking away from the base of a lamp-post at a speed of 0. 6 m/sec. If the lamp is 3.6 m above the ground level, then find the length of her shadow after 4 seconds.
Solution:
Let AB be the height of the lamp-post above the ground level.
AB = 3.6 m = 360 cm
Let CD be the height of the girl.
CD = 1.2 m = 120 cm
The distance travelled by the girl in 4 seconds (AC)
= 4 × 0.6 = 2.4m = 240 cm
Consider ∆ECD and ∆EAB
Given (CD || AB)
∠EAB = ∠ECD = 90°
∠E is common

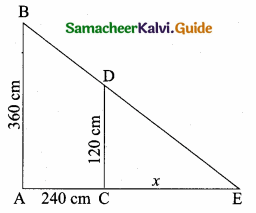
∴ ∆ EAB = ∆ ECD = 90°
\(\frac { AB }{ CD } \) = \(\frac { AE }{ CE } \)
= \(\frac { x+240 }{ x } \) ⇒ 3 = \(\frac { x+240 }{ x } \)
3x = x + 240
2x = 240 ⇒ x = \(\frac { 240 }{ 2 } \) = 120 cm
∴ Lenght of girls shadow after 4 seconds = 120 cm

Question 3.
In ∆ ABC, AB = AC and BC = 6 cm. D is a point on the side AC such that AD = 5 cm and CD = 4 cm. Show that ∆BCD ~ ∆ACB and hence find BD.
Solution:
Given, In ∆ ABC, AB = AC = 9 cm and BC = 6 cm, AD = 5 cm and CD = 4 cm
\(\frac { BC }{ AC } \) = \(\frac { 6 }{ 9 } \) = \(\frac { 2 }{ 3 } \) …..(1)
\(\frac { CD }{ CB } \) = \(\frac { 4 }{ 6 } \) = \(\frac { 2 }{ 3 } \) ……..(2)

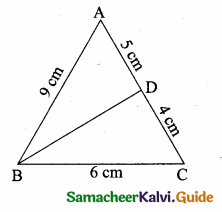
From (1) and (2) we get,
\(\frac { BC }{ AC } \) = \(\frac { CD }{ CB } \)
In ∆ BCD and ∆ ACB
∠C = ∠C (common angle)
\(\frac { BC }{ AC } \) = \(\frac { CD }{ CB } \)
∴ ∆ BCD ~ ∆ ACB
\(\frac { BD }{ AB } \) = \(\frac { BC }{ AC } \) ⇒ \(\frac { BD }{ 9 } \) = \(\frac { 6 }{ 9 } \) ⇒ ∴ BD = 6 cm

Question 4.
The lengths of three sides of a triangle ABC are 6 cm, 4 cm and 9 cm. ∆PQR ~ ∆ABC. One of the lengths of sides of APQR is 35 cm. What is the greatest perimeter possible for ∆PQR?
Solution:
Given, ∆ PQR ~ ∆ ABC

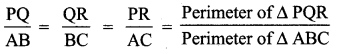
Perimeter of ∆ ABC = 6 + 4 + 9 = 19 cm
When the perimeter of ∆ PQR is the greatest only the corresponding side QR must be equal to 35 cm

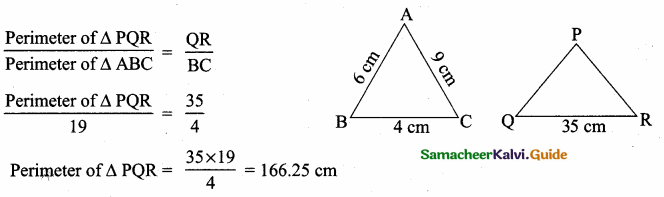
Question 5.
A man sees the top of a tower in a mirror which is at a distance of 87.6 m from the tower. The mirror is on the ground, facing upward. The man is 0.4 m away from the mirror, and the distance of his eye level from the ground is 1.5 m. How tall is the tower? (The foot of man, the mirror and the foot of the tower lie along a straight line).
Solution:
Let AB and ED be the heights of the man and the tower respectively. Let C be the point of incidence of the tower in the mirror.
In ∆ ABC and ∆ EDC, we have

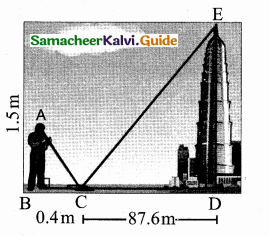
∠ABC = ∠EDC = 90°
∠BCA = ∠DCE
(angular elevation is same at the same instant, i.e., the angle of incidence and the angle of reflection are same.)
∴ ∆ ABC ~ ∆ EDC (AA similarity criterion)
Thus,
\(\frac { ED }{ AB } \) = \(\frac { DC }{ BC } \) (corresponding sides are proportional)
ED = \(\frac { DC }{ BC } \) × AB = \(\frac { 87.6 }{ 0.4 } \) × 1.5 = 328.5
Hence, the height of the tower is 328.5 m.

Question 6.
In ∆ PQR, given that S is a point on PQ such that ST || QR and \(\frac { PS }{ SQ } \) = \(\frac { 3 }{ 5 } \). If PR = 5.6 cm, then find PT.
Solution:
In ∆ PQR, we have ST || QR and by Thales theorem,
\(\frac { PS }{ SQ } \) = \(\frac { PT }{ TR } \) …….(1)
Let PT = x
Thus, TR = PR – PT = 5.6 – x

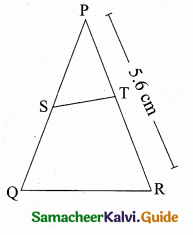
From (1), we get PT = TR (\(\frac { PS }{ SQ } \))
x = (5.6 – x) (\(\frac { 3 }{ 5 } \))
5x = 16.8 – 3x
8x = 16.8
x = \(\frac { 16.8 }{ 8 } \) = 2.1
That is PT = 2.1 cm

Question 7.
In a ∆ ABC, D and E are points on the sides AB and AC respectively such that DE || BC. If AD = 4x – 3, BD = 3x – 1, AE = 8x – 7 and EC = 5x – 3, then find the value of x.
Solution:
In ∆ ABC, DE || BC. By BPT theorem. (Thales theorem)
We get \(\frac { AD }{ DB } \) = \(\frac { AE }{ EC } \) ⇒ \(\frac { 4x-3 }{ 3x-1 } \) = \(\frac { 8x-7 }{ 5x-3 } \)

(8x – 7) (3x – 1) = (4x – 3) (5x – 3)
24x² – 8x – 21x + 7 = 20x² – 12x – 15x + 9
24x² – 8x – 21x + 7 = 20x² – 27x + 9
24x² – 29x + 7 – 20x² + 27x – 9 = 0
∴ 4x² – 2x – 2 = 0
÷ 2 ⇒ 2x² – x – 1 = 0
(x – 1) (2x + 1) = 0

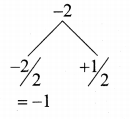
x – 1 = 0 (or) 2x + 1 = 0
x = 1 (or) 2x = – 1
x = – \(\frac { 1 }{ 2 } \) ⇒ since, x ≠ – \(\frac { 1 }{ 2 } \)
∴ The value of x = 1

Question 8.
In the figure AC || BD and CE || DF. if OA = 12 cm, AB = 9cm, OC = 8 cm and EF = 4.5 cm, then find FO.
Solution:

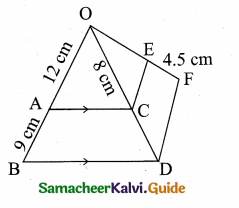
In OBD, AC || BD
∴ \(\frac { OA }{ AB } \) = \(\frac { OC }{ CD } \) (By Thales theorem)
\(\frac { 12 }{ 9 } \) = \(\frac { 8 }{ CD } \)
∴ CD = \(\frac{9 \times 8}{12}\) = 6 CM
In ODF, CE || DF
\(\frac { OC }{ CD } \) = \(\frac { OE }{ EF } \) (By Thales theorem)
\(\frac { 8 }{ 6 } \) = \(\frac { OE }{ 4.5 } \) ⇒ OE = \(=\frac{8 \times 4.5}{6}\) = 6 cm
FO = FE + EO = 4.5 + 6 = 10.5 cm
∴ The value of FO = 10.5 cm

Question 9.
Check whether AD is the bisector of ∠A of ∆ABC in each of the following.
(i) AB = 4 cm, AC = 6 cm, BD 1.6 cm, and CD = 2.4 cm.
Solution:
\(\frac { BD }{ DC } \) = \(\frac { 1.6 }{ 2.4 } \) = \(\frac { 16 }{ 24 } \) = \(\frac { 2 }{ 3 } \) …..(1)
\(\frac { AB }{ AC } \) = \(\frac { 4 }{ 6 } \) = \(\frac { 2 }{ 3 } \) ……..(2)
From (1) and (2) we get,

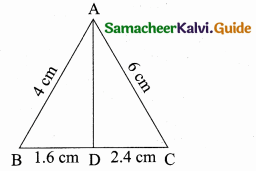
\(\frac { BD }{ DC } \) = \(\frac { AB }{ AC } \)
By the converse of angle bisector theorem we have,
∴ AD is the internal bisector of ∠A

(ii) AB = 6 cm, AC = 8 cm, BD = 1.5 cm and CD = 3 cm
Solution:
\(\frac { BD }{ DC } \) = \(\frac { 1.5 }{ 3 } \) = 0.5 …….(1)
\(\frac { AB }{ AC } \) = \(\frac { 6 }{ 8 } \) = \(\frac { 3 }{ 4 } \) …….(2)

From (1) and (2) we get,
\(\frac { BD }{ DC } \) ≠ \(\frac { AB }{ AC } \)
Hence AD is not the bisector of ∠A.

Question 10.
In a ∆ABC, AD is the internal bisector of ∠A, meeting BC at D. If AB 5.6 cm, AC = 6 cm and DC = 3 cm, find BC.
Solution:
Given, AB = 5.6 cm, AC = 6 cm, DC = 3 cm
Let BD be x
In ∆ ABC, AD is the internal bisector of ∠A.
By Angle bisector theorem, we have,

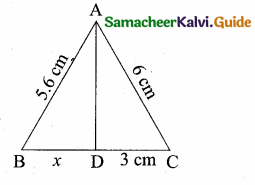
\(\frac { BD }{ DC } \) = \(\frac { AB }{ AC } \) ⇒ \(\frac { x }{ 3 } \) = \(\frac { 5.6 }{ 6 } \)
x = \(\frac{3 \times 5.6}{6}\) = 2.8 cm
∴ BC = BD + DC = 2.8 + 3 = 5.8 cm
Length of BC = 5.8 cm

Question 11.
In the figure, tangents PA and PB are drawn to a circle with centre O from an external point P. If CD is a tangent to the circle at E and AP = 15 cm, find the perimeter of ∆PCD.
Solution:
We know that the lengths of the two tangents from an exterior point to a circle are equal.
∴ CA = CE, DB = DE and PA = PB
Now, the perimeter of ∆PCD

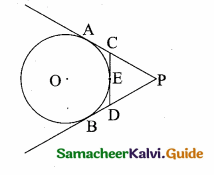
= PC + CD + DP
= PC + CE + ED + DP
= PC + CA + DB + DP
= PA + PB = 2 PA (PB = PA)
Thus, the perimeter of APCD = 2 × 15 = 30 cm

Question 12.
ABCD is a quadrilateral such that all of its sides touch a circle. If AB = 6 cm, BC = 6.5 cm and CD = 7 cm, then find the length of AD.
Solution:
Let P, Q, R and S be the points where the circle touches the quadrilateral.
We know that the lengths of the two tangents drawn from an exterior point to a circle are equal. Thus, we have,

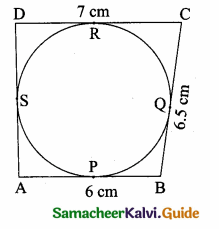
AP = AS, BP = BQ, CR = CQ and DR = DS
Hence, AP + BP + CR + DR = AS + BQ + CQ + DS
⇒ AB + CD = AD + BC
⇒ AD = AB + CD – BC
= 6 + 7 – 6.5 = 6.5
Thus, AD = 6.5 cm

Question 13.
A man goes 10 m due east and then 24 m due north. Find the distance from the starting point.
Solution:
Let the initial position of the man be “O” and the final position be B.
In the ∆AOB,

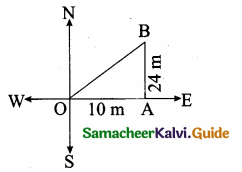
OB² = OA² + AB²
OB² = 10² + 24²
= 100 + 576 = 676
OB = \(\sqrt { 676 }\) = 26 m
The man is at a distance of 26 m from the starting point.

Question 14.
Suppose AB, AC and BC have lengths 13, 16 and 20 respectively. If \(\frac { AF }{ FB } \) = \(\frac { 4 }{ 5 } \) and \(\frac { CE }{ EA } \) = \(\frac { 5 }{ 12 } \) Find BD and DC.
Solution:
Given that AB = 13, AC = 16 and BC = 20. Let BD = JC and DC = y.
Using Ceva’s theorem we have

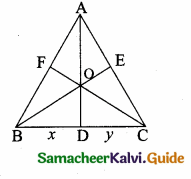
\(\frac { BD }{ DC } \) × \(\frac { CE }{ EA } \) × \(\frac { AF }{ FB } \) = 1 ………(1)
Substitute the given values
\(\frac { x }{ y } \) × \(\frac { 5 }{ 12 } \) × \(\frac { 4 }{ 5 } \) = 1 ⇒ \(\frac { x }{ y } \) × \(\frac { 1 }{ 3 } \) = 1
\(\frac { x }{ y } \) = 3 ⇒ x = 3y
x – 3y = 0 ……(2)
Given BC = 20
x + y = 20 …..(3)
Subtract (2) and (3)

Question 15.
ABC is a right – angled triangle at B. Let D and E be any two points on AB and BC respectively. prove that AE² + CD² = AC² + DE².
Solution:
In the right ∆ ABE right- angled at B.
AE² = AB² + BE² …..(1)
In the right ∆ DBC,CD² = BD² + BC² ………(2)
Adding (1) and (2) we get
AE² + CD² = AB² + BE² + BD² + BC²
= (AB² + BC²) + (BC² + BD²)
AE² + CD² = AC² + DE²
Hence it is proved

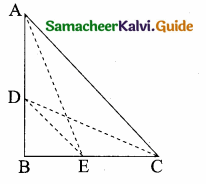
[AC² = AB² + BC²]
[DE² = BE² + BD²]

III. Answer the following questions

Question 1.
A point O in the interior of a rectangle ABCD is joined to each of the vertices A, B, C and D. Prove that OA² + OC² = OB² + OD².
Solution:
Given: O is any point inside the rectangle ABCD.
To prove: OA² + OC² = OB² + OD²
Construction: Through “O” draw EF || AB.
Proof: Using Pythagoras theorem

In the right ∆OEA,
∴ OA²= OE² + AE² …(1)
(By Pythagoras theorem)
In the right ∆OFC,
OC² = OF² + FC² …(2)
(By Pythagoras theorem)
OB² = OF² + FB² … (3)
(By Pythagoras theorem)
In the right ∆OED,
OD² = OE² + ED² … (4)
(By Pythagoras theorem)
By adding (3) and (4) we get
OB² + OD² = OF² + FB² + OE² + ED²
= (OE² + FB²) + (OF² + ED²)
= (OE² + EA²) + (OF² + FC²)
[FB = EA and ED = FC]
OB² + OD² = OA² + OC² using (1) and (2)

Question 2.
A lotus is 20 cm above the water surface in a pond and its stem is partly below the water surface. As the wind blew, the stem is pushed aside so that the lotus touched the water 40 cm away from the original position of the stem. How much of the stem was below the water surface originally?
Solution:
Let O be the bottom of the stem immersed in water.
Let B be the lotus, AB be the length of the stem above the water surface
AB = 20 cm
Let OA be the length of the stem below the water surface
Let OA = x cm
Let C be the point where the lotus touches the water surface when the wind blow.
OC = OA + AB
OC = x + 20 cm
In ∆ AOC, OC² = OA² + AC²
(x + 20)² = x² + 40²
x² + 400 + 40x = x² + 1600

40x = 1600 – 400
40x = 1200
x = \(\frac { 1200 }{ 40 } \) = 30 cm
The stem is 30 cm below the water surface.

Question 3.
In the figure, DE || BC and \(\frac { AD }{ BD } \) = \(\frac { 3 }{ 5 } \), calculate the value of

Solution:
(i) Given, DE || BC and \(\frac { AD }{ BD } \) = \(\frac { 3 }{ 5 } \)
Let AD = 3k and BD = 5k; AB = 3k + 5k = 8k
In ∆^le ABC and ∆ADE
∠A = ∠A (common angle)
∠ABC = ∠ADE (corresponding angle)
Since DE || BC
∴ ∆ ABC ~ ∆ ADE

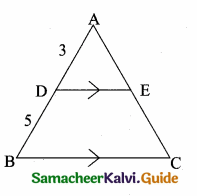
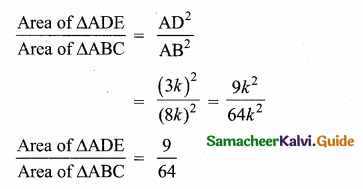
(ii) Let Area of ∆ ADE be 9k and Area of ∆ ABC be 64 k
Area of ∆ BCED = Area of ∆ ABC – Area of ∆ ADE
= 64 k – 9 k
= 55 k

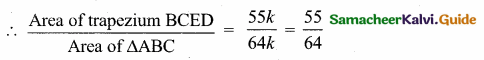
Question 4.
A boy is designing a diamond shaped kite, as shown in the figure where AE = 16 cm, EC = 81 cm. He wants to use a straight cross bar BD. How long should it be?
Solution:
Let in AB be “x”, BE be “z” and BC be “y”
In the right ∆ AEB,
AB² = AE² + BE²
x² = 16² + Z² …….(1)
In the right ∆ BEC,
BC² = EC² + BE²
y² = 81² + z² ……(2)

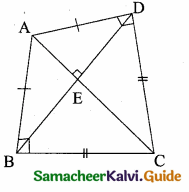
In the right ∆ ACD,
AC² = AD² + DC²
97² = x² + y² ….(3)
Add (1) and (2) ⇒ x² + y² = 162 + z² + 81² + z²
x² + y² = 2z² + 16² + 81²
97² = 2z² + 16² + 81² (from 3)
9409 = 2z² + 256 + 6561
= 2z² + 6817
2z² = 9409 – 6817 = 2592
z² = \(\frac { 2592 }{ 2 } \) = 1296
z = \(\sqrt { 1296 }\) = 36
∴ Length of cross bar BD = 2 × BE = 2 × 36 = 72 cm

Question 5.
Find the unknown values in each of the following figures. All lengths are given in centimetres (All measures are not in scale)

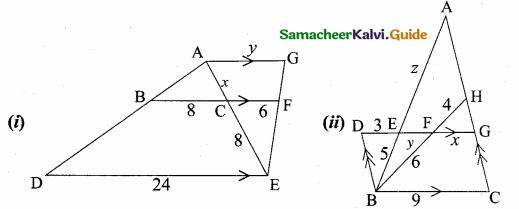
Solution:
(i) In ∆ ABC and ∆ ADE,
∠A = ∠A (common angle)
∠ABC = ∠ADE (corresponding angle) [BC || DE]
∆ ABC ~ ∆ ADE (By AAA similarity)

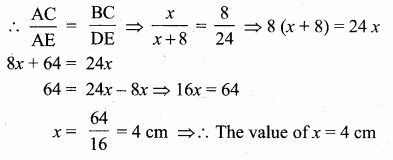
In ∆ EAG and ∆ ECF,
∠E || ∠E (common angle)
∠ECF = ∠EAG (corresponding angle)
Given CF || AG
∆ EAG ~ ∆ ECF
\(\frac { EC }{ EA } \) = \(\frac { CF }{ AG } \) ⇒ \(\frac { 8 }{ x+8 } \) = \(\frac { 6 }{ y } \) ⇒ \(\frac { 8 }{ 12 } \) = \(\frac { 6 }{ y } \) (x = 4)
8y = 6 × 12
y = \(\frac{12 \times 6}{8}\) = 9 cm
∴ The value of x = 4 cm and y = 9 cm.

(ii) In ∆ HBC and ∆ HFG,
∠H = ∠H (common angle)
∠HFG = ∠HBC (corresponding angle)
Given FG || BC
\(\frac { HF }{ HB } \) = \(\frac { FG }{ BC } \) ⇒ \(\frac { 4 }{ 10 } \) = \(\frac { x }{ 9 } \) ⇒ 10x = 36
x = 3.6 cm
In ∆ FBD and ∆ FHD,
∠BFD = ∠HFG (vertically opposite angle)
∠FBD = ∠FHG (Alternate angles)
By AA similarity
∆ FBD ~ ∆ FHG
\(\frac { FG }{ FD } \) = \(\frac { FH }{ FB } \) ⇒ \(\frac { x }{ 3+y } \) = \(\frac { 4 }{ 6 } \)
4 (3 + y) = 3.6 × 6
3 + y = \(\frac{3.6 \times 6}{4}\) = 5.4 ⇒ y = 5.4 – 3 = 2.4 cm
In ∆ AEG and ∆ ABC,
∠A = ∠A (common angle)
∠AEG = ∠ABC (corresponding angles)
Given EG || BC
\(\frac { AE }{ AB } \) = \(\frac { EG }{ BC } \)
\(\frac { z }{ z+5 } \) = \(\frac { x+y }{ 9 } \) ⇒ \(\frac { z }{ z+5 } \) = \(\frac { 3.6+2.4 }{ 9 } \)
\(\frac { z }{ z+5 } \) = \(\frac { 6 }{ 9 } \) ⇒ \(\frac { z }{ z+5 } \) = \(\frac { 2 }{ 3 } \)
3z = 2z + 10 ⇒ 3z – 2z = 10 ⇒ z = 10
∴ The values of x = 3.6 cm, y = 2.4 cm and z = 10 cm.

Question 6.
The internal bisector of ∠A of AABC meets BC at D and the external bisector of ∠A meets BC produced at E. Prove that \(\frac { BD }{ BE } \) = \(\frac { CD}{ CE } \)
Solution:
Given: In ∆ ABC, AD is the internal bisector of ∠A meets BC at D. AE is the external bisector of ∠A meets BC produced to E.
To Proof: \(\frac { BD }{ BE } \) = \(\frac { CD }{ CE } \)

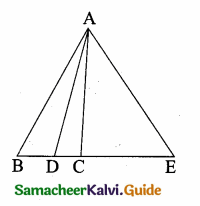
Proof: In ∆ ABC, AD is the internal bisector of ∠A.
By ABT theorem we have,
∴ \(\frac { BE }{ CD } \) = \(\frac { AB }{ AC } \) ……….(1)
In ∆ABC, AE is the external bisector of ∠A.
By ABT theorem we have,
∴ \(\frac { BE }{ CE } \) = \(\frac { AB }{ AC } \) ……….(2)
From (1) and (2) we get
\(\frac { BD }{ CD } \) = \(\frac { BE }{ CE } \) ⇒ ∴ \(\frac { BD }{ BE } \) = \(\frac { CD }{ CE } \)

Question 7.
In a quadrilateral ABCD, the bisectors of ∠B and ∠D intersect on AC at E. Prove that \(\frac { AB }{ BC } \) = \(\frac { AD }{ DC } \)
Solution:
Given: ABCD is a quadrilateral. BE is the bisector of ∠B intersecting AC at E, DE is the bisector of ∠D intersecting AC at E.
To proove: \(\frac { AB }{ BC } \) = \(\frac { AD }{ DC } \)
Proof: In ∆ABC, BE is the internal bisector of ∠D.
By Angle bisector theorem we have,
\(\frac { AE }{ EC } \) = \(\frac { AB }{ BC } \) ……..(1)

In ∆ ACD, DE is the internal bisector of ∠C.
By Angle bisector theorem we have,
∴ \(\frac { AE }{ EC } \) = \(\frac { AD }{ DC } \) ……….(2)
From (1) and (2) we get,
\(\frac { AB }{ BC } \) = \(\frac { AD }{ DC } \)

Question 8.
ABCD is a quadrilateral with AB parallel to DC. A line drawn parallel to AB meets AD at P and BC at Q. Prove that \(\frac { AP }{ PD } \) = \(\frac { BQ }{ QC } \)
Solution:
Given: ABCD is a quadrilateral. AB || DC.
The line PQ intersect AD at P and BC at Q

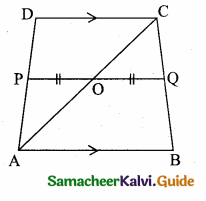
To prove: \(\frac { AP }{ PD } \) = \(\frac { BQ }{ QC } \)
Proof: In the ∆ABC, OQ || AB
By BPT, theorem we have, \(\frac { AO }{ OC } \) = \(\frac { BQ}{ QC } \) ……………(1)
In the ∆ACD, PO || DC
By BPT, theorem we have, \(\frac { AO }{ OC } \) = \(\frac { AP }{ PD } \) ……..(2)
From (1) and (2) we get, \(\frac { AP }{ PD } \) = \(\frac { BQ }{ QC } \)

Question 9.
D is the midpoint of the side BC of AABC. If P and Q are points on AB and on AC such that DP bisects ∠BDA and DQ bisects ∠ADC, then prove that PQ || BC.
Solution:
In ∆ABD,DP is the angle bisector of ∠BDA.
∴ \(\frac { AP }{ PB } \) = \(\frac { AD }{ BD } \) (angle bisector theorem) ……..(1)
In ∆ADC, DQ is the bisector of ∠ADC
∴ \(\frac { AQ }{ QC } \) = \(\frac { AD }{ DC } \) (angle bisector theorem) ……….(2)

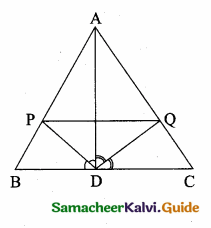
But, BD = DC (D is the midpoint of BC)
Now (2) ⇒ ∴ \(\frac { AQ }{ QC } \) = \(\frac { AD }{ BD } \)
From (1) and (3) We get,
∴ \(\frac { AP }{ PB } \) = \(\frac { AQ }{ QC } \)
Thus PQ || BC (converse of thales theorem)

Question 10.
ABCD is a trapezium with AB || DC. The diagonal AC and BD intersect at E. If ∆AED ~ ∆BEC. Prove that AD = BC.
Solution:
By given data ABCD is a trapezium with AB || DC.
In ∆ ECD and ∆ ABE

∠EDC = ∠EBA
∠ECD = ∠EAB
∴ ∆ DEC ~ ∆ BEA by AA – similarity
\(\frac { DE }{ BE } \) = \(\frac { EC }{ EA } \) = \(\frac { DC }{ BA } \)
\(\frac { DE }{ BE } \) = \(\frac { EC }{ EA } \)
\(\frac { DE }{ EC } \) = \(\frac { BE }{ EA } \) ……….(1)

Also given ∆ DEA ~ ∆ CEB
\(\frac { DE }{ CE } \) = \(\frac { EA }{ EB } \) = \(\frac { DA }{ CB } \)
\(\frac { DE }{ CE } \) = \(\frac { EA }{ EB } \) …………(2)
From (1) and (2) we get
\(\frac { BE }{ EA } \) = \(\frac { EA }{ EB } \) ⇒ EB² = EA²
∴ EB = EA
Substitute in (2) we get
\(\frac { EA }{ EA } \) = \(\frac { DA }{ CB } \)
1 = \(\frac { DA }{ CB } \) ⇒ ∴ AD = DC Hence it is proved