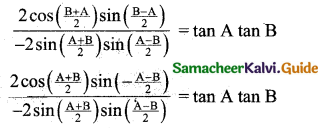Students can download 11th Business Maths Chapter 4 Trigonometry Ex 4.3 Questions and Answers, Notes, Samcheer Kalvi 11th Business Maths Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus, helps students complete homework assignments and to score high marks in board exams.
Tamilnadu Samacheer Kalvi 11th Business Maths Solutions Chapter 4 Trigonometry Ex 4.3
Samacheer Kalvi 11th Business Maths Trigonometry Ex 4.3 Text Book Back Questions and Answers
Question 1.
Express each of the following as the sum or difference of sine or cosine:
(i) sin\(\frac{A}{8}\) sin\(\frac{3A}{8}\)
(ii) cos(60° + A) sin(120° + A)
(iii) cos\(\frac{7 A}{3}\) sin\(\frac{5 A}{3}\)
(iv) cos 7θ sin 3θ
Solution:
(i) sin\(\frac{A}{8}\) sin\(\frac{3A}{8}\)
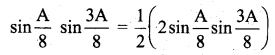
[∵ 2 sin A sin B = cos(A – B) – cos(A + B)

[∵ cos(-θ) = cos θ]
(ii) cos(60° + A) sin(120° + A) = \(\frac{1}{2}\) [2 cos(60° + A) sin(120° + A)] [Multiply and divide by 2]
= \(\frac{1}{2}\) [sin((60° + A) + (120° + A))] – sin((60° + A) – (120° + A))]
[∵ 2 cos A sin B = sin(A + B) – sin(A – B)]
= \(\frac{1}{2}\) [sin(180° + 2A) – sin(60° + A – 120° – A)]
= \(\frac{1}{2}\) [(-sin 2A) – sin(-60°)]
= \(\frac{1}{2}\) [-sin 2A + sin 60°]
= \(\frac{1}{2}\) [-sin 2A + \(\frac{\sqrt{3}}{2}\)]
(iii) cos\(\frac{7 A}{3}\) sin\(\frac{5 A}{3}\)
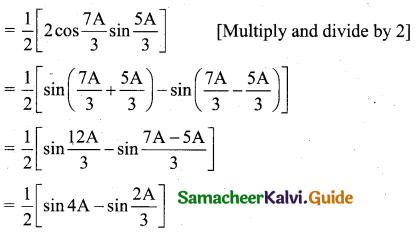
(iv) cos 7θ sin 3θ = \(\frac{1}{2}\) [sin(7θ + 3θ) – sin(7θ – 3θ)]
= \(\frac{1}{2}\) (sin 10θ – sin 4θ)
![]()
Question 2.
Express each of the following as the product of sine and cosine
(i) sin A + sin 2A
(ii) cos 2A + cos 4A
(iii) sin 6θ – sin 2θ
(iv) cos 2θ – cos θ
Solution:
(i) sin A + sin 2A = 2 sin(\(\frac{A+2 A}{2}\)) cos(\(\frac{A-2 A}{2}\))
[∵ sin C + sin D = sin(\(\frac{C+D}{2}\)) cos(\(\frac{C-D}{2}\))]
= 2 sin \(\frac{3A}{2}\) cos \(\frac{A}{2}\) [∵ cos(-θ) = cos θ]
(ii) cos 2A + cos 4A = 2 cos(\(\frac{2 \mathrm{A}+4 \mathrm{A}}{2}\)) cos(\(\frac{2 \mathrm{A}-4 \mathrm{A}}{2}\))
[∵ cos C + cos D = 2 cos(\(\frac{C+D}{2}\)) cos(\(\frac{C-D}{2}\))
= 2 cos(\(\frac{6 \mathrm{A}}{2}\)) cos(\(\frac{6 \mathrm{-2A}}{2}\))
= 2 cos(3A) cos (-A) [∵ cos(-θ) = cos θ]
= 2 cos 3A cos A
(iii) sin 6θ – sin 2θ = 2 cos(\(\frac{6 \theta+2 \theta}{2}\)) cos(\(\frac{6 \theta-2 \theta}{2}\))
[∵ sin C – sin D = 2 cos(\(\frac{C+D}{2}\)) sin(\(\frac{C-D}{2}\))
= 2 cos(\(\frac{8 \theta}{2}\)) sin(\(\frac{4 \theta}{2}\))
= 2 cos 4θ sin 2θ
(iv) cos 2θ – cos θ = -2 sin(\(\frac{2 \theta+\theta}{2}\)) sin(\(\frac{2 \theta-\theta}{2}\))
[∵ cos C – cos D = -2 sin(\(\frac{C+D}{2}\)) sin(\(\frac{C-D}{2}\))
= -2 sin(\(\frac{3 \theta}{2}\)) sin(\(\frac{\theta}{2}\))
![]()
Question 3.
Prove that
(i) cos 20° cos 40° cos 80° = \(\frac{1}{8}\)
(ii) tan 20° tan 40° tan 80° = √3.
Solution:
(i) cos 20° cos 40° cos 80° = \(\left(\frac{2 \sin 20^{\circ}}{2 \sin 20^{\circ}}\right)\) cos 20° cos 40° cos 80°
[multiply and divide by 2 sin 20°]
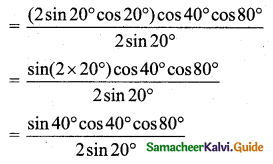
(Multiply and divide by 2)

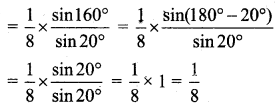
[∵ sin(180° – θ) = sin θ]
(ii) tan 20° tan 40° tan 80°

Consider sin 20° × sin 40° sin 80°
= sin 20° sin (60° – 20°) sin (60° + 20°)
= sin 20° [sin2 60° – sin2 20°]
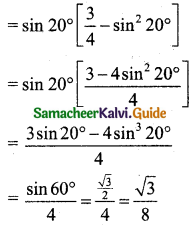
cos 20° × cos 40° cos 80° = \(\frac{1}{8}\) [∵ from (i)] …… (2)
divide (1) by (2) we get, tan 20° tan 40° tan 80° = \(\frac{\frac{\sqrt{3}}{8}}{\frac{1}{8}}\) = √3
Question 4.
Prove that
(i) (cos α – cos β)2 + (sin α – sin β)2 = 4 \(\sin ^{2}\left(\frac{\alpha-\beta}{2}\right)\)
(ii) sin A sin(60° + A) sin(60° – A) = sin 3A
Solution:
(i) LHS = (cos α – cos β)2 + (sin α – sin β)2
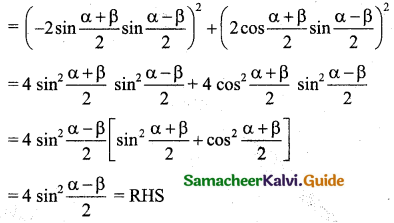
(ii) LHS = 4 sin A sin (60° + A) . sin (60° – A)
= 4 sin A {sin (60° + A) . sin (60° – A)}
= 4 sin A {sin2 60° – sin2 A}
= 4 sin A {\(\frac{3}{4}\) – sin2 A}
= 3 sin A – 4 sin3 A
= sin 3A
= RHS
![]()
Question 5.
Prove that
(i) sin (A – B) sin C + sin (B – C) sin A + sin(C – A) sin B = 0
(ii) \(2 \cos \frac{\pi}{13} \cos \frac{9 \pi}{13}+\cos \frac{3 \pi}{13}+\cos \frac{5 \pi}{13}=0\)
Solution:
Consider sin (A – B) sin C
= (sin A cos B – cos A sin B) sin C
= sin A cos B sin C – cos A sin B sin C …….. (1)
Similarly sin(B – C) sin A = sin B cos C sin A – cos B sin C sin A …….. (2)
[Replace A by B, B by C, C by A in (1)]
and sin(C – A) sin B [Replace A by B, B by C, C by A in (2)]
= sin C cos A sin B – cos C sin A sin B …….. (3)
Adding (1), (2) and (3) we get
sin (A – B) sin C + sin (B – C) sin A + sin(C – A) sin B = 0
(ii) \(2 \cos \frac{\pi}{13} \cos \frac{9 \pi}{13}+\cos \frac{3 \pi}{13}+\cos \frac{5 \pi}{13}=0\)
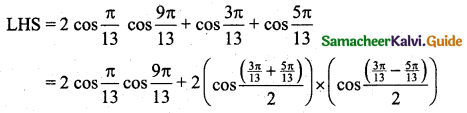
[∵ cos C + cos D = 2 cos(\(\frac{C+D}{2}\)) cos(\(\frac{C-D}{2}\))]
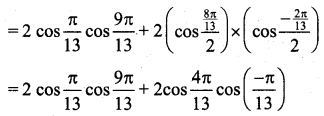
[∵ cos(-θ) = cos θ]
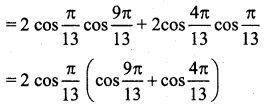
[take 2 cos \(\frac{\pi}{3}\) as commom)

Hence proved.
Question 6.
Prove that
(i) \(\frac{\cos 2 A-\cos 3 A}{\sin 2 A+\sin 3 A}=\tan \frac{A}{2}\)
(ii) \(\frac{\cos 7 \mathbf{A}+\cos 5 \mathbf{A}}{\sin 7 \mathbf{A}-\sin 5 \mathbf{A}}=\cot \mathbf{A}\)
Solution:
(i) \(\frac{\cos 2 A-\cos 3 A}{\sin 2 A+\sin 3 A}=\tan \frac{A}{2}\)
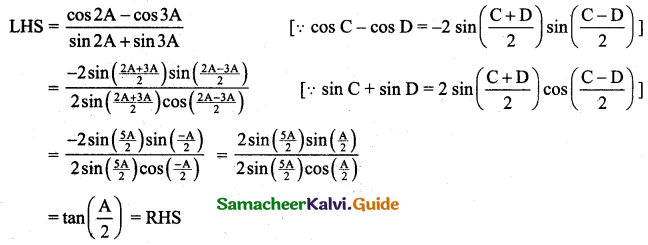
(ii) \(\frac{\cos 7 \mathbf{A}+\cos 5 \mathbf{A}}{\sin 7 \mathbf{A}-\sin 5 \mathbf{A}}=\cot \mathbf{A}\)
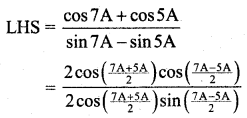
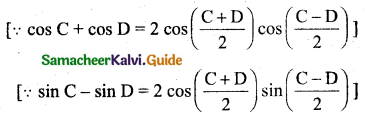

Hence proved.
![]()
Question 7.
Prove that cos 20° cos 40° cos 60° cos 80° = \(\frac{3}{16}\).
Solution:
LHS = cos 20° cos 40° cos 60° cos 80°
= cos 20° cos 40° (\(\frac{1}{2}\)) cos 80° [∵ cos 60° = \(\frac{1}{2}\)]
= \(\frac{1}{2}\) (cos 20° cos 40° cos 80°)
= \(\frac{1}{2}\left(\frac{2 \sin 20^{\circ}}{2 \sin 20^{\circ}}\right)\) (cos 20° cos 40° cos 80°)
[multiply and divide by 2 sin 20°]

[multiply and divide by 2]

Hence Proved.
Question 8.
Evaluate:
(i) cos 20° + cos 100° + cos 140°
(ii) sin 50° – sin 70° + sin 10°
Solution:
(i) LHS = (cos 20° + cos 100°) + cos 140°
= 2 \(\cos \left(\frac{20^{\circ}+100^{\circ}}{2}\right) \cos \left(\frac{20^{\circ}-100^{\circ}}{2}\right)\) + cos 140°
[∵ cos C + cos D = 2 cos(\(\frac{C+D}{2}\)) cos(\(\frac{C-D}{2}\))]
= 2 cos 60° cos(-40°) + cos 140°
= 2 × \(\frac{1}{2}\) × cos(-40°) + cos(180° – 140°)
[∵ cos(-θ) = cos θ, cos 60° = \(\frac{1}{2}\)
= cos 40° – cos 40°
= 0
Hence Proved.
(ii) LHS = (sin 50° – sin 70°) + sin 10°
= 2 \(\cos \left(\frac{50^{\circ}+70^{\circ}}{2}\right) \sin \left(\frac{50^{\circ}-70^{\circ}}{2}\right)\) + sin 10°
[∵ sin C – sin D = 2 cos(\(\frac{C+D}{2}\)) sin(\(\frac{C-D}{2}\))]
= 2 cos 60° sin(-10°) + sin 10°
= 2 × \(\frac{1}{2}\) (-sin 10°) + sin 10° [∵ sin(-θ) = -sin θ]
= -sin 10° + sin 10°
= 0
= RHS
![]()
Question 9.
If cos A + cos B = \(\frac{1}{2}\) and sin A + sin B = \(\frac{1}{4}\), prove that \(\tan \left(\frac{\mathbf{A}+\mathbf{B}}{2}\right)=\frac{\mathbf{1}}{2}\)
Solution:
Given that cos A + cos B = \(\frac{1}{2}\)
\(2 \cos \left(\frac{\mathrm{A}+\mathrm{B}}{2}\right) \cos \left(\frac{\mathrm{A}-\mathrm{B}}{2}\right)=\frac{1}{2}\) …….. (1)
Also given that sin A + sin B = \(\frac{1}{4}\)
\(2 \sin \left(\frac{\mathrm{A}+\mathrm{B}}{2}\right) \cos \left(\frac{\mathrm{A}-\mathrm{B}}{2}\right)=\frac{1}{4}\) ……. (2)
\(\frac{(2)}{(1)}\) gives

Question 10.
If sin(y + z – x), sin(z + x – y), sin(x + y – z) are in A.P, then prove that tan x, tan y and tan z are in A.P.
Solution:
In A.P. commom difference are equal, namely t2 – t1 = t3 – t2
sin(z + x – y) – sin(y + z – x) = sin(x + y – z) – sin(z + x – y)
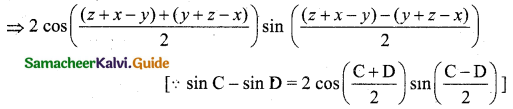
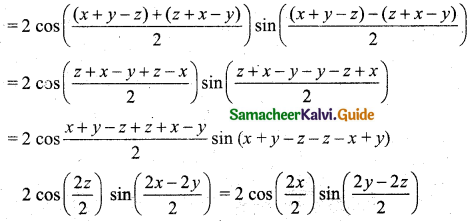
cos z sin (x – y) = cos x sin (y – z)
cos z (sin x cos y – cos x sin y) = cos x (sin y cos z – cos y sin z)
Divide bothsides by cos x cos y cos z we get
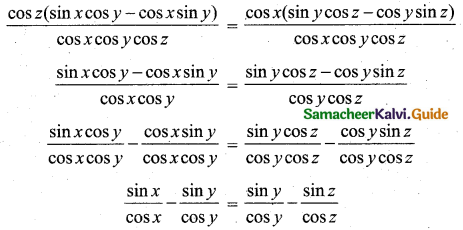
tan x – tan y = tan y – tan z
Multiply both sides by (-1) we get,
tan y – tan x = tan z – tan y
This means tan x, tan y, and tan z are in A.P.
Hence proved.
![]()
Question 11.
If cosec A + sec A = cosec B + sec B prove that cot(\(\frac{A+B}{2}\)) = tan A tan B.
Solution:
Given that cosec A + sec A = cosec B + sec B
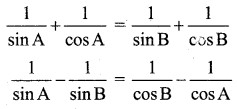
Arrange T-ratios of the sine and cosine in the separate side

[∵ sin C – sin D = 2 cos(\(\frac{C+D}{2}\)) sin(\(\frac{C-D}{2}\))]