Samacheer Kalvi 11th Maths Guide Chapter 9 Limits and Continuity Ex 9.5

Tamilnadu State Board New Syllabus Samacheer Kalvi 11th Maths Guide Pdf Chapter 9 Limits and Continuity Ex 9.5 Text Book Back Questions and Answers, Notes.

Tamilnadu Samacheer Kalvi 11th Maths Solutions Chapter 9 Limits and Continuity Ex 9.5

Question 1.
Prove that f(x) = 2x² +3x – 5 is continuous at all points in R.
Answer:
f(x) = 2x² + 3x – 5
Clearly f(x) is defined for all points of R.
Let x₀ be an arbitrary point in R. Then
f(x₀) = 2x₀² + 3x₀ – 5 ——- (1)

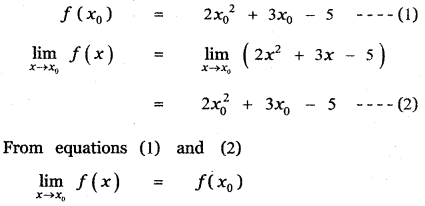
Thus, f(x) is defined at all points of R limit of f(x) exist at all points of R and is equal to the value of the function f (x).
Thus f (x) is continuous at all points of R.

Question 2.
Examine the continuity of the following
(i) x + sin x
Answer:
Let f(x) = sin x
f (x) is defined at all points of R.
Let x₀ be an arbitrary point in R.

= x_o + sin x₀ …….. (1)
f (x_o) = x_o + sin x_o ……… (2)
From equations (1) and (2) we get

∴ At all points of R, the limit of f (x) exists and is equal to the value of the function.
Thus, f( x) satisfies ail conditions for continuity.
Therefore, f(x) is continuous at all points of f(x).

(ii) X² cos x
Answer:
Let f(x) = x² cos x
f (x) is defined at all points of R.
Let x₀ be an arbitrary point in R. Then

= x²₀ cos ₀
f(x₀) = x²₀ cos ₀
From equation (1) and (2), we have

∴ The limit at x = x₀ exist and is equal to the value of the function f(x) at x = x₀.
Since x₀ is arbitrary, the limit of the function exist and is equal to the value of the function for all points in R.
∴ f( x) satisfies all conditions for continuity. Hence
f (x) is a continuous function in R.

(iii) e^x tan x
Answer:
Let f(x) = e^x tan x
f (x) is defined at ail points of R.
except at (2n + 1)\(\frac{\pi}{2}\), n ∈ Z.
Let x₀ be an arbitrary point in R – (2n + 1)\(\frac{\pi}{2}\), n ∈ Z

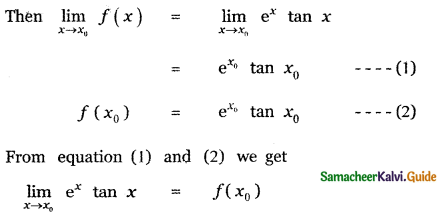
∴ Limit at x = x₀ exist and is equal to the value of the function f(x) at x = x₀.
Since x₀ is arbitrary the limit of the function. f(x) exists at all points in R – (2n + 1)\(\frac{\pi}{2}\), n ∈ Z and is equal to the value of the function f (x) at that points.
∴ f (x) satisfies all conditions for continuity. Hence,
f(x) is continuous at all points of R – (2n + 1)\(\frac{\pi}{2}\), n ∈ Z

(iv) e^2x + x²
Answer:
Let f(x) = e^2x + x²
Clearly, f(x) is defined for all points in R.
Let x₀ be an arbitrary point in R.

From equations (1) and (2) we have,
The limit of the function f(x) exist at x = x₀ and is equal to the value of the function f(x) at x – x₀.
Since x₀ is an arbitrary point in R, the above is true for all points in R. Hence f (x) satisfies all conditions for continuity. Hence f (x) is continuous at all points of R.

(v) x . log x
Answer:
Let f(x) = x log x
The function f(x) is defined in the open interval (0 , ∞) since log x is defined for x > 0. Let x₀ be an arbitrary point in (0, ∞). Then

= x₀ log x₀
f(x₀) = x₀ log x₀
From equation (1) and (2) we have

∴ The limit of the function f(x) exists at x = x₀ and is equal to the value of the function .
Since x₀ is an arbitrary point the above is true for all points in (0, ∞).
∴ f(x) is continuous at all points of (0, ∞).

(vi) \(\frac{\sin x}{x^{2}}\)
Answer:
f(x) = \(\frac{\sin x}{x^{2}}\)
f(x) is not defined at x = 0
∴ f(x) is defined for all points of R – {0}
Let x₀ be an arbitrary point in R – {0}. Then

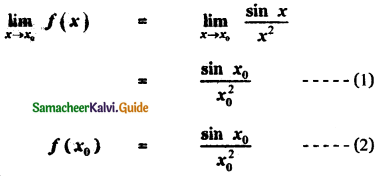
From equation (1) and (2) we have

∴ The limit of the function f(x) exist at x = x₀ and is equal to the value of the function f(x) at x = x₀.
Since x₀ is an arbitrary point in R – {0}, the above result is true for all points in R – {0}.
∴ f(x) is continuous at all points of R – {0}.

(vii) \(\frac{x^{2}-16}{x+4}\)
Answer:
Let f(x) = \(\frac{x^{2}-16}{x+4}\)
f(x) is not defined at x = – 4
∴ f(x) is defined for all points of R – {- 4}.
Let x₀ be an arbitrary point in R – {- 4}. Then

From equation (1) and (2) we have

Thus the limit of the function f (x) exist at x = x₀ and is equal to the value of the function f (x) at x = x₀.
Since x₀ is an arbitrary point in R – {- 4} the above result is true for all points in R – { – 4}.
∴ f(x) is continuous at all points of R – {- 4}.

(viii) |x + 2| + |x – 1|
Answer:
let f(x) = |x + 2| + |x – 1|
f( x) is defined for all points of R. Let x₀ be an arbitrary point in R. Then

From equation (1) and (2) we get

Thus the limit of the function f(x) exist at x = x₀ and is equal to the value of the function at x = x₀. Since x = x₀ is an arbitrary point in R, the above
result is true for all points in R. Hence f (x) is continuous at all points of R.

(ix) \(\frac{|x-2|}{|x+1|}\)
Answer:
Let f(x) = \(\frac{|x-2|}{|x+1|}\)
f(x) is defined for all points of R except at x = – 1.
∴ f (x) is defined for all points of R – { – 1 }.
Let x₀ be an arbitrary point in R – {- 1}.Then


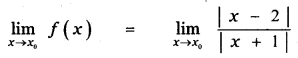
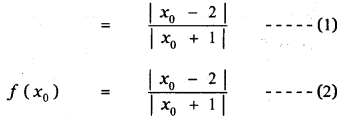
From equation (1) and (2) we have

Hence the limit of the function f(x) at x = x₀ exists and is equal to the value of the function at x = x₀.
Since x = x₀ is an arbitrary point in R – { – 1 }, the above result is true for all points in R – {- 1).
∴ f(x) is continuous at all points of R – {- 1}.

(x) cot x + tan x
Answer:
Let f(x) = cot x + tan x

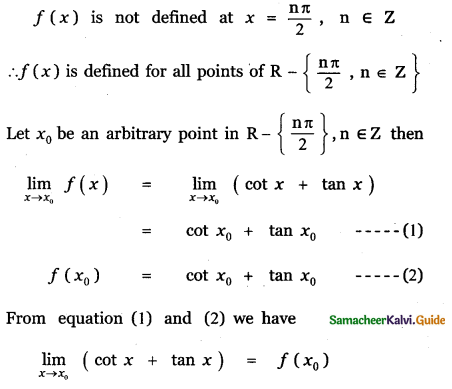
∴ The limit of the function f(x) exists at x = x₀ and is equal to the value of the function f (x) at x = x₀.
Since x₀ is an arbitrary point , the above result is true for all points of R – \(\left\{\frac{\mathrm{n} \pi}{2}\right\}\), n ∈ z.
∴ f(x) is continuous at all points of R – \(\left\{\frac{\mathrm{n} \pi}{2}\right\}\), n ∈ Z

Question 3.
Find the points of discontinuity of the function f, where
(i)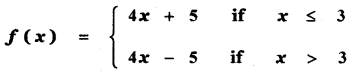
Answer:

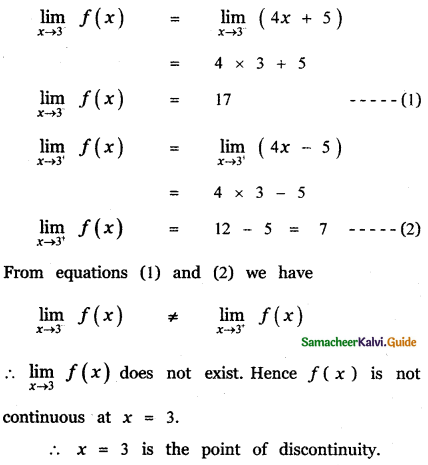
(ii)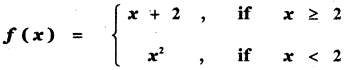
Answer:

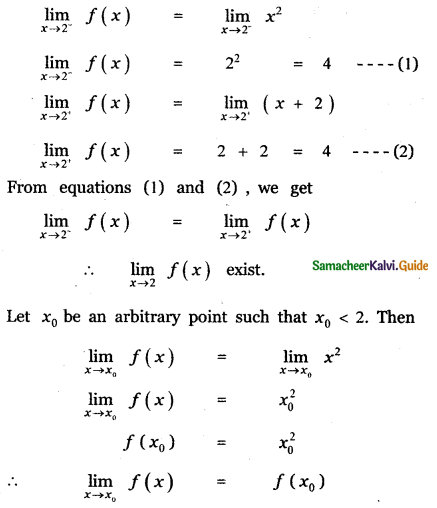
For the point x₀ < 2, we have the limit of the function that exists and is equal to the value of the function at that point.
Since x₀ is an arbitrary point the above result is true for all x < 2.
∴ f(x) is continuous in (-∞, 2).
Let x₀ be an arbitrary point such that x₀ > 2 then

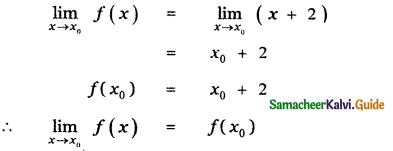
∴ For the point x₀ > 2, the limit of the function exists and is equal to the value of the function.
Since x₀ is an arbitrary point the above result is true for all x > 2.
∴ The function is continuous at all points of (2, ∞). Hence the given function is continuous at all points of R.

(iii)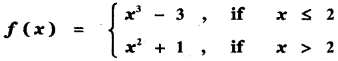
Answer:
Clearly, the given function is defined at all points of R.
Case (i) At x = 2

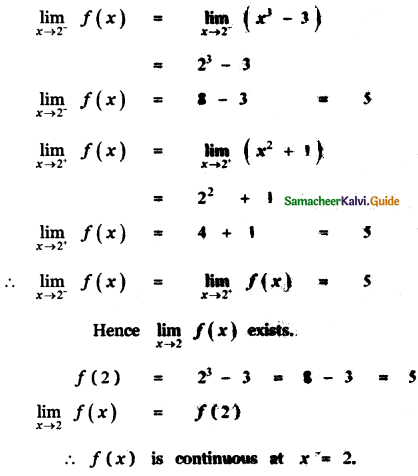
Case (ii) for x < 2
Let x₀ be an arbitrary point in (- ∞, 2).

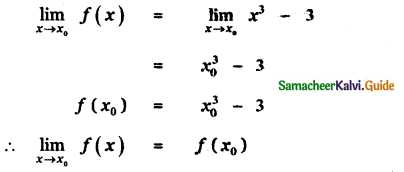
∴ f(x)is continuous at x = x₀ in (- ∞, 2).
Since x₀ is an arbitrary point in (- ∞, 2), f(x) is continuous at all points. f(-∞, 2).

Case (iii) for x > 2
Let y₀ be an arbitrary point in (2, ∞). Then


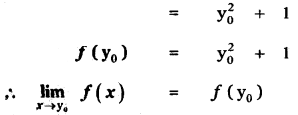
Hence, f(x) is continuous at x = y₀ in (2, ∞).
Since y_o is an arbitrary point of (2, ∞), f (x) is continuous at all points of (2, ∞).

∴ By case (i) case (ii) and case (iii) f(x) is continuous at all points of R.

(iv)
Answer:

Hence, f (x) is continuous at x = x₀. Since x₀ is an arbitrary point of \(\left(\frac{\pi}{4}, \frac{\pi}{2}\right)\), f(x) is continuous at all points of \(\left(\frac{\pi}{4}, \frac{\pi}{2}\right)\).
Hence, f (x) is continuous at all points \(\left[0, \frac{\pi}{2}\right)\),

Question 4.
At the given point x₀ discover whether the given function is continuous or discontinuous citing the reasons for your answer
(i)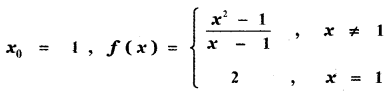
Answer:

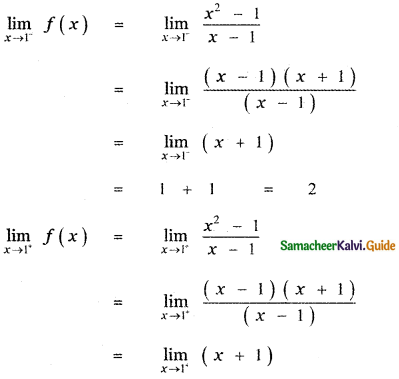
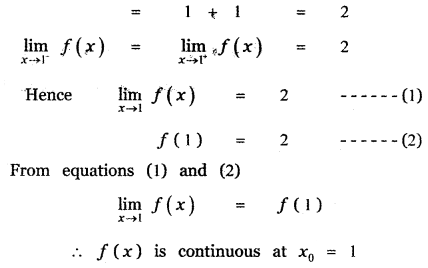
(ii)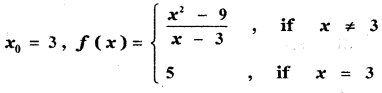
Answer:

Question 5.
Show that the function

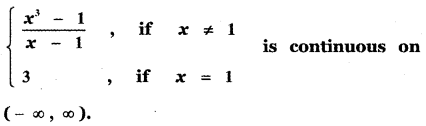
Answer:

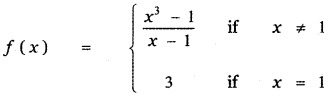
Clearly, the given function f(x) is defined at all points of R.
Case (i) Let x₀ ∈ (- ∞, 1) then

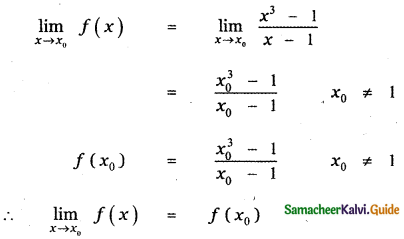
f (x) is continuous at x = x₀.
Since x₀ is arbitrary f(x) is continuous at all points of (-∞, 1).

Case (ii) Let x₀ ∈ (1, ∞) then

f (x) is continuous at x = x₀.
Since x₀ is an arbitrary point of (1, ∞), f(x) is continuous at all points of (1, ∞).

Case (iii) Let x₀ = 1 then

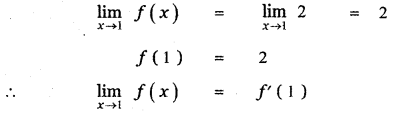
Hence, f (x) is continuous at x = 1.
Using all the three cases, we have f (x) is continuous at all the points of R.

Question 6.

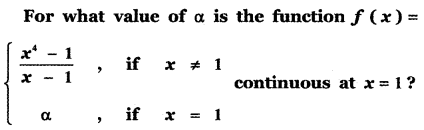
Answer:

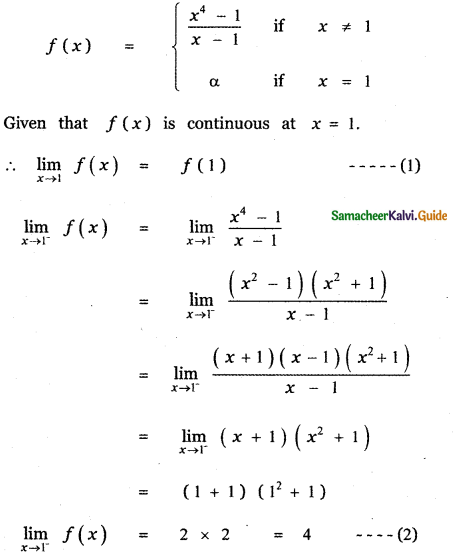
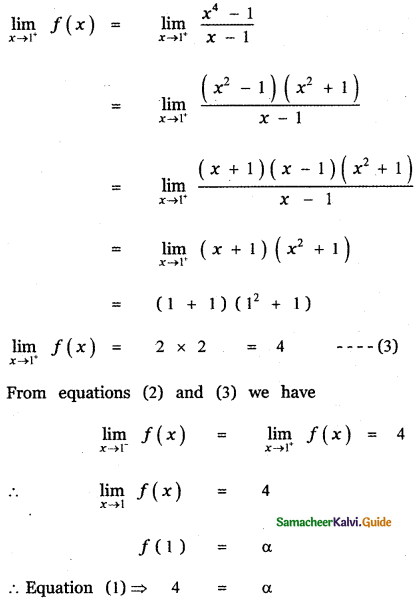
Question 7.

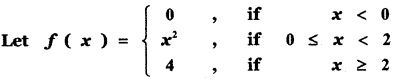
Graph the function. Show that f(x) continuous on (- ∞, ∞)
Answer:


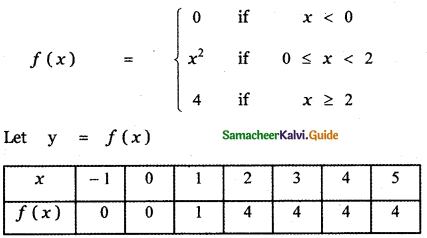
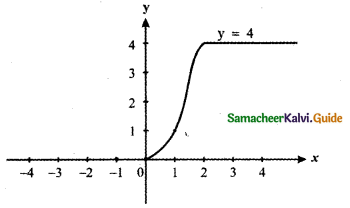
When x < 0 We have y = 0
When 0 ≤ x < 2 We have y = x²
When x ≥ 2 We have y = 4
Case (i) If x < 0 ie. (-∞, 0) then f (x) = 0
which is clearly continuous in (-∞, 0).

Case (ii) If 0 ≤ x < 2 je. [0 , 2)
Let x₀ be an arbitrary point in [0, 2)

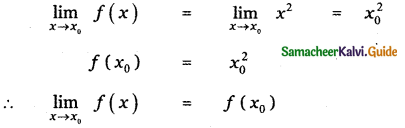
Hence f(x) is continuous at x = x₀. Since x = x₀ is an arbitrary f(x) is continuous at all points of [0, 2).

Case (iii) x ≥ 2 je. [2, ∞)
f( x) = 4 which is clearly continuous in [2 , ∞)

Case (iv) at x = 2,

f(2) = 4
∴ f(x) is continuous at x = 2.
∴ Using case (j) case (ii) case (iii) and case (iv) we have f(x) is continuous at all points of R.

Question 8.
If f and g are continuous functions with f(3) = 5 and [2f(x) – g(x)] = 4, find g(3)
Answer:
Given f and g are continuous functions.

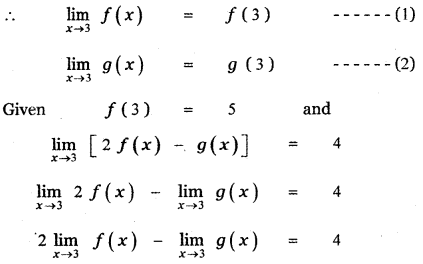
2 f(3) – g(3) = 4
2 × 5 – g(3) = 4
10 – 4 = g(3)
g(3) = 6

Question 9.
Find the points at which f is discontinuous. At which of these points f is continuous from the right, from the left, or neither? Sketch the graph of f.
(i)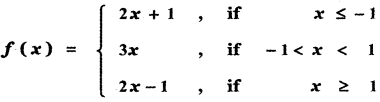
Answer:

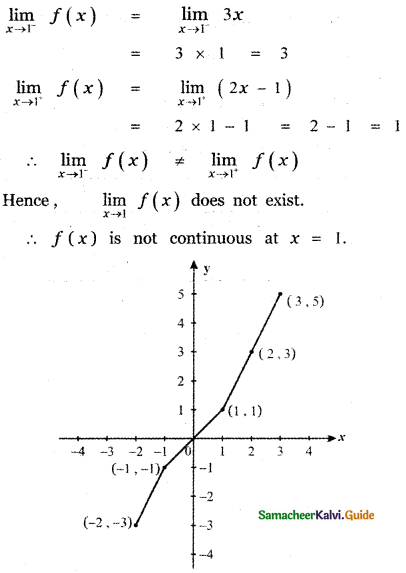
(ii)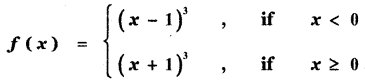
Answer:

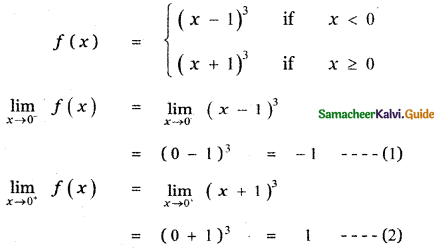
Question 10.
A function f is defined as follows:

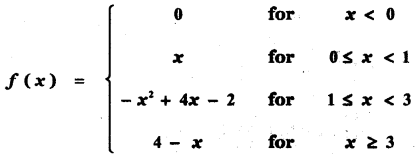
is the function continuous?
Answer:

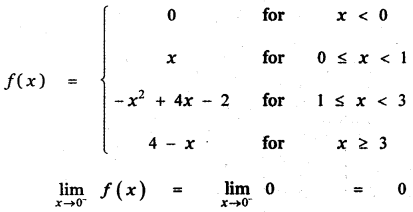
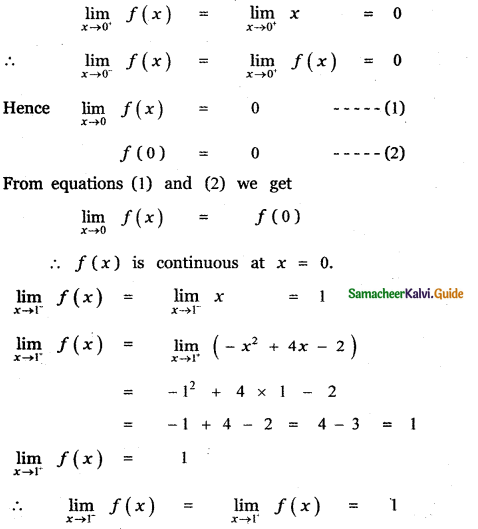
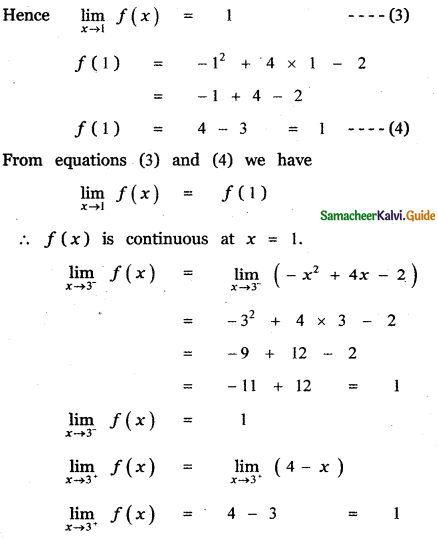
Question 11.
Which of the following functions f has a removable discontinuity at x = x₀? If the discontinuity is removable, find a function g that agrees with f for x ≠ x₀ and is continuous on R
(i)
Answer:
f(x) is not defined at x = -2

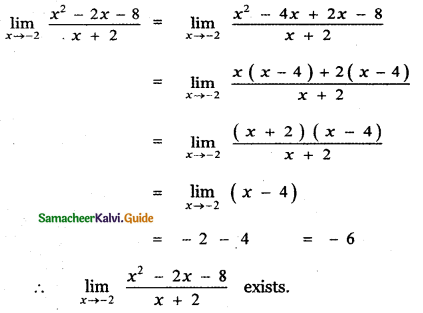
Redefine the function f(x) as

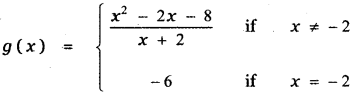
∴ f (x) has a removable discontinuity at x = -2.
Clearly, g (x) is continuous on R.

(ii)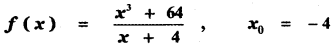
Answer:
The function f(x) is not defined at x = -4.

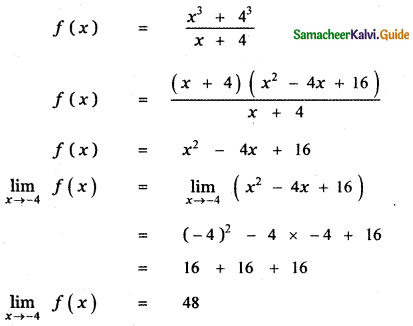
Limit the function f (x) exist at x = -4.
∴ The function f (x) has a removable discontinuity at x = -4.
Redefine the function f (x) as

Clearly, the function g(x) is continuous on R.

(iii)
The function f(x) is not defined at x = 9.


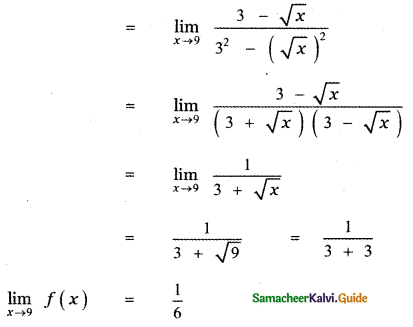
∴ Limit of the function f(x) exists at x = 9.
Hence, the function f(x) has a removable discontinuity at x = 9. Redefine the function f(x) as

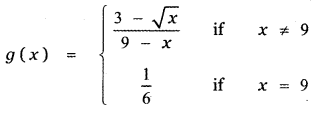
Clearly, g (x) is defined at all points of R and is continuous on R.

Question 12.
Find the constant b that makes g continuous on (-∞, ∞).

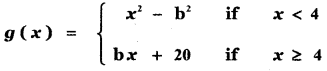
Answer:

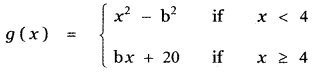
Given g is continuous on R.
∴ g (x) is continuous at x = 4.

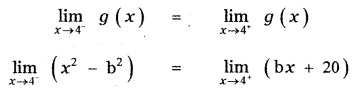
4² – b² = b × 4 + 20
16 – b² = 4b + 20
b² + 4b + 20 – 16 = 0
b² + 4b + 4 = 0
(b + 2)² = 0
b + 2 = 0 ⇒ b = -2

Question 13.
Consider the function f (x) = x sin \(\frac{\pi}{x}\) What value must we give f (0) in order to make the function continuous everywhere?
Answer:
f(x) = x sin \(\frac{\pi}{x}\)
Define f(x) on R as

∴ f(0) = 0. Then f(x) is continuous on R.

Question 14.
The function f(x) = \(\frac{x^{2}-1}{x^{3}-1}\) is not defined at x = 1. What value must we give f(1) in order to make f(x) continuous at x = 1 ?
Answer:


The function f (x) has a removable discontinuity at x = 1. Redefine f (x) as

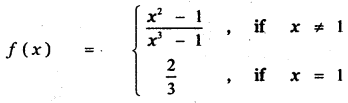
∴ f(1) = \(\frac{2}{3}\). Then f(x) will be continuous at x = 1

Question 15.
State how continuity is destroyed at x = x₀ for each of the following graphs.
Answer:
(a)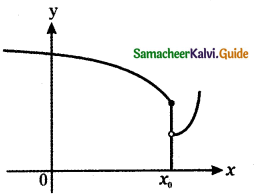
The left – hand limit and right hand limit does not coincide at x = x₀

(b)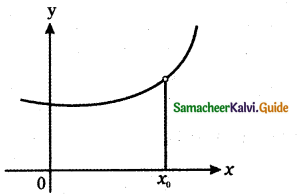
The function f(x) is not defined at x = x₀ and hence the continuity is destroyed at x = x₀

(c)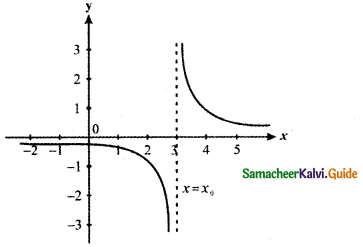
The limit of f(x) does not exist at x = x₀

(d)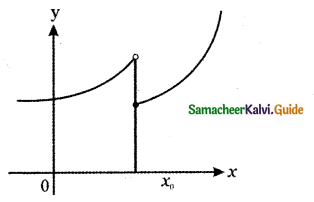
The left hand limit and right – hand limit does not coincide at x = x₀