Students can download Maths Chapter 1 Set Language Ex 1.2 Questions and Answers, Notes, Samacheer Kalvi 9th Maths Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus, helps students complete homework assignments and to score high marks in board exams.
Tamilnadu Samacheer Kalvi 9th Maths Solutions Chapter 1 Set Language Ex 1.2
Question 1.
Find the cardinal number of the following sets.
(i) M = {p, q, r, s, t, u}
(ii) P = {x : x = 3n + 2, n ∈ W and x < 15}
(iii) Q = {y : y = \(\frac{4}{3n}\), n ∈ N and 2 < n ≤ 5}
(iv) R = {x : x is an integer, x ∈ Z and – 5 ≤ x < 5}
(v) S = The set of all leap years between 1882 and 1906.
Solution:
(i) n (M) = 6
(ii) n (P) = 5 [n = {0, 1, 2, 3 . . . . 14}]
(iii) Since n = {3, 4, 5} ; n (Q) = 3
(iv) X = {-5, -4, -3, -2, -1, 0, 1, 2, 3, 4} ∴ n (R) = 10
(v) S = {1884, 1888, 1892, 1896, 1904}; n (S) = 5
![]()
Question 2.
Identify the following sets as finite or infinite.
(i) X = The set of all districts in Tamilnadu.
(ii) Y = The set of all straight lines passing through a point.
(iii) A = {x : x ∈ Z and x < 5}
(iv) B = {x : x² – 5x + 6 = 0, x ∈ N}
Solution:
(i) Finite
(ii) Infinite set (many lines can be drawn from a point)
(iii) Infinite set {A = ……. -2, -1, 0, 1, 2, 3, 4}
(iv) Finite set [x² – 5x + 6 = 0 ⇒ (x – 3) (x – 2) = 0; x = 3 and 2]
Question 3.
Which of the following sets are equivalent or unequal or equal sets?
(i) A = The set of vowels in the English alphabets.
B = The set of all letters in the word “VOWEL”
(ii) C = {2, 3, 4, 5}
D = {x : x ∈ W, 1 < x < 5}
(iii) X = {x : x is a letter in the word “LIFE”}
Y = {F, I, L, E}
(iv) G = {x : x is a prime number and 3 < x < 23}
H = {x : x is a divisor of 18}
Solution:
(i) Equivalent set [n(A) = n(B) = 5] ∴ A ≈ B
(ii) Unequal sets [C = {2, 3, 4, 5}; D = {2, 3, 4}]
(iii) Equal sets [X = {L, I, F, E}; Y = {F, I, L, E} [n(X) = 4 = n(Y)] ∴ X ≈ Y
(iv) Equivalent sets [G = {5, 7, 11, 13, 17, 19}; H = {1, 2, 3, 6, 9, 18}]
[n(G) = n(H) = 6 ∴ G ≈ H)]
![]()
Question 4.
Identify the following sets as null set or singleton set.
(i) A = {x : x ∈ N, 1 < x < 2}
(ii) B = The set of all even natural numbers which are not divisible by 2
(iii) C = {0}.
(iv) D = The set of all triangles having four sides.
Solution:
(i) Null set [No natural numbers is in between 1 and 2]
(ii) Null set [All the even natural numbers are not divisible by 2]
(Hi) Singleton set [n (C) = 1]
(iv) Null set [All the triangles has 3 sides]
Question 5.
State which pairs of sets are disjoint or overlapping?
(i) A = {f, i, a, s} and B = {a, n, f, h, s}
A = {f, i, a, s} and B = {a, n, f, h, s}
A and B are overlapping sets
(ii) C = {x : x is a prime number, x > 2} and D = {x : x is an even prime number}
C= {3, 5, 7…….}
D = {2}
C and D are disjoint sets
(iii) E = {x : x is a factor of 24} and F = {x : x is a multiple of 3, x < 30}
E = {1, 2, 3, 4, 6, 8, 12, 24}
F = {3, 6, 9, 12, 15, 18, 21, 24, 27} [Hint: E ∩ F = {3, 6, 24, …….}]
E and F are overlapping sets
![]()
Question 6.
If S = {square, rectangle, circle, rhombus, triangle}. List the elements of the following subset of S.
(i) The set of shapes which have 4 equal sides.
(ii) The set of shapes which have radius.
(iii) The set of shapes in which the sum of all interior angles is 180°.
(iv) The set of shapes which have 5 sides.
Solution:
(i) Subset of S = {square, rhombus}
(ii) Subset of S = {circle}
(iii) Subset of S = {triangle}
(iv) Subset of S = { }
Question 7.
If A = {a,{a, b}}, write all the subsets of A.
Solution:
A = {a, {a, b}}
Subset of A are Ø, {a}, {a, b}, {a, {a, b}} (or) { }, {a}, {a,b, {a,{a,b}}
P(A) = {Ø, {a}, {a, b}, {a {a, b}} (or) {{ }, {a}, {a,b, {a,{a,b}}
![]()
Question 8.
Write down the power set of the following sets.
(i) A = {a, b}
(ii) B = {1, 2, 3}
(iii) D = {p, q, r, s}
(iv) E = Ø
Solution:
(i) A = {a, b)
P(A) = {{},{a},{b}, {a, b}}
(ii) B = {1, 2, 3}
P(B) = {{}, {1}, {2}, {3}, {1,2}, {2, 3}, {1,3}, {1,2,3}}
(iii) D = {p, q, r, s}
P(D) = {{},{p},{q},{r},{s},{p, q} {p, r} {p, s}
{q, r}, {q, s}, {r, s}, {p, q, r} {q, r, s}
{p, r, s} {p, q, s} {p, q, r, s}}
(iv) E = Ø
P(E) = {{}}
Note: (empty set is the subset of all the sets)
![]()
Question 9.
Find the number of subsets and the number of proper subsets of the following sets.
(i) W = {red, blue, yellow}
(ii) X = {x² : x ∈ N, x² ≤ 100}
Solution:
(i) W = {red, blue, yellow}
n (W) = 3
The number of subsets of W = n [P(W)] = 2m
= 23 = 8
Number of proper subsets of W = n[P(W)] – 1
= 8 – 1
= 7 (or)
Number of proper subsets of W = 2m – 1
= 23 – 1 = 8 – 1 = 7
(ii) X = {x2 : x ∈ N, x2 ≤ 100}.
X= {1,2, 3, 4, …. 10}
n(X) = 10
The number of subsets of X = n[P(X)]
= 2m
= 210 = 1024
Number of proper subsets of X = 2m – 1
= 1024 – 1
= 1023
![]()
Question 10.
(i) If n(A) = 4, find n[P(A)]
(ii) If n(A) = 0, find n[P(A)]
(Hi) If n[P(A)] = 256, find n(A)
Solution:
(i) n (A) = 4
n [P(A)] = 2m = 24
= 16
(ii) n (A) = 0
n [P(A)] = 2m = 2° = 1
(iii) n [P(A)] = 256
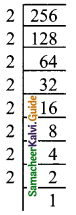
2m = 28
∴ n (A) = 8