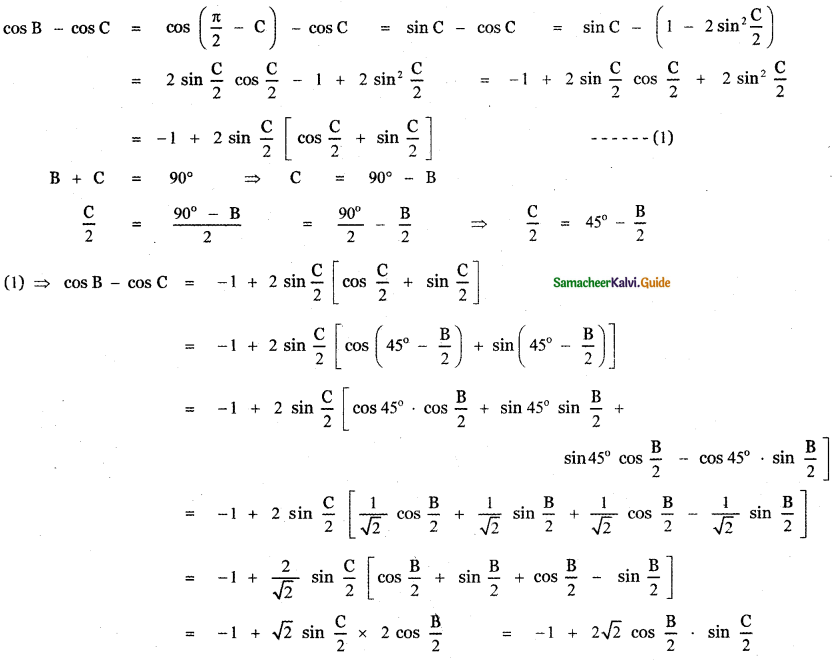Tamilnadu State Board New Syllabus Samacheer Kalvi 11th Maths Guide Pdf Chapter 3 Trigonometry Ex 3.7 Text Book Back Questions and Answers, Notes.
Tamilnadu Samacheer Kalvi 11th Maths Solutions Chapter 3 Trigonometry Ex 3.7
Question 1.
If A + B + C = 180° prove that
(i) sin 2A + sin 2B + sin 2C = 4 sin A sin B sin C
Answer:
sin 2A + sin 2 B + sin 2 C = 2 sin \(\left(\frac{2 \mathrm{~A}+2 \mathrm{~B}}{2}\right)\) . cos \(\left(\frac{2 \mathrm{~A}-2 \mathrm{~B}}{2}\right)\) + 2 sin C cos C
= 2 sin (A + B) . cos (A – B) + 2 sin C cos C
= 2 sin( 180° – C) cos (A – B) + 2 sin C cos C (∴ A + B + C = 180°)
= 2 sin C cos (A – B) + 2 sin C cos C
= 2 sin C (cos(A – B) + cos C)
= 2 sin C [(cos (A – B) + cos (180° – (A + B))]
= 2 sin C [cos (A – B) – cos (A + B)]
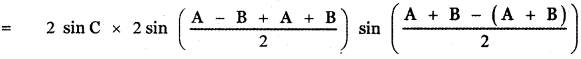
= 2 sin C . 2 sin A . sin B
= 4 sin A sin B sin C
![]()
(ii) cos A + cos B – cos C = -1 + 4 cos \(\frac{\mathbf{A}}{2}\) cos \(\frac{B}{2}\) sin \(\frac{\mathbf{c}}{2}\)
Answer:
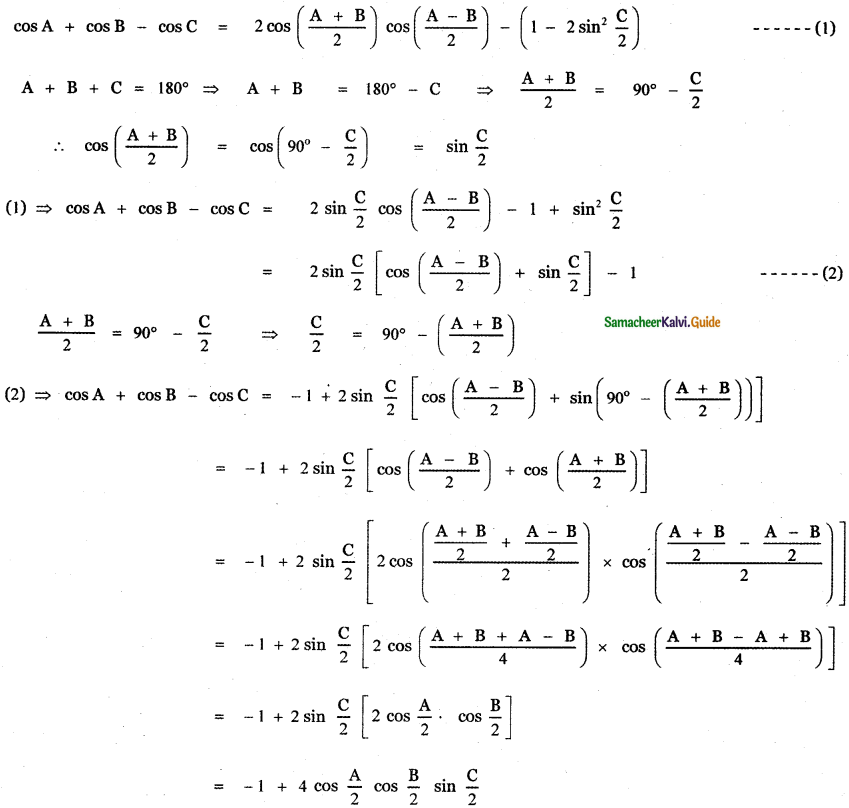
![]()
(iii) sin 2A + sin 2B + sin 2C = 2 + 2 cos A cos B cos C
Answer:

![]()
(iv) sin 2A + sin 2B – sin 2C = 2 sin A sin B cos C
Answer:
Given A + B + C = 180° ⇒ c = 180° – (A + B)
![]()
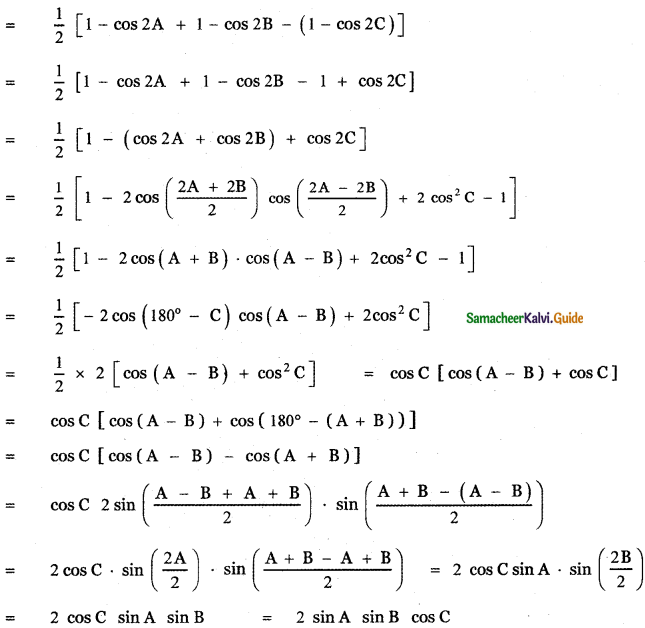
![]()
(v) ![]()
Answer:
Given A + B + C = 180° ⇒ A + B = 180° – C

![]()
(vi) sin A + sin B + sin C = 4 cos \(\frac{\mathbf{A}}{2}\) cos \(\frac{\mathbf{B}}{2}\) cos \(\frac{\mathbf{c}}{2}\)
Answer:

![]()
(vii) sin(B + C – A) + sin(C + A – B) + sin(A + B – C) = 4 sin A sin B sin C
Answer:
Given A + B + C = 180°
sin ( B + C – A) = sin (180° – A – A) = sin (180° – 2A) = sin 2A
sin ( C + A – B) = sin (180° – B – B) = sin ( 180° – 2B) = sin 2B
sin (A + B – C) = sin (180° – C – C) = sin ( 180° – 2C) = sin 2C
∴ sin ( B + C – A) + sin ( C + A – B) + sin (A + B – C) = sin 2A + sin 2B + sin 2C
= 2 sin \(\left(\frac{2 \mathrm{~A}+2 \mathrm{~B}}{2}\right)\) . cos \(\left(\frac{2 \mathrm{~A}-2 \mathrm{~B}}{2}\right)\) + 2 sin C . cos C
= 2 sin(A + B) . cos(A – B) + 2 sin C cos C
= 2 sin(180° – C) cos(A – B) + 2 sin C cos C
= 2 sin C cos(A – B) + 2 sin C cos C
= 2sin C(cos(A – B) + cos C)
= 2 sin C [cos(A – B) + cos (180° -(A + B))]
= 2 sin C [cos(A – B) – cos(A + B)]
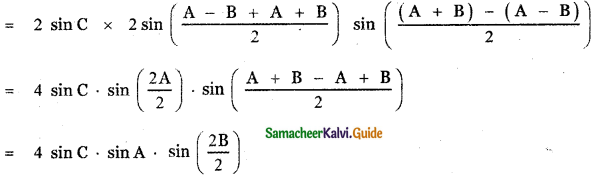
![]()
Question 2.
If A + B + C = 2s, then prove that sin (s – A) sin (s- B )+ sin s . sin(s – C) = sin A sin B
Answer:
Given A + B + C = 2s , we have sin A sin B = \(\frac { 1 }{ 2 }\) [cos ( A – B) – cos ( A + B)]
sin(s – A) sin(s – B) + sins . sin(s – C) = \(\frac { 1 }{ 2 }\) [cos((s – A) – (s – B)) – cos(s – A + s – B)] + \(\frac { 1 }{ 2 }\) [cos (s – (s – C)) – cos (s + s – C)]
= \(\frac { 1 }{ 2 }\) [cos (s – A – s + B) – cos(2s – A – B)] + \(\frac { 1 }{ 2 }\) [cos(s – s + C) – cos(2s – C)]
= \(\frac { 1 }{ 2 }\) [cos (B – A) – cos(2s – A – B)] + \(\frac { 1 }{ 2 }\) [cos C – cos(2s – C)]
= \(\frac { 1 }{ 2 }\) [cos(A – B) – cos(A + B + C – A – B)] + \(\frac { 1 }{ 2 }\) [cos C – cos (A + B + C – C)]
= \(\frac { 1 }{ 2 }\) [cos (A – B) – cos C] + \(\frac { 1 }{ 2 }\) [cos C – cos(A + B)]
= \(\frac { 1 }{ 2 }\) [cos (A – B) – cos C + cos C – cos (A + B)] = \(\frac { 1 }{ 2 }\) [cos(A – B) – cos(A + B)]
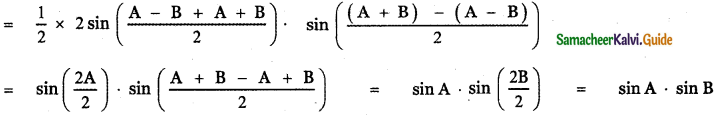
![]()
Question 3.
If x + y + z = xyz prove that
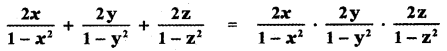
Answer:
Given x + y + z = x y z ,
Let x = tan A,
y = tan B,
z = tan C
x + y + z = xyz ⇒ tan A + tan B + tan C = tan A tan B tan C
tan A + tan B + tan C – tan A tan B tan C = 0 ——– (1)
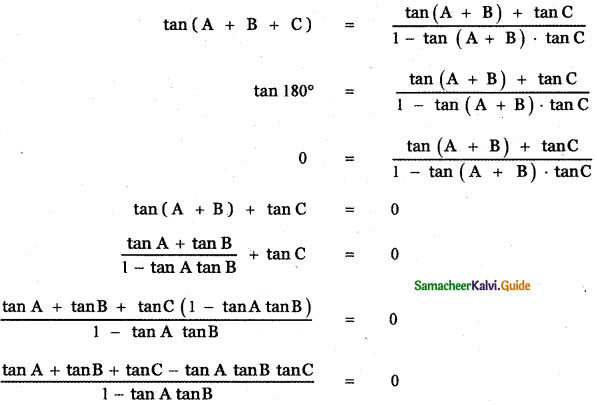
tan A + tan B + tan C – tan A tan B tan C = 0 ——– (2)
A + B + C = 180° ⇒ tan A + tan B + tan C – tan A tan B tan C = 0
⇒ tan (A + B + C) = 0
⇒ tan A + tan B + tan C – tan A tan B tan C = 0
⇒ tan (A + B + C) = 0
∴ tan 2(A + B + C) = o
⇒ tan (2A + 2B + 2C) = 0
⇒ tan 2A + tan 2B + tan 2C – tan 2A tan 2B tan 2C = 0 By eqn (1)
tan 2 A + tan 2B + tan 2C = tan 2A tan 2B tan 2C
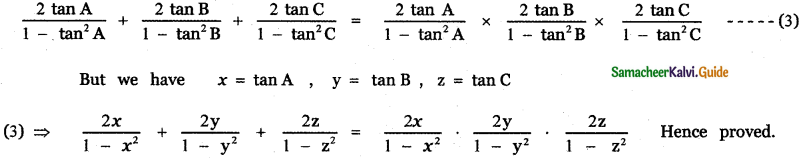
![]()
Question 4.
If A + B + C = prove the following
(i) sin 2A + sin 2B + sin 2C = 4 cos A cos B cos C
Answer:
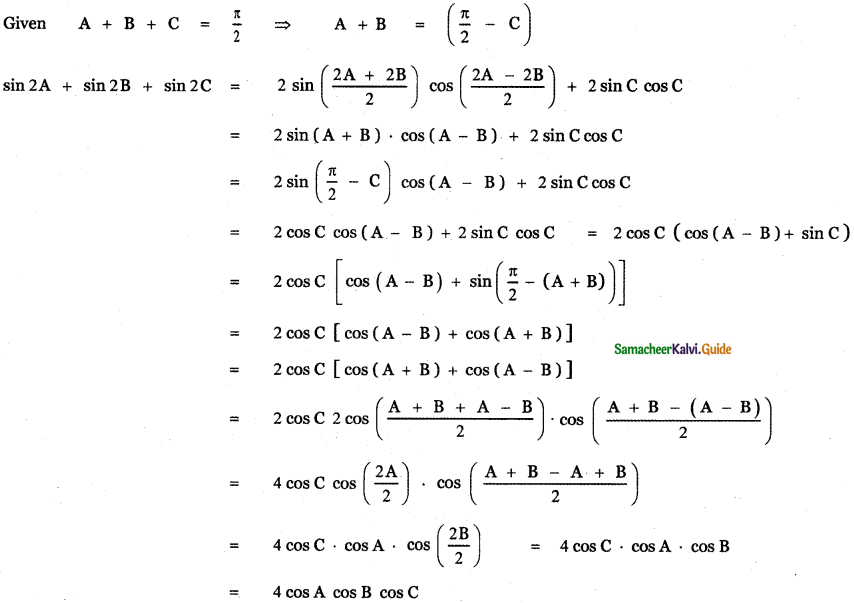
![]()
(ii) cos 2A + cos 2B + cos 2C = 1 + 4 sin A sin B sin C
Answer:
![]()

![]()
Question 5.
If ∆ABC is a right triangle and if ∠A = \(\frac{\pi}{2}\) then prove that
(i) cos2 B + cos2 C = 1
Answer:
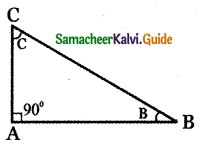
∆ ABC is a right triangle. Given ∠A = 90°
we know A + B + C = 180°
∴ B + C = 180° – A
B + C = 180° – 90° = 90°

(ii) sin2 B + sin2 C = 1
Answer:

![]()
(iii) cos B – cos C = -1 + 2√2 cos \(\frac{\mathbf{B}}{2}\) . sin \(\frac{\mathbf{C}}{2}\)
Answer: