Tamilnadu State Board New Syllabus Samacheer Kalvi 12th Maths Guide Pdf Chapter 2 Complex Numbers Ex 2.9 Textbook Questions and Answers, Notes.
Tamilnadu Samacheer Kalvi 12th Maths Solutions Chapter 2 Complex Numbers Ex 2.9
Choose the most suitable answer.
Question 1.
in + in+1+ in+2 + in+3 is:
(a) 0
(b) 1
(c) -1
(d) z
Solution:
(a) 0
Hint:
in + in+1+ in+2 + in+3
= in[1 + i + i² + i³]
= in[1 + i – 1 – i]
in (0) = 0
Question 2.
The value of \(\sum _{ i=1 }^{ 13 }{ ({ i }^{ n }+{ i }^{ n-1 }) } \) is
(a) 1 + i
(b) i
(c) 1
(d) 0
Solution:
(a) 1 + i
Hint:
\(\sum _{ i=1 }^{ 13 }{ ({ i }^{ n }+{ i }^{ n-1 }) } \)= (i1 + i² + i3 + … + i13) + (i0 + i1 + i2 + … + i12)
= i0 + 2(i1 + i² + i3 + ….. i12) + i13
= 1 + 2(i – 1 – i + 1 + … + 1) + 1
= 1 + 2(0) + i = 1 + i
![]()
Question 3.
The area of the triangle formed by the complex numbers z, iz, and z + iz in the Argand’s diagram is:
(a) \(\frac{1}{2}\) |z|²
(b) |z|²
(c) \(\frac{3}{2}\) |z|²
(d) 2|z|²
Solution:
(a) \(\frac{1}{2}\) |z|²
Hint:
Area of the triangle formed by the complex numbers z, iz and z + iz.
Let z = a + ib ⇒point (a, b)
iz = – b + ia ⇒ point (- b, a)
z + iz =(a – b) + i(a + b) point((a – b),(a + b))
Area of the triangle
= \(\frac {1}{2}\) [x1(y2 – y3) + x2(y3 – y1) + x3(y1 – y2)]
= \(\frac {1}{2}\) [a(a – a – b) -b(a + b – b) + (a – b)(b – a)]
= \(\frac {1}{2}\) [-ab – ab + ab – a² – b² + ab]
Question 4.
The conjugate of a complex number is \(\frac{1}{i-2}\) Then, the complex number is:
(a) \(\frac{1}{i+2}\)
(b) \(\frac{-1}{i+2}\)
(c) \(\frac{-1}{i-2}\)
(d) \(\frac{1}{i-2}\)
Solution:
(b) \(\frac{-1}{i+2}\)
Hint:
Conjugate of complex number is \(\frac{1}{i-2}\)
∴ the complex number is \(\frac{-1}{i+2}\)
Question 5.
If z = \(\frac{(√3+i)^3(3i+4)²}{(8+6i)²}\)
(a) 0
(b) 1
(c) 2
(d) 3
Solution:
(c) 2
Hint:
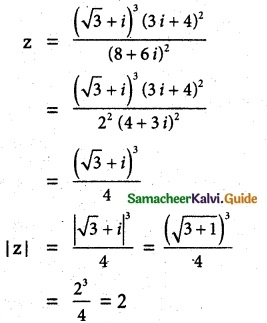
![]()
Question 6.
If z is a non zero complex number, such that 2iz² = \(\bar { z }\) then | z | is:
(a) \(\frac{1}{2}\)
(b) 0
(c) 1
(d) 2
Solution:
z is a non zero complex number
Given 2iz² = \(\bar { z }\)
let z = x + iy
2i(x + iy)² = x – iy
simplifying 2i(x² – y² + 2ixy) = x – iy
-4xy + 2i(x² – y²) = x – iy
Equating real and imaginary parts
-4xy = x, 2(x² – y²) = -y
solving x = \(\frac{√3}{4}\), y =-\(\frac{1}{4}\)
z = \(\frac{√3}{4}\) – \(\frac{i}{4}\)
|z| = \(\sqrt { \frac{3}{16}+\frac{1}{16}}\) = \(\sqrt { \frac{1}{4}}\)
= \(\frac {1}{2}\)
Question 7.
If |z – 2 + i | ≤ 2, then the greatest value of |z| is:
(a) √3 – 2
(b) √3 + 2
(c) √5 – 2
(d) √5 + 2
Solution:
(d) √5 + 2
Hint:
|z – 2 + i | ≤ 2
|z + (-2 + i)| ≤ |z| + |-2 + i|
|z| ± √5
Given |z – 2 + i| ≤ 2
∴ |z| ± √5 ≤ 2
|z| ≤ 2 – √5. |z| ≤ 2 + √5
∴ The greatest value is 2 + √5
Question 8.
If |z – \(\frac{3}{2}\)| = 2 then the least value of |z| is:
(a) 1
(b) 2
(c) 3
(d) 5
Solution:
(a) 1
Hint:

|z|² – 2 |z| + 1 ≤ 3 + 1
(|z| – 1)² ≤ 4
|z| – 1 ≤ ± 2
|z| ≤ 2 + 1 and |z| ≤ – 2 + 1
|z| = -1
But |z| = 1
![]()
Question 9.
If |z| = 1, then the value of \( \frac { 1+z }{ 1+\bar { z } } \) is
(a) z
(b) \( \bar { z } \)
(c) \(\frac{1}{z}\)
(d) 1
Solution:
(a) z
Hint:

Question 10.
The solution of the equation |z| – z = 1 + 2i is:
(a) \(\frac{3}{2}\) – 2i
(b) – \(\frac{3}{2}\) + 2i
(c) 2 – \(\frac{3}{2}\)i
(d) 2 + \(\frac{3}{2}\)i
Solution:
(a) \(\frac{3}{2}\) – 2i
Hint:
|z| – z = 1 + 2i
Let z = x + iy
|z| = x + iy + 1 + 2i
\(\sqrt{x^2+y^2}\) = (x + 1) + i(y + 2)
\(\sqrt{x^2+y^2}\) = x + 1 y + 2 = 0
x² + y² = (x + 1)² y = -2
y² = 2x + 1
2x = 3
x = \(\frac{3}{2}\)
∴z = x + iy = \(\frac{3}{2}\) – 2i
Question 11.
If |z1| = 1,|z2| = 2, |z3| = 3 and |9z1z2 + 4z1z3 + z2z3| = 12, then the value of |z1 + z2 + z3| is:
(a) 1
(b) 2
(c) 3
(d) 4
Solution:
(b) 2
Hint:
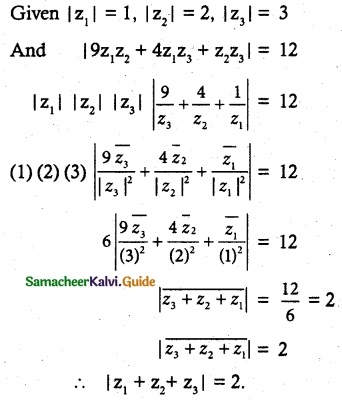
![]()
Question 12.
If z is a complex number such that z∈C\R and z + \(\frac{1}{z}\) ∈R, then |z| is:
(a) 0
(b) 1
(c) 2
(d) 3
Solution:
(b) 1
Hint:
If z ∈ C\R and z + \(\frac{1}{z}\) ∈ R
Then |z| = 1
Question 13.
z1, z2 and z3 are complex numbers such that z1 + z2 + z3 = 0 and |z1| = |z2| = |z3| = 1 then \({ z }_{ 1 }^{ 2 }+{ z }_{ 2 }^{ 2 }+{ z }_{ 3 }^{ 2 }\) is
(a) 3
(b) 2
(c) 1
(d) 0
Solution:
(d) 0
Question 14.
If \(\frac{z-1}{z+1}\)is purely imaginary, then |z| is
(a) \(\frac{1}{2}\)
(b) 1
(c) 2
(d) 3
Solution:
(b) 1
Hint:
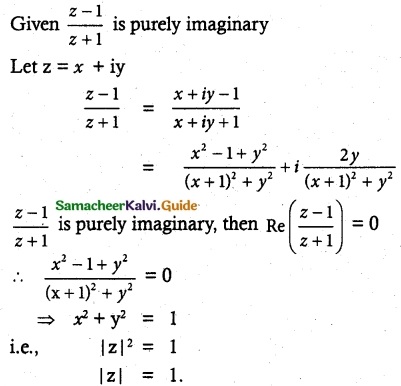
![]()
Question 15.
If z = x + iy is a complex number such that |z + 2| = |z – 2|, then the locus of z is:
(a) real axis
(b) imaginary axis
(c) ellipse
(d) circle
Solution:
(b) imaginary axis
Hint:
|z + 2| = |z – 2|
Let z = x + iy
|(x + 2) + iy| = |(x – 2) + iy|
(x + 2)² + y² = (x – 2)² + y²
x² + 4x + 4 = x² – 4x + 4
⇒ x = 0
Question 16.
The principal argument of \(\frac{3}{-1+i}\) is:
(a) \(\frac{-5π}{6}\)
(b) \(\frac{-2π}{3}\)
(c) \(\frac{-3π}{4}\)
(d) \(\frac{-π}{2}\)
Solution:
(c) \(\frac{-3π}{4}\)
Hint:
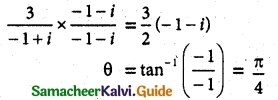
Since Real and imaginary parts are negative.
‘θ’ lies in 3rd quadrant.
∴ Principal argument = – \(\frac {3π}{4}\) [∵ \(\frac {π}{4}\) – π]
Question 17.
The principal argument of (sin 40° + i cos 40°)5 is:
(a) – 110°
(b) -70°
(c) 70°
(d) 110°
Solution:
(a) – 110°
Hint:
z = (sin 40° + i cos 40°)5
= (cos 50° + i sin 50°)5
= cos 250° + i sin 250°]
= cos (360° – 110°) + i sin (360° – 110°)
= cos 110° – i sin 110°
= cos (-110°) + i sin (-110°)
∴ Principal argument is – 110°
![]()
Question 18.
If (1 + i) (1 + 2i) (1 + 3i) ……. (l + ni) = x + iy, then 2.5.10 …… (1 + n²) is:
(a) 1
(b) i
(c) x² + y²
(d) 1 + n²
Solution:
(c) x² + y²
Hint:
(1 + i) (i + 2i) ….. (1 + ni) = x + iy
Taking of two modulli of each of squaring
2.5.10 … (1 +n²) = x²+ y²
Question 19.
If ω ≠ 1 is a cubic root of unity and (1 + ω)7 = A + Bω, then (A, B) equals:
(a) (1, 0)
(b) (-1, 1)
(c) (0, 1)
(d) (1, 1)
Solution:
(d) (1, 1)
Hint:
(1 + ω)7 = A + Bω
(- ω²)7 = A + Bω
– ω14 = A + Bω
– ω² = A + Bω
1 + ω² = A + Bω
∴ A = 1, B = 1
Question 20.
The principal argument of the complex number \(\frac{(1+i \sqrt{3})^{2}}{4 i(1-i \sqrt{3})}\) is:
(a) \(\frac{2π}{3}\)
(b) \(\frac{π}{6}\)
(c) \(\frac{5π}{6}\)
(d) \(\frac{π}{2}\)
Solution:
(d) \(\frac{π}{2}\)
Hint:
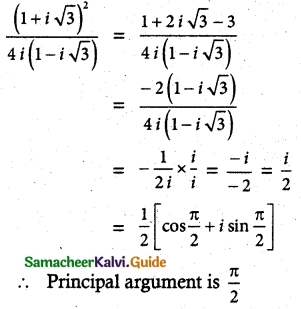
![]()
Question 21.
If α and β are the roots of x² + x + 1 = 0, then α2020 + β2020 is
(a) -2
(b) -1
(c) 1
(d) 2
Solution:
(b) -1
Hint:
x² + x + 1 = 0
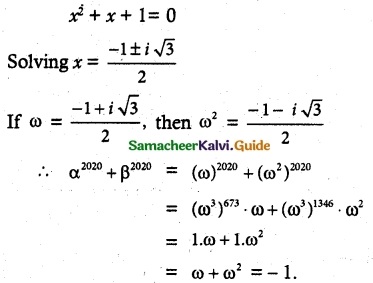
Question 22.
The product of all four values of (cos\(\frac{π}{3}\) + i sin \(\frac{π}{3}\))\(\frac{3}{4}\) is:
(a) -2
(b) -1
(c) 1
(d) 2
Solution:
(c) 1
Question 23.
If ω ≠ 1 is a cubic root of unity and

(a) 1
(b) -1
(c) √3 i
(d) -√3 i
Solution:
(d) -√3 i
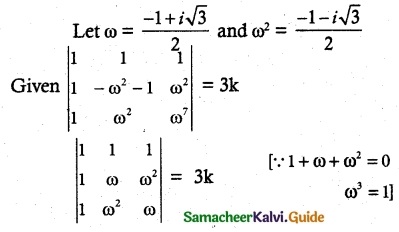
= 1(ω² – ω4) – (ω – ω²) + 1(ω² – ω) = 3k
= ω² – ω – ω + ω² + ω² – ω = 3k
= 3ω² – 3ω = 3k
= 3(ω² – ω) = 3k
∴ k = ω² – ω
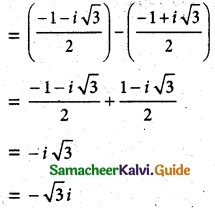
![]()
Question 24.
The value of (\(\frac{1+√3 i}{1-√3 i}\))10 is:
(a) cis\(\frac{2π}{3}\)
(b) cis\(\frac{4π}{3}\)
(c) -cis\(\frac{2π}{3}\)
(d) -cis\(\frac{2π}{3}\)
Solution:
(a) cis\(\frac{2π}{3}\)
Hint:
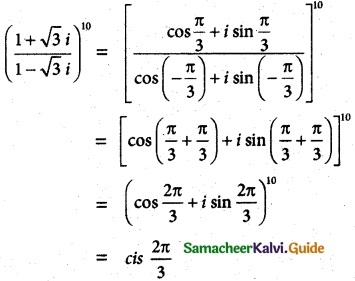
Question 25.
If ω = cis\(\frac{2π}{3}\), then the number of distinct roots of 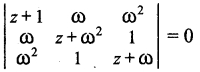
(a) 1
(b) 2
(c) 3
(d) 4
Solution:
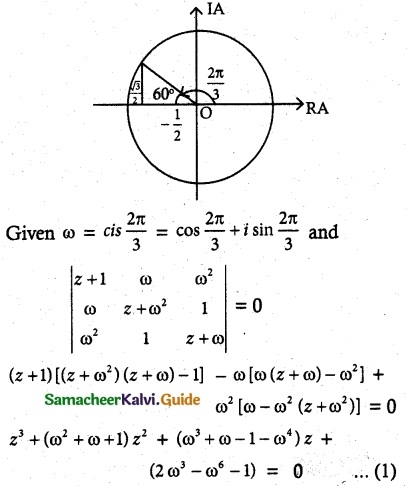
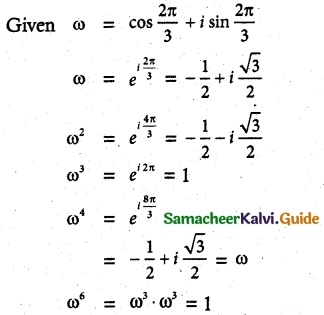
The expansion 1 becomes
z³ + (0) z² + (0) z + 0 = 0
⇒ z³ = 0
z = 0 is the only solution.
![]()