Tamil Nadu 11th Maths Model Question Paper 3 English Medium

Students can Download Tamil Nadu 11th Maths Model Question Paper 3 English Medium Pdf, Tamil Nadu 11th Maths Model Question Papers helps you to revise the complete Tamilnadu State Board New Syllabus, helps students complete homework assignments and to score high marks in board exams.

TN State Board 11th Maths Model Question Paper 3 English Medium

General Instructions:

1. The question paper comprises of four parts.
2. You are to attempt all the parts. An internal choice of questions is provided wherever applicable.
3. All questions of Part I, II, III and IV are to be attempted separately.
4. Question numbers 1 to 20 in Part I are Multiple Choice Questions of one mark each.
   These are to be answered by choosing the most suitable answer from the given four alternatives and writing the option code and the corresponding answer
5. Question numbers 21 to 30 in Part II are two-mark questions. These are to be answered in about one or two sentences.
6. Question numbers 31 to 40 in Part III are three-mark questions. These are to be answered in above three to five short sentences.
7. Question numbers 41 to 47 in Part IV are five-mark questions. These are to be answered in detail Draw diagrams wherever necessary.

Time: 2:30 Hours
Maximum Marks: 90

PART – 1

I. Choose the correct answer. Answer all the questions: [20 × 1 = 20]

Question 1.
Let R be the universal relation on a set X with more than one element then R is ………………
(a) Not reflexive
(b) Not symmetric
(c) Transitive
(d) None of the above
Answer:
(c) Transitive

Question 2.
The value of log_ab log_bc log_ca is …………………..
(a) 2
(b) 1
(c) 3
(d) 4
Answer:
(b) 1

Question 3.
If log \(\log _{\sqrt{ }}\) 0.25 = 4 then the value of x is ………………..
(a) 0.5
(b) 2.5
(c) 1.5
(d) 1.25
Answer:
(a) 0.5

Question 4.
The product of r consecutive positive integers is divisible by ………………..
(a) r!
(b) (r-1)!
(c) (r+l)!
(d) r^r
Answer:
(a) r!

Question 5.
The value of tan75° – cot 75° is ……………….
(a) 1
(b) 2 + \(\sqrt{3}\)
(c) 2 – \(\sqrt{3}\)
(d) 2\(\sqrt{3}\)
Answer:
(d) 2\(\sqrt{3}\)

Question 6.
If (1 +x²)²(1 + x)² = a₀ + a₁ x + a₂x² …. + xⁿ⁺⁴ and if a₀, a₁, a₂, are in AP, then n is …………………..
(a) 1
(b) 2
(c) 3
(d) 4
Answer:
(c) 3

Question 7.
If _nC₁₂ = _nC₅ then _nC₂ = …………………
(a) 72
(b) 306
(c) 152
(d) 153
Answer:
(d) 153

Question 8.
The line (p + 2q)x + (p- 3q)y =p – q for different values of p and q passes through the point …………………
(a) (\(\frac{3}{5}\), \(\frac{2}{5}\))
(b) (\(\frac{2}{5}\), \(\frac{2}{5}\))
(c) (\(\frac{3}{5}\), \(\frac{3}{5}\))
(d) (\(\frac{2}{5}\), \(\frac{3}{5}\))
Answer:
(d) (\(\frac{2}{5}\), \(\frac{3}{5}\))

Question 9.
The number of terms in the expansion of [(a + b)²]¹⁸ = ………………..
(a) 19
(b) 18
(c) 36
(d) 37
Answer:
(d) 37

Question 10.
A line passes through the point (2, 2) and is perpendicular to the line 3x + y = 5 then its y intercept is …………………
Answer:
(a) \(\frac{3}{4}\)
(b) \(\frac{4}{3}\)
(c) 5
(d) \(\frac{1}{3}\)

Question 11.
If a and b are the roots of the equation x² – kx + 16 = 0 satisfy a² + b² = 32, then the value of k is ………………..
(a) 10
(b) -8
(c) -8, 8
(d) 6
Answer:
(c) -8, 8

Question 12.
If A is a square matrix of order 3 then |kA| = ………………….
(a) k |A|
(b) k²|A|
(c) k³|A|
(d) k|A³|
Answer:
(c) k³|A|

Question 13.
If ABCD is a parallelogram then \(\bar { AB } \) + \(\bar { AD } \) + \(\bar { CD } \) + \(\bar { CD } \) = ………………..
(a) 2(\(\bar { AB } \) + \(\bar { AD } \))
(b) 4\(\bar { AC } \)
(c) 4\(\bar { BD } \)
(d) \(\bar { o } \)
Answer:
(d) \(\bar { o } \)

Question 14.
\(\lim _{x \rightarrow 0}\) x cot x = ………………….
(a) 0
(b) 1
(c) -1
(d) ∞
Answer:
(b) 1

Question 15.
If x = \(\frac { 1-t^{ 2 } }{ 1+t^{ 2 } } \) and y = \(\frac { 2t }{ 1+t^{ 2 } } \) then \(\frac{dy}{dx}\) = ………………..
(a) \(\frac{y}{x}\)
(b) \(\frac{-y}{x}\)
(c) –\(\frac{x}{y}\)
(d) \(\frac{x}{y}\)
Answer:
(c) –\(\frac{x}{y}\)

Question 16.
If y = \(\frac { (1-x)^{ 2 } }{ x^{ 2 } } \) then \(\frac{dy}{dx}\) is …………………..
(a) \(\frac { 2 }{ x^{ 2 } } \) + \(\frac { 2 }{ x^{ 3 } } \)
(b) –\(\frac { 2 }{ x^{ 2 } } \) + \(\frac { 2 }{ x^{ 3 } } \)
(c) –\(\frac { 2 }{ x^{ 2 } } \) – \(\frac { 2 }{ x^{ 3 } } \)
(d) –\(\frac { 2 }{ x^{ 3 } } \) + \(\frac { 2 }{ x^{ 2 } } \)
Answer:
(d) –\(\frac { 2 }{ x^{ 3 } } \) + \(\frac { 2 }{ x^{ 2 } } \)

Question 17.
If y = \(\frac{sinx+cosx}{sinx-cosx}\) then \(\frac{dy}{dx}\) at x = \(\frac { \pi }{ 2 } \) is ………………….
(a) 1
(b) 0
(c) -2
(d) 2
Answer:
(c) -2

Question 18.
\(\int \frac{\sin ^{8} x-\cos ^{8} x}{1-2 \sin ^{2} x \cos ^{2} x}\) dx is ……………………
(a) \(\frac{1}{2}\) sin2x + c
(b) –\(\frac{1}{2}\) sin2x + c
(c) \(\frac{1}{2}\) cos 2x + c
(d) – \(\frac{1}{2}\) cos 2x + c
Answer:
(b) –\(\frac{1}{2}\) sin2x + c

Question 19.
An urn contains 5 red and 5 black balls. A balls is drawn at random, its colour is noted and is returned to the urn. Moreover, 2 additional balls of the colour drawn are put in the urn and then a ball is drawn at random. The probability that the second ball drawn is red will be ………………
(a) \(\frac{5}{12}\)
(b) \(\frac{1}{2}\)
(c) \(\frac{7}{12}\)
(d) \(\frac{1}{4}\)
Answer:
(b) \(\frac{1}{2}\)

Question 20.
Let A and B be two events such that P(\(\bar { AUB } \)) = \(\frac{1}{6}\) , Then the events A and B are P(A∩B) = 1/4 and P(\(\bar { A } \)) = 1/4 is ………………
(a) Equally likely but not independent
(b) Independent but not equally likely
(c) Independent and equally likely
(d) Mutually inclusive and dependent
Answer:
(b) Independent but not equally likely

PART – II

II. Answer any seven questions. Question No. 30 is compulsory. [7 × 2 = 14]

Question 21.
Let A and B are two sets such that n(A) = 3 and n(B) = 2. If (x, 1), (y, 2) and (z, 1) are in A × B, find A and B where x, y, z are distinct elements?
Answer:
n(A) = 3 ⇒ set A contains 3 elements
n(B) = 2 ⇒ set B contains 2 elements
we are given (x, 1), (y, 2), (z, 1) are elements in A × B
⇒ A = {x, y, z} and B = {1, 2}

Question 22.
Solve |5x — 12| ← 2
Answer:
5x – 12 > -2 (or) 5x – 12 < 2 ⇒ 5x > -2 + 12 (= 10)
⇒ x > \(\frac{10}{5}\) = 2
x > 2
(or)
5x < 2 + 12 (= 14)
⇒ x < \(\frac{14}{5}\)
so 2 < x < \(\frac{14}{5}\)

Question 23.
If ¹⁰P_r-1 = 2 × 6 P_r, find r?
Answer:
¹⁰P_r-1 = 2 × 6P_r

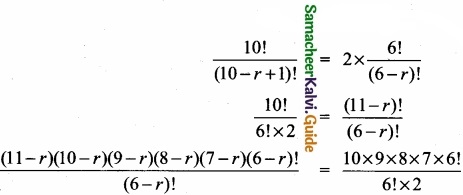
⇒ (11 – r) (10 – r) (9 – r) (8 – r) (7 – r) = 10 × 9 × 4 × 7
= 5 × 2 × 3 × 3 × 2 × 2 × 7
= 7 × 6 × 5 × 4 × 3
⇒ 11 – r = 7
11 – 7 = r
r = 4

Question 24.
The number of bacteria in a certain culture doubles every hour. If there were 30 bacteria present in the culture originally, how many bacteria will be present at the end of 2^nd hour, 4^th hour and n^th hour?
Answer:
No. of bacteria at the beginning = 30
No. of bacteria after 1 hour = 30 × 2 = 60
No. of bacteria after 2 hours = 30 × 2² = 30 × 4 = 120
No. of bacteria after 4 hours = 30 × 2⁴ = 30 × 16 = 480
No. of bacteria after n^th hour = 30 × 2ⁿ

Question 25.
Find |A| if A = \(\left[\begin{array}{ccc}
0 & \sin \alpha & \cos \alpha \\
\sin \alpha & 0 & \sin \beta \\
\cos \alpha & -\sin \beta & 0
\end{array}\right]\)
Answer:
\(\left[\begin{array}{ccc}
0 & \sin \alpha & \cos \alpha \\
\sin \alpha & 0 & \sin \beta \\
\cos \alpha & -\sin \beta & 0
\end{array}\right]\)
= 0M₁₁ – sin αM₁₂ + cos αM₁₃
= 0 – sin α(0 – cos α sin β) + cos α(- sin α sin β – 0) = 0

Question 26.
Find the value of λ for which the vectors \(\vec { a } \) = 3\(\hat { i } \) + 2\(\hat { j } \) + 9\(\hat { k } \) and \(\vec { a } \) = \(\hat { i } \) + λ\(\hat { j } \) +3\(\hat { k } \) are parallel?
Answer:
Given \(\vec { a } \) and \(\vec { b } \) are parallel ⇒\(\vec { a } \) = t\(\vec { b } \) (where t is a scalar)
(i.e.,) 3\(\hat { i } \) + 2\(\hat { j } \) + 9\(\hat { k } \) = t\(\hat { i } \) + λ\(\hat { j } \) + 3\(\hat { k } \))
equating \(\hat { i } \) components we get 3 = t
equating \(\hat { j } \) components
(i.e); 2 = tλ
2 = 3λ ⇒λ = 2/3

Question 27.
Evaluate \(\underset { x\rightarrow \pi }{ lim } \) \(\frac{sin 3x}{sin 2x}\)
Answer:

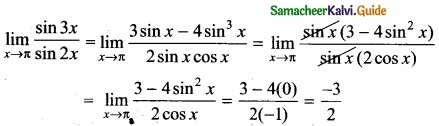
Question 28.
Find the derivative of sinx² with respect to x²?
Answer:

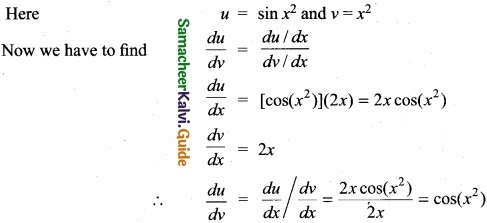
Question 29.
Let the matrix M = \(\begin{bmatrix} x & y \\ z & 1 \end{bmatrix}\) if x, y and z are chosen at random from the set {1, 2, 3}, and repetition is allowed (i.e., x = y = z), what is the probability that the given matrix M is a singular matrix?
Answer:
If the given matnx M is singular, then = \(\begin{vmatrix} x & y \\ z & 1 \end{vmatrix}\) = 0
That is, x – yz = 0
Hence the possible ways of selecting (x, y, z) are
{(1, 1, 1), (2, 1, 2), (2, 2, 1), (3, 1, 3), (3, 3, 1)} = A (say)
The number of favourable cases n(A) = 5
The total number of cases are n(S) = 3³ = 27
The probability of the given matrix is a singular matrix is
P(A) = \(\frac{n(A)}{n(S)}\) = \(\frac{5}{27}\)

Question 30.
Evaluate \(\frac { x^{ 2 } }{ 1+x^{ 6 } } \)
Answer:

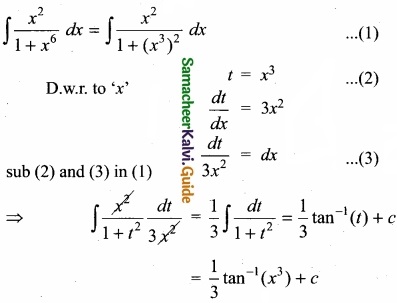
PART – III

III. Answer any seven questions. Question No. 40 is compulsory. [7 × 3 = 21]

Question 31.
If f, g, h are real valued functions defined on R, then prove that (f + g) o h = f o h + g o h. What can you say about fo(g + h)? Justify your answer?

Question 32.
Solve \(\frac{4}{x+1}\) ≤ 3 ≤ \(\frac{6}{x+1}\), x > 0?

Question 33.
Prove that cos^-1 \(\frac{4}{5}\) + tan^-1 \(\frac{3}{5}\) = tan^-1 \(\frac{27}{11}\)?

Question 34.
There are 15 candidates for an examination. 7 candidates are appearing for mathematics examination while the remaining 8 are appearing for different subjects. In how many ways
can they be seated in a row so that no two mathematics candidates are together?

Question 35.
Prove that if a, b, c are in H.P. if and only if \(\frac{a}{c}\) = \(\frac{a-b}{b-c}\)?

Question 36.
If (-4, 7) is one vertex of a rhombus and if the equation of one diagonal is 5x – y + 7 = 0, then find the equation of another diagonal?

Question 37.
Verify the existence of \(\underset { x\rightarrow 1 }{ lim } \) f(x), where f(x) = \(\left\{\begin{aligned}
\frac{|x-1|}{x-1}, & \text { for } x \neq 1 \\
0, & \text { for } x=1
\end{aligned}\right.\)

Question 38.
If y = sin^-1 x then find y?

Question 39.
Evaluate cot² x + tan² x?

Question 40.
Show that
\(\left|\begin{array}{ccc}
2 b c-a^{2} & c^{2} & b^{2} \\
c^{2} & 2 c a-b^{2} & a^{2} \\
b^{2} & a^{2} & 2 a b-c^{2}
\end{array}\right|=\left|\begin{array}{ccc}
a & b & c \\
b & c & a \\
c & a & b
\end{array}\right|^{2}\)

PART – IV

IV. Answer all the questions. [7 × 5 = 35]

Question 41 (a).
The total cost of airfare on a given route is comprised of the base cost C and the fuel surcharge S in rupee. Both C and S are functions of the mileage m; C(m) = 0.4m + 50 and S(m) = 0.03m. Determine a function for the total cost of a ticket in terms of the mileage and find the airfare for flying ¡600 miles?

[OR]

(b) Evaluate \(\sqrt { x^{ 2 }+x+1 } \)?

Question 42 (a).
Determine the region in the plane determined by the inequalities y ≥ 2x and -2x + 3y ≤ 6?

[OR]

(b) If y(cos^-1 x)², prove that (1-x²) \(\frac { d^{ 2 }y }{ dx^{ 2 } } \) – x \(\frac{dy}{dx}\) – 2 = 0. Hence find y₂ when x = 0?

Question 43(a).
Prove that ⁿC_r + ⁿC_r-1 = ⁿ⁺¹C_r

[OR]

(b) If the binomial coefficients of three consecutive terms in the expansion of (a + x)ⁿ are in the ratio 1 : 7 : 42, then find n?

Question 44 (a).
(a) Prove that

1. sin A + sin( 120° + A) + sin (240° + A) = O
2. cos A+ cos (120° + A) + cos (120° – A) = O

[OR]

(b) A box contains two white balls, three black balls and four red balls. In how many ways can three balls be drawn from the box, if at least one black ball is to be included in the draw?

Question 45 (a).
Show that \(\left|\begin{array}{ccc}
a^{2}+x^{2} & a b & a c \\
a b & b^{2}+x^{2} & b c \\
a c & b c & c^{2}+x^{2}
\end{array}\right|\) is divisible by x⁴?

[OR]

(b) \(\left[\begin{array}{ccc}
0 & p & 3 \\
2 & q^{2} & -1 \\
r & 1 & 0
\end{array}\right]\) is skew-symmetric, find the values of p, q and r?

Question 46 (a).
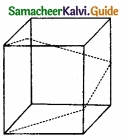
In a shopping mall there is a hail of cuboid shape with dimension 800 × 800 × 720 units, which needs to be added the facility of an escalator in the path as shown by the dotted line in the figure. Find

1. The minimum total length of the escalator
2. The heights at which the escalator changes its direction
3. The slopes of the escalator at the turning points.

[OR]

(b) Evaluate \(\lim _{x \rightarrow a} \frac{\sqrt{x-b}-\sqrt{a-b}}{x^{2}-a^{2}}(a>b)\)

Question 47 (a).
Evaluate ∫\(\frac { 3x+5 }{ x^{ 2 }+4x+7 } \) dx

[OR]

(b) A factory has two Machines – I and II. Machine-I produces 60% of items and Machine-II produces 40% of the items of the total output. Further 2% of the items produced by Machine-I are defective whereas 4% produced by Machine-II are defective. If an itci is drawn at random what is the probability that it is defective?