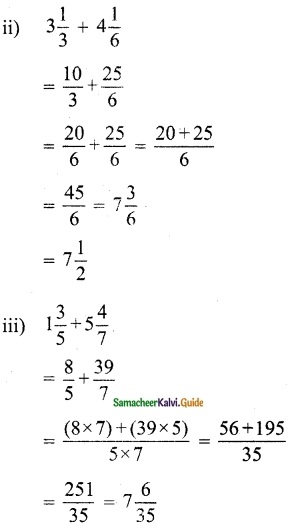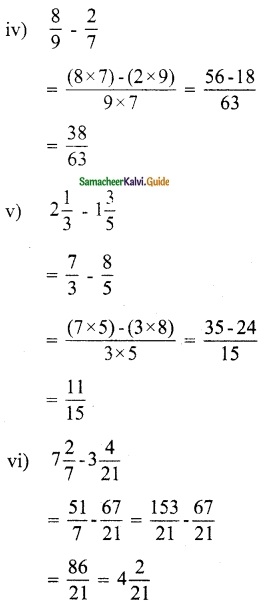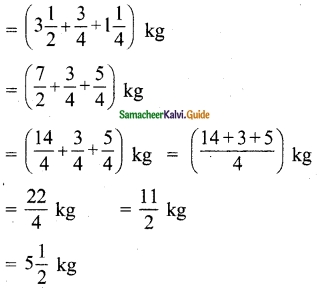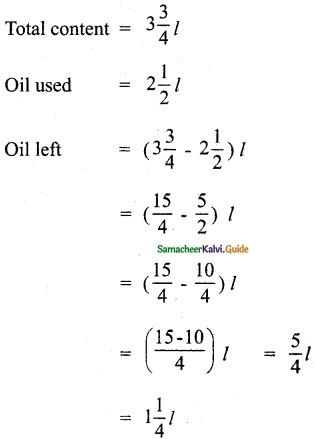Category: Class 6

Students can download Maths Chapter 3 Bill, Profit and Loss Ex 3.2 Questions and Answers, Notes, Samacheer Kalvi 6th Maths Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus, helps students complete homework assignments and to score high marks in board exams.

ASTRAL Pivot Point Calculator

Tamilnadu Samacheer Kalvi 6th Maths Solutions Term 2 Chapter 3 Bill, Profit and Loss Ex 3.2

Miscellaneous Practice Problems

Question 1.
A Shopkeeper buys three articles for Rs 325, Rs 450, and Rs 510. He is able to sell them for Rs 350, Rs 425, and Rs 525 respectively. Find the gain or loss to the shopkeeper on the whole.
Solution:
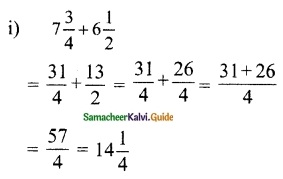
C.P of three articles = 325 + 450 + 510 = ₹ 1285
S.P of three articles = 350 + 425 + 525 = ₹ 1,300
Here S.P > C.P
Profit = S.P – C.P = 1,300 – 1285 = ₹ 15
The shopkeeper gained = ₹ 15

Question 2.
A stationery shop owner bought a scientific calculator for ₹ 750. He had put a battery worth ₹ 100 in it. He had spent ₹ 50 for its outer pouch. He was able to sell it at ₹ 850. Find his profit or loss.
Solution:
Cost of the scientific calculator = ₹ 750
Cost of its battery = ₹ 100
Cost of outer pouch = ₹ 50
Cost Price of the calculator = ₹ 750 + ₹ 100 + ₹ 50 = ₹ 900
S.P = ₹ 850
Here S.P > C.P
Loss = C.P – S.P = 900 – 850 = ₹ 50
Loss = ₹ 50

Question 3.
Nathan paid Rs 800 and bought 10 bottles of honey from a village vendor. He sold them in a gain for Rs 100 per bottle. Find his profit or loss.
Solution:
C.P of 10 bottles of honey = ₹ 800
C.P of 1 bottle honey = 800/10 = ₹ 80
S.P of a bottle honey = ₹ 100
Here S.P > C.P
Profit per bottle = ₹ 100 – ₹ 80 = ₹ 20
Profit for 10 bottles = 20 × 10 = ₹ 200
Profit = ₹ 200

Question 4.
A man bought 400 metres of cloth for ₹ 60,000 and sold it at the rate of ₹ 400 per metre. Find his profit or loss.
Solution:
C.P of 400 metres of cloth = ₹ 60,000
S.P per metre = ₹ 400
S.P of 400 metres of cloth = 400 × 400 = ₹ 1,60,000
Here S.P > C.P
Profit = C.P – S.P = 1,60,000 – 60,000 = ₹ 1,00,000

Challenge Problems

Question 5.
A fruit seller bought 2 dozen bananas at Rs 20 a dozen and sold them at Rs 3 per banana. Find his gain or loss.
Solution:
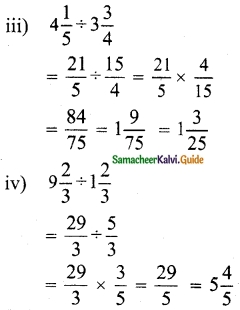
Cost of one dozen banana = ₹ 20
Cost of 2 dozen bananas = ₹ 20 × 2 = ₹ 40
C.P = ₹ 40
S.P per banana = ₹ 3
S.P for 2 dozen banana = ₹ 3 × 24 = ₹ 72
Here S.P > C.P
Profit = S.P – C.P = 72 – 40 = 32
Profit = ₹ 32

Question 6.
A store purchased pens at ₹ 216 per dozen. He paid ₹ 58 for conveyance and sold the pens at the discount of n per pen and made an overall profit of ₹ 50. Find the M.P of each pen.
Solution:
Cost of a dozen pens = ₹ 216
Paid for conveyance = ₹ 58
Cost price of 12 pens = 216 + 58 = ₹ 274 [∵ 1 dozen = 12]
Profit of 12 pens = ₹ 50
Profit = S.P – C.P
⇒ 50 = S.P – 274
⇒ S.P = 50 + 274 = ₹ 324
Also discount allowed per pen = ₹ 2
Discount for 12 pens = 2 × 12 = ₹ 24
S.P = M.P – Discount
⇒ 324 = M.P – 24
⇒ M.P = 324 + 24 = ₹ 348
Marked price for 12 pens = ₹ 348
M.P of a pen = \(\frac { 348 }{ 12 }\) = ₹ 29
M.P per pen = ₹ 29

Question 7.
A Vegetable vendor buys 10 kg of tomatoes per day at Rs 10 per kg, for the first three days of a week. 1 kg of tomatoes got smashed every day for those 3 days. For the remaining 4 days of the week, he buys 15 kg of tomatoes daily per kg. If for the entire week he sells tomatoes at Rs 20 per kg, then find his profit or loss for the week.
Solution:
Total tomatoes bought for 3 days = 3 × 10 = 30 kg
Cost of 1 kg = ₹ 10
Cost of 30kg tomatoes = 30 × 10 = ₹ 300
Total tomatoes bought for other 4 days = 4 × 15 = 60 kg
Cost of 1 kg = ₹ 8
Cost of 60 kg tomatoes = 60 × 8 = ₹ 480
Total cost of 90 kg tomatoes = 300 + 480 = ₹ 780
C.P = ₹ 780
Tomatoes smashed = 3 kg
Total kg of Tomatoes for sale = 90 – 3 = 87 kg
S.P of 1 kg tomatoes = ₹ 20
S.P of 87 kg tomatoes = 87 × 20 = ₹ 1740
Here S.P > C.P
Profit = S.P – C.P = 1740 – 780 = ₹ 960
Profit = ₹ 960

Question 8.
An electrician buys a used T.V for ₹ 12,000 and a used Fridge for ₹ 11,000. After spending ₹ 1000 on repairing the T.V and ₹ 1500 on painting the Fridge, he fixes up the M.P of T.V as ₹ 15,000 and that of the Fridge as ₹ 15,500. If he gives each ₹ 1000 discount oh each find his profit or loss.
Solution:
(i) Cost of a T.V = ₹ 12,000
Paid for repair = ₹ 1,000
C.P of the T.V = 12,000 + 1000 = ₹ 13,000
M.P of the T.V = ₹ 15,000
Discount on a TV = ₹ 1000
S.P = M.P – Discount = 15,000 – 1000 = ₹ 14,000
Here S.P > C.P
Profit = S.P – C.P = 14,000 – 13,000 = ₹ 1,000
Profit on the T.V = ₹ 1,000
(ii) Cost of the Fridge = ₹ 11,000
Painting charge = ₹ 1500
C.P of the Fridge = 11000 + 1500 = ₹ 12,500
M.P of the Fridge = ₹ 15,500
Discount allowed = ₹ 1000
S.P = M.P – Discount = ₹ 15,500 – ₹ 1000 = ₹ 14,500
Here also S.P > C.P
Profit = ₹ 14,500 – ₹ 12,500 = ₹ 2000
Total profit = Profit on T.V + Profit on Fridge = ₹ 1000 + ₹ 2000 = ₹ 3000
Profit = ₹ 3000

Students can download Maths Chapter 2 Integers Ex 2.1 Questions and Answers, Notes, Samacheer Kalvi 6th Maths Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus, helps students complete homework assignments and to score high marks in board exams.

Tamilnadu Samacheer Kalvi 6th Maths Solutions Term 3 Chapter 2 Integers Ex 2.1

Question 1.
Fill in the blanks:
(i) The potable water available at 100 m below the ground level is denoted as ……… m.
(ii) A swimmer dives to a depth of 7 feet from the ground into the swimming pool. The integer that represents this, is ……… feet.
(iii) -46 is to the ……….. of -35 on the number line.
(iv) There are ……… integers from -5 to +5 (both inclusive)
(v) …….. is an integer which is neither positive nor negative.
Solution:
(i) 100
(ii) -7
(iii) left
(iv) 11
(v) 0

Question 2.
Say True or False
(i) Each of the integers -18, 6, -12, 0 is greater than -20.
(ii) -1 is to the right of 0.
(iii) -10 and 10 are at equal distance from 1.
(iv) All negative integers are greater than zero.
(v) All whole numbers are integers.
Solution:
(i) True
(ii) False
(iii) False
(iv) False
(v) True

Question 3.
Mark the numbers 4, -3, 6, -1 and -5 on the number line.
Solution:

Question 4.
On the number line, which number is
(i) 4 units to the right of -7?
(ii) 5 units to the left of 3?
Solution:
(i) -3
(ii) -2

Question 5.
Find the opposite of the following numbers.
(i) 44
(ii) -19
(iii) 0
(iv) -312
(v) 789
Solution:
(i) Opposite of 44 is – 44
(ii) Opposite of-19 is + 19 or 19
(iii) Opposite of 0 is 0
(iv) Opposite of-312 is + 312 or 312
(v) Opposite of 789 is – 789.

Question 6.
If 15 km east of a place is denoted as +15 km, What is the integer that represents 15 km west of it?
Solution:
Opposite of east is west.
∴ If 15 km east is + 15 km, then 15 km west is – 15 km.

Question 7.
From the following number lines, identify the correct and the wrong representations with reason.

Solution:
(i) Wrong, Integers are not continuously marked
(ii) Correct, Integers are correctly marked.
(iii) Wrong, Integer -2 is marked wrongly.
(iv) Correct, Integers are marked at equal distance.
(v) Wrong, negative integers marked wrongly.

Question 8.
Write all the integers between the given numbers.
(i) 7 and 10
(ii) -5 and 4
(iii) -3 and 3
(iv) -5 and 0
Solution:
(i) 8, 9
(ii) -4, -3, -2, -1, 0, 1, 2, 3
(iii) -2, -1, 0, 1, 2
(iv) -4, -3, -2, -1

Question 9.
Put the appropriate signs as <, > or = in the blank.
(i) -7 ___ 8
(ii) -8 ___ -7
(iii) -999 ___ -1000
(iv) 0 ___ -200
Solution:
(i) <
(ii) <
(iii) >
(iv) =
(v) >

Question 10.
Arrange the following integers in ascending order.
(i) -11, 12, -13, 14, -15, 16, -17, 18, -19, -20
(ii) -28, 6, -5, -40, 8, 0, 12, -1, 4, 22
(iii) -100, 10, -1000, 100, 0, -1, 1000, 1, -10
Solution:

(i) -11, 12, -13, 14, -15, 16, -17, 18, -19, -20

• First separating the positive integers 12, 14, 16, 18 and the negative integers -11,-13,-15,-17,-19,-20.
• Then arranging the positive integers in ascending order we get 12, 14, 16, 18 and negative integers in ascending order as -20, -19, -17, -15, -13, -11 4
• Now the ascending order : -20, -19, -17, -15, -13, -11, 12, 14, 16, 18.

(ii) -28, 6, -5, -40, 8, 0, 12, -1, 4, 22

• Positive integers are 6, 8, 12, 4, 22 Negative integers are -28, -5, -40, -1
• Arranging the positive integers in ascending order we get 4, 6, 8, 12, 22 and the negative integers in ascending order -40, -28, -5, -1
• The number 0 is neither positive nor negative and stands in the middle.
• In ascending order : -40, -28, -5, -1, 0, 4, 6, 8,12, 22

(iii) -100, 10, -1000, 100, 0, -1, 1000, 1, -10

• Separating positive integers 10, 100, 1000, 1 and negative integers -100, -1000, -1, -10.
• Now the positive integers in ascending order 1,10,100,1000 and the negative integers in ascending order. -1000, -100, -10, -1
• Also ‘0’ stand in the middle as its is neither positive nor negative.
• ∴ The numbers in ascending order: -1000, -100, -10, -1, 0, 1, 10, 100, 1000.

Question 11.
Arrange the following integers in descending order.
(i) 14, 27, 15, -14, -9, 0, 11, -17
(ii) -99, -120, 65, -46, 78, 400, -600
(iii) 111, -222, 333, -444, 555, -666, 777, -888
Solution:
(i) 27, 15, 14, 11, 0, -9, -14, -17
(ii) 400, 78, 65, -46, -99, -120, -600
(iii) 777, 555, 333, 111, -222, -444, -666, -888

Objective Type Questions

Question 12.
There are ……… positive integers from -5 to 6.
(a) 5
(b) 6
(c) 7
(d) 11
Solution:
(c) 7

Question 13.
The opposite of 20 units to the left of 0 is
(a) 20
(b) 0
(c) -20
(d) 40
Solution:
(a) 20

Question 14.
One unit to the right of -7 is
(a) +1
(b) -8
(c) -7
(d) -6
Solution:
(d) -6

Question 15.
3 units to the left of 1 is
(a) -4
(b) -3
(c) -2
(d) 3
Solution:
(c) -2

Question 16.
The number which determines marking the position of any number to its opposite on a number line is
(a) -1
(b) 0
(c) 1
(d) 10
Solution:
(b) 0

Students can download Maths Chapter 1 Fractions Ex 1.2 Questions and Answers, Notes, Samacheer Kalvi 6th Maths Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus, helps students complete homework assignments and to score high marks in board exams.

Tamilnadu Samacheer Kalvi 6th Maths Solutions Term 3 Chapter 1 Fractions Ex 1.2

Miscellaneous Practice Problems

Question 1.
Sankari purchased 2\(\frac{1}{2}\) m cloth to stich a long skirt and 1\(\frac{3}{4}\) m cloth to stitch blouse. If the cost is Rs. 120 per metre then find the cost of cloth purchased by her.
Solution:
Total cloth purchased

cost of 1 metre = Rs. 120
Total cost of cloth purchased
= Rs. 120 × \(\frac{17}{4}\)
= Rs. 510

Question 2.
From his office, a person wants to reach his house on foot which is at a distance of 5\(\frac{3}{4}\) km. If he had walked 2\(\frac{1}{2}\) km, how much distance still he has to walk to reach his house?
Solution:

Question 3.
Which is smaller? The difference between 2\(\frac{1}{2}\) and 3\(\frac{2}{3}\) or the sum of 1\(\frac{1}{2}\) and 2\(\frac{1}{4}\).
Solution:

∴ The difference of 2\(\frac{1}{2}\) and 3\(\frac{2}{3}\) is smaller

Question 4.
Mangai bought 6\(\frac{3}{4}\) kg of apples. If Kalai 1 bought 1\(\frac{1}{2}\) times a Mangai bought, then how many kilograms of apples did Kalai buy?
Solution:
Apples bought by Mangai = 6\(\frac{3}{4}\) kg
Apples bought by Kalai

Question 5.
The length of the staircase is 5\(\frac{1}{2}\) m. If one step is set at \(\frac{1}{4}\) m, then how many steps will be there in the staircase?

Solution:
Total length of the staircase = 5\(\frac{1}{2}\) m
length of each step = \(\frac{1}{4}\) m
No of steps in the stair case

= 22 steps

Challenge Problems

Question 6.
By using the following clues, find who am I?
(i) Each of my numerator and denominator is a single-digit number.
(ii) The sum of my numerator and denominator is a multiple of 3.
(iii) The product of my numerator and denominator is a multiple of 4.
Solution:
The numerator may be any one of!, 2, 3,4, 5, 6, 7, 8, 9 and the denominator may be any one of 1, 2,3,4, 5,6, 7,8,9. Sum of numerator and denominator is a multiple of 3.
∴ Possible proper fractions are \(\frac{1}{2}, \frac{1}{5}, \frac{1}{8}, \frac{2}{4}, \frac{2}{7}, \frac{3}{6}, \frac{3}{9}, \frac{4}{5}, \frac{4}{8}, \frac{5}{7}, \frac{6}{9}\)
Also given the product of numerator and denominator is a multiple of 4.
∴ Possible fractions are \(\frac{1}{8}, \frac{2}{4}, \frac{4}{5}, \frac{4}{8}\)

Question 7.
Add the difference between 1\(\frac{1}{3}\) and 3\(\frac{1}{6}\) and the difference between 4\(\frac{1}{6}\) and 2\(\frac{1}{3}\)
Solution:

Adding Difference

Question 8.
What fraction is to be subtracted from 9\(\frac{3}{7}\) to get 3\(\frac{1}{5}\)?
Solution:
Let the fraction be x
According to the problem

The fraction to be subtracted is 6\(\frac{8}{35}\)

Question 9.
The sum of two fractions is 5\(\frac{3}{9}\). If one of the fractions is 2\(\frac{3}{4}\), find the other fraction.
Solution:
Let the other fraction be x
According to the problem,

∴ The other fraction is 2\(\frac{7}{12}\)

Question 10.
By what number should 3\(\frac{1}{16}\) be multiplied to get 9\(\frac{3}{16}\)?
Solution:
Let the number be x
According to the problem,

x = 3
The number is 3

Question 11.
Complete the fifth row in the Leibnitz triangle which is based on subtraction.

Solution:

Question 12.
A painter painted \(\frac{3}{8}\) of the wall of which one third is painted in yellow colour. What fraction is the yellow colour of the entire wall?

Solution:
yellow colour of the entire wall
= \(\frac{3}{8}\) × \(\frac{1}{3}\)
= \(\frac{1}{8}\)

Question 13.
A rabbit has to cover 26\(\frac{1}{4}\) m to fetch its food. If it covers 1\(\frac{3}{4}\) m in one jump, then how many jumps will it take to fetch its food?

Solution:
Total distance = 26\(\frac{1}{4}\) m
Distance covered in one jump = 1\(\frac{3}{4}\) m
Number of jumps required to fetch the food

= 15

Question 14.
Look at the picture and answer the following questions.

(i) What is the distance from the school to Library via Bus stop?
(ii) What is the distance between School and Library via Hospital?
(iii) Which is the shortest distance between (i) and (ii)?
(iv) The distance between School and Hospital is times the distance between School and Bus stop.
Solution:


(iii) Via bus stop
(iv) 6 times (6 × \(\frac{3}{4}\) = \(\frac{18}{4}\) = \(\frac{9}{2}\) = 4\(\frac{1}{2}\))

Students can download Maths Chapter 1 Fractions Ex 1.1 Questions and Answers, Notes, Samacheer Kalvi 6th Maths Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus, helps students complete homework assignments and to score high marks in board exams.

Tamilnadu Samacheer Kalvi 6th Maths Solutions Term 3 Chapter 1 Fractions Ex 1.1

Question 1.
Fill in the blanks:
(i) 7\(\frac{3}{4}\) + 6\(\frac{1}{2}\) ………..
(ii) The sum of a whole number and a proper fraction is called ……….
(iii) 5\(\frac{1}{3}\) – 3\(\frac{1}{2}\) ………..
(iv) 8 ÷ \(\frac{1}{2}\) ………..
(v) The number which has its own reciprocal is ……….
Solution:

(ii) Mixed Fraction

(iii) 5\(\frac{1}{3}\) – 3\(\frac{1}{2}\)
= \(\frac{16}{3}\) – \(\frac{7}{2}\) = \(\frac{32-21}{6}\) = \(\frac{11}{6}\)
= 1\(\frac{5}{6}\)

(iv) 8 ÷ \(\frac{1}{2}\)
= 8 × \(\frac{2}{1}\)
= 16

(v) 1

Question 2.
Say True or False
(i) 3\(\frac{1}{2}\) can be written as 3 + \(\frac{1}{2}\).
(ii) The sum of any two proper fractions is always an improper fraction.
(iii) The mixed fraction of \(\frac{13}{4}\) is 3\(\frac{1}{4}\).
(iv) The reciprocal of an improper fraction is always a proper fraction.
(v) 3\(\frac{1}{4}\) × 3\(\frac{1}{4}\) = 9\(\frac{1}{16}\)
Solution:
(i) True
(ii) False
(iii) True
(iv) True
(v) False

Question 3.
Answer the following:
(i) Find the sum of \(\frac{1}{7}\) and \(\frac{3}{9}\).
(ii) What is the total of 3\(\frac{1}{3}\) and 4\(\frac{1}{6}\)?
(iii) Simplify: 1\(\frac{3}{5}\)+5\(\frac{4}{7}\).
(iv) Find the difference between \(\frac{8}{9}\) and \(\frac{2}{7}\)
(v) Subtract: 1\(\frac{3}{5}\) from 2\(\frac{1}{3}\).
(vi) Simplify: 7\(\frac{2}{7}\) – 3\(\frac{4}{21}\)
Solution:
(i) \(\frac{1}{7}\) + \(\frac{3}{9}\)
= \(\frac{9+21}{63}\) = \(\frac{30}{63}\) = \(\frac{10}{21}\)

Question 4.
Convert mixed fractions into improper fractions and vice versa:
(i) 3\(\frac{7}{18}\)
(ii) \(\frac{99}{7}\)
(iii) \(\frac{47}{6}\)
(iv) 12\(\frac{1}{9}\)
Solution:
(i) 3\(\frac{7}{18}\) = \(\frac{61}{18}\)
(ii) \(\frac{99}{7}\) = 14\(\frac{1}{7}\)
(iii) \(\frac{47}{6}\) = 7\(\frac{5}{6}\)
(iv) 12\(\frac{1}{9}\) = \(\frac{109}{9}\)

Question 5.
Multiply the following:
(i) \(\frac{2}{3}\) × 6
(ii) 8\(\frac{1}{3}\) × 5
(iii) \(\frac{3}{8}\) × \(\frac{4}{5}\)
(iv) 3\(\frac{5}{7}\) × 1\(\frac{1}{13}\)
Solution:
(i) \(\frac{2}{3}\) × 6 = 4

(ii) 8\(\frac{1}{3}\) × 5
= \(\frac{25}{3}\) × 5
= \(\frac{125}{3}\)
= 41\(\frac{2}{3}\)

(iii) \(\frac{3}{8}\) × \(\frac{4}{5}\)
= \(\frac{12}{40}\) = \(\frac{3}{10}\)

(iv) 3\(\frac{5}{7}\) × 1\(\frac{1}{13}\)
= \(\frac{26}{7}\) × \(\frac{14}{13}\)
= \(\frac{4}{1}\)
= 4

Question 6.
Divide the following
(i) \(\frac{3}{7}\) ÷ 4
(ii) \(\frac{4}{3}\) ÷ \(\frac{5}{9}\)
(iii) 4\(\frac{1}{5}\) ÷ 3\(\frac{3}{4}\)
(iv) 9\(\frac{2}{3}\) ÷ 1\(\frac{2}{3}\)
Solution:
(i) \(\frac{3}{7}\) ÷ 4
= \(\frac{3}{7}\) × \(\frac{1}{4}\) = \(\frac{3}{28}\)

(ii) \(\frac{4}{3}\) ÷ \(\frac{5}{9}\)
= \(\frac{4}{3}\) × \(\frac{9}{5}\)
= \(\frac{12}{5}\)
= 2\(\frac{2}{5}\)

Question 7.
Gowri purchased 3\(\frac{1}{2}\) kg of tomatoes, \(\frac{3}{4}\) kg of brinjal and 1\(\frac{1}{4}\) kg of onion. What is the total weight of the vegetables she bought?
Solution:
Total weight of vegetables bought

Question 8.
An oil tin contains 3\(\frac{3}{4}\) litres of oil of which 2\(\frac{1}{2}\) litres of oil is used. How much oil is left over?
Solution:

Question 9.
Nilavan can walk 4\(\frac{1}{2}\) km in an hour. How much distance will he cover in 3\(\frac{1}{2}\) hours?
Solution:
Distance walked in an hour = 4\(\frac{1}{2}\) km
Distance covered in 3\(\frac{1}{2}\)
Hours

Question 10.
Ravi bought a curtain of length 15\(\frac{3}{4}\) m. If he cut the curtain into small pieces each of length 2\(\frac{1}{4}\) m, then how many small curtains will he get?
Solution:
Total length = 15\(\frac{3}{4}\)
Length of the small pieces = 2\(\frac{1}{4}\)
Small curtains obtained

= 7

Objective Type Questions

Question 11.
Which of the following statement is incorrect?
(a) \(\frac{1}{2}\) > \(\frac{1}{3}\)
(b) \(\frac{7}{8}\) > \(\frac{6}{7}\)
(c) \(\frac{8}{9}\) < \(\frac{9}{10}\)
(d) \(\frac{10}{11}\) > \(\frac{9}{10}\)
Solution:
(d) \(\frac{10}{11}\) > \(\frac{9}{10}\)

Question 12.
The difference between \(\frac{3}{7}\) and \(\frac{2}{9}\) is
(a) \(\frac{13}{63}\)
(b) \(\frac{1}{9}\)
(c) \(\frac{1}{7}\)
(d) \(\frac{9}{16}\)
Solution:
(a) \(\frac{13}{63}\)

Question 13.
The reciprocal of \(\frac{53}{17}\) is
(a) \(\frac{53}{17}\)
(b) 5\(\frac{3}{17}\)
(c) \(\frac{17}{53}\)
(d) 3\(\frac{5}{17}\)
Solution:
(c) \(\frac{17}{53}\)

Question 14.
If \(\frac{6}{7}=\frac{\mathbf{A}}{49}\), then the value of A is
(a) 42
(b) 36
(c) 25
(d) 48
Solution:
(a) 42
Hint: \(\frac{6 \times 49}{7}=42\)

Question 15.
Pugazh has been given four choices for his pocket money by his father. Which of the choices should he take in order to get the maximum money?
(a) \(\frac{2}{3}\) of Rs 150
(b) \(\frac{3}{5}\) of Rs 150
(c) \(\frac{4}{5}\) of Rs 150
(d) \(\frac{1}{5}\) of Rs 150
Solution:
(c) \(\frac{4}{5}\) of Rs 150

Students can download Maths Chapter 4 Geometry Ex 4.4 Questions and Answers, Notes, Samacheer Kalvi 6th Maths Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus, helps students complete homework assignments and to score high marks in board exams.

Tamilnadu Samacheer Kalvi 6th Maths Solutions Term 1 Chapter 4 Geometry Ex 4.4

Miscellaneous Practise Problems

Question 1.
Find the type of lines marked in thick lines (Parallel, intersecting or perpendicular)

Solution:
(i) Parallel lines
(ii) Parallel lines
(iii) Parallel and Perpendicular lines
(iv) Intersecting lines

Question 2.
Find the parallel and intersecting line segments in the picture given below.

Solution:
(a) Parallel line segments

• \(\overline{\mathrm{YZ}} \text { and } \overline{\mathrm{DE}}\)
• \(\overline{\mathrm{EA}} \text { and } \overline{\mathrm{ZV}}\)
• \(\overline{\mathrm{VW}} \text { and } \overline{\mathrm{AB}}\)
• \(\overline{\mathrm{WX}} \text { and } \overline{\mathrm{BC}}\)
• \(\overline{\mathrm{YX}} \text { and } \overline{\mathrm{DC}}\)
• \(\overline{\mathrm{YD}} \text { and } \overline{\mathrm{XC}}\)
• \(\overline{\mathrm{XC}} \text { and } \overline{\mathrm{WB}}\)
• \(\overline{\mathrm{WB}} \text { and } \overline{\mathrm{VA}}\)
• \(\overline{\mathrm{VA}} \text { and } \overline{\mathrm{ZE}}\)
• \(\overline{\mathrm{ZE}} \text { and } \overline{\mathrm{YD}}\)

(b) Intersecting line segments

• DE and ZV
• WX and DC

Question 3.
Name the following angles as shown in the figure.

(i) ∠1 =
(ii) ∠2 =
(iii) ∠3 =
(iv) ∠1 + ∠2 =
(v) ∠2 + ∠3 =
(vi) ∠1 + ∠2 + ∠3 =
Solution:
(i) ∠1 = ∠DBC or ∠CBD
(ii) ∠2 = ∠DBE or ∠EBD
(iii) ∠3 = ∠ABE or ∠EBA
(iv) ∠1 + ∠2 = ∠EBC or ∠CBE
(v) ∠2 + ∠3 = ∠ABD or ∠DHA
(vi) ∠1 + ∠2 + ∠3 = ∠ABC or ∠B or ∠CBA

Question 4.
Measure the angles of the given figures using a protractor and identify the type of angle as acute, obtuse, right or straight.

Solution:
(i) right angle
(ii) acute angle
(iii) straight angle
(iv) obtuse angle

Question 5.
Draw the following angles using the protractor.
(i) 45°
(ii) 120°
(iii) 65°
(iv) 135°
(v) 0°
(vi) 180°
(vii) 38°
(viii) 90°
Solution:

Question 6.
From the figures given below, classify the following pairs of angles into complementary and non-complementary.

Solution:
We know that the two angles are complementary if they add up to 90°.
Therefore (a) (i) is complementary.
In (v) ∠ABC and ∠CBD are complementary
(b) (ii), (iii), (iv) and (v) are non-complementary

Question 7.
From the figures given below, classify the following pairs of angles into supplementary and non supplementary.

Solution:
Ans: (ii) and (iv) are supplementary angles.
(i), and (iii) non-supplementary angles.

Question 8.
From the figure

(i) name a pair of complementary angles.
(ii) name a pair of supplementary angles.
Solution:
(i) ∠FAE; ∠EAD
(ii) ∠FAD; ∠DAC
∠BAC; ∠CAE
∠FAB; ∠BAC
∠FAB; ∠FAE

Question 9.
Find the complementary angle of
(i) 30°
(ii) 26°
(iii) 85°
(iv) 0°
(v) 90°
Solution:

Question 10.
Find the supplementary angle of
(i) 70°
(ii) 35°
(iii) 165°
(iv) 90°
(v) 0°
(vi) 180°
(vii) 95°
Solution:

Challenging Problems

Question 11.
Think and write an object having
(i) Parallel lines (1) ……….. (2) ………. (3) ………..
(ii) Perpendicular lines (1) ……… (2) ……… (3) ………..
(iii) Intersecting lines (1) ……….. (2) ………. (3) ……….
Solution:
(i) Legs of the table, railway tracks, edges of the scale
(ii) Adjacent sides of a Board, Crossbars of windows, Adjacent sides of the textbook
(iii) Crossbars of windows, Ladder, blades of a scissor.

Question 12.
Which angle is equal to twice its complement.
Solution:
Let the angle be x
According to the problem, x = 2 × (90 – x)
x = 180 – 2x
x + 2x = 180
3x = 180
x = \(\frac{180}{3}\)
x = 60
∴ The angle is 60°

Question 13.
Which angle is equal to two-thirds of its supplement.
Solution:
Let the angle be x
According to the problem,
x = \(\frac{2}{3}\) × (180° – x)
3x = 2(180 – x)
3x = 360 – 2x
3x + 2x = 360°
5x = 360°
x = \(\frac{360°}{5}\)
x = 72°
∴ The angle is 72°

Question 14.
Given two angles are supplementary and one angle is 20° more than the other. Find the two angles.
Solution:
Let the angles be x and x + 20°
According to the problem,
x + x + 20 = 180°
2x + 20° = 180°
2x = 180° – 20°
2x = 160°
x = \(\frac{160°}{2}\)
x = 80°
x + 20 = 80° + 20°
= 100°
∴ The two angles are 80° and 100°

Question 15.
Two complementary angles are in ratio 7 : 2. Find the angles.
Solution:
Let the angles be 7x, 2x
According to the problem,
7x + 2x = 90
9x = 90
x = \(\frac{90}{9}\)
x = 10
7x = 7 × 10
= 70
2x = 2 × 10
= 20
∴ Two angles are 70° and 20°

Question 16.
Two supplementary angles are in ratio 5 : 4. Find the angles.
Solution:
Total of two supplementary angles = 180°
Given they are in the ratio 5 : 4
Dividing total angles to 5 + 4 = 9 equal parts.
One angle \(=\frac{5}{9} \times 180=100^{\circ}\)
Another angle \(=\frac{4}{9} \times 180=80^{\circ}\)
Two angles are 100° and 80°.

Students can download Maths Chapter 5 Information Processing Ex 5.2 Questions and Answers, Notes, Samacheer Kalvi 6th Maths Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus, helps students complete homework assignments and to score high marks in board exams.

Tamilnadu Samacheer Kalvi 6th Maths Solutions Term 2 Chapter 5 Information Processing Ex 5.2

Miscellaneous Practice Problems

Question 1.
Write the missing numbers in the trees.

Solution:

Question 2.
Write the missing operations in the trees.

Solution:

Question 3.
Check whether the Tree diagrams are equal or not.

Solution:
c ÷ (a ÷ b), a ÷ (b ÷ c) Not equal

Challenge Problems

Question 4.
Convert ti e following questions into tree diagrams:
(i) The number of people who visited a library in the last 5 months were 1210, 2100, 2550, 3160 and 3310. Draw the tree diagram of the total number of people who had used the library for the 5 months.
(ii) Ram had a bank deposit of Rs. 7,55,250 and he had withdrawn Rs. 5,34,500 for educational purpose. Find the amount left in his account. Draw a tree diagram for this.
(iii) In a cycle factory, 1,600 bicycles were manufactured on a day. Draw tree diagram to find the number of bicycle produced in 20 days.
(iv) A company with 30 employees decided to distribute Rs. 90, 000 as a special bonus equally among its employees. Draw tree diagram to show how much will each receive?
Solution:

Question 5.
Write the numerical expression which gives the answer 10 and also convert into tree diagram.
Solution:

Question 6.
Use brackets in appropriate place to the expression 3 x 8 – 5 which gives 19 and convert it into tree diagram for it.
Solution:

= 3 × 8 – 5
= (3 × 8) – 5 = 24 – 5
= 19

Question 7.
A football team gains 3 and 4 points for successive 2 days and loses 5 points on the third day. Find the total points scored by the team and also represent this in tree diagram.
Solution:

Students can download Maths Chapter 5 Information Processing Ex 5.1 Questions and Answers, Notes, Samacheer Kalvi 6th Maths Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus, helps students complete homework assignments and to score high marks in board exams.

Tamilnadu Samacheer Kalvi 6th Maths Solutions Term 2 Chapter 5 Information Processing Ex 5.1

Question 1.
Convert the following numerical expressions into Tree diagrams
(i) 8 + (6 × 2)
(ii) 9 – (2 × 3)
(iii) (3 × 5) – (4 – 2)
(iv) [(2 × 4) + 2] × (8 – 2)]
(v) [(6 + 4) × 7] – [2 × (10 – 5)]
(vi) [(4 × 3) – 2] + [8 × (5 – 3)]
Solution:

Question 2.
Convert the following tree diagrams into numerical expressions.

Solution:
(i) The numerical Expression is 9 × 8
(ii) The numerical expression is (7 + 6) – 5
(iii) The numerical expression is (8 + 2) – (6 + 1)
(iv) The numerical expression is (5 × 6) – (10 ÷ 2)

Question 3.
Convert the following algebraic expressions into tree diagrams.
(i) 10 v
(ii) 3a – b
(iii) 5x + y
(iv) 20t × p
(v) 2(a + b)
(vi) (x × y) – (y × z)
(vii) 4x + 5y
(viii) (Im – n) ÷ (pq + r)
Solution:

Question 4.
Convert Tree diagrams into Algebraic expressions.

Solution:
(i) Algebraic Expression is p + q
(ii) Algebraic Expression is l – m
(iii) Algebraic Expression is (a × b) – c (or) (ab) – c
(iv) Algebraic Expression is (a + b) – (c + d)
(v) Algebraic Expression is (8 ÷ a) + [ (6 ÷ 4) + 3]

Students can download Maths Chapter 4 Geometry Ex 4.3 Questions and Answers, Notes, Samacheer Kalvi 6th Maths Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus, helps students complete homework assignments and to score high marks in board exams.

Tamilnadu Samacheer Kalvi 6th Maths Solutions Term 2 Chapter 4 Geometry Ex 4.3

Miscellaneous Practice Problems

Question 1.
What are the angles of an isosceles right-angled triangle?
Solution:
Since it is a right-angled triangle
One of the angles is 90°
The other two angles are equal because it is an isosceles triangle.
The other two angles must be 45° and 45°
Angles are 90°, 45°, 45°.

Question 2.
Which of the following correctly describes the given triangle?

(a) It is a right isosceles triangle
(b) It is an acute isosceles triangle
(c) It is an obtuse isosceles triangle
(d) It is an obtuse scalene triangle
Solution:
(c) It is an obtuse isosceles triangle

Question 3.
Which of the following is not possible?
(a) An obtuse isosceles triangle.
(b) An acute isosceles triangle.
(c) An obtuse equilateral triangle.
(d) An acute equilateral triangle.
Solution:
(c) An obtuse equilateral triangle.

Question 4.
If one angle of an isosceles triangle is 124°, then find the other angles
Solution:
In an isosceles triangle, any two sides are equal. Also, the two angles are equal.
Sum of three angles of a triangle = 180°
Given one angle = 124°
Sum of other two angles = 180° – 124° = 56°
Other angles are = \(\frac{56}{2}\) = 28°
28° and 28°.

Question 5.
The diagram shows a square ABCD. If the line segment joins A and C, then mention the type of triangle so formed.
Solution:

Isosceles right-angled triangles

Question 6.
Draw a line segment AB of length 6 cm. At each end of this line segment AB, draw a line perpendicular to the line segment AB. Are these lines parallel?
Solution:

yes, they are parallel

Challenge Problems

Question 7.
Is a triangle possible with the angles 90°, 90°, and 0°, Why?
Solution:
No, a triangle cannot have more than one right angle.

Question 8.
Which of the following statements is true? Why?
(a) Every equilateral triangle is an isosceles triangle.
(b) Every isosceles triangle is an equilateral triangle
Solution:
“(a)” is true, because an isosceles triangle need not have three equal sides

Question 9.
If one angle of an isosceles triangle is 70°, then find the possibilities for the other two angles.
Solution:
(i) Given one angle = 70°
Also, it is an isosceles triangle.
Another one angle also can be 70°.
Sum of these two angles = 70° + 70° = 140°
We know that the sum of three angles in a triangle = 180°.
Third angle = 180° – 140° = 40°
One possibility is 70°, 70°, and 40°
(ii) Also if one angle is 70°
Sum of other two angles = 180° – 70° = 110°
Both are equal. They are \(\frac{110}{2}\) = 55°.
Another possibility is 70°, 55° and 55°.

Question 10.
Which of the following can be the sides of an isosceles triangle?
(a) 6 cm, 3 cm, 3 cm
(b) 5 cm, 2 cm, 2 cm
(c) 6 cm, 6 cm, 7 cm
(d) 4 cm, 4 cm, 8 cm
Solution:
(c) 6 cm, 6 cm, 7 cm

Question 11.
Study the given figure and identify the following triangles,

(a) equilateral triangle
(b) isosceles triangle
(c) scalene triangles
(d) acute triangle
(e) obtuse triangle
(f) right triangle
Solution:(a) BC = 1 + 1 + 1 + 1 = 4 cm
AB = AC = 4 cm
∆ABC is an equilateral triangle.
(b) ∆ABC and ∆AEF are isosceles triangles.
Since AB = AC = 4 cm Also AE = AF.
(c) In a scalene triangle, no two sides are equal.
∆AEB, ∆AED, ∆ADF, ∆AFC, ∆ABD, ∆ADC, ∆ABF, and ∆AEC are scalene triangles.
(d) In an acute-angled triangle all the three angles are less than 90°.
∆ABC, ∆AEF, ∆ABF, and ∆AEC are acute-angled triangles.
(e) In an obtuse-angled triangle any one of the angles is greater than 90°.
∆AEB and ∆AFC are obtuse angled triangles.
(f) In a right triangle, one of the angles is 90°.
∆ADB, ∆ADC, ∆ADE, and ∆ADF are right-angled triangles.

Question 12.
Two sides of the triangle are given in the table. Find the third side of the triangle.

Solution:
(i) between 3 and 11
(ii) between 0 and 16
(iii) between 4 and 11
(iv) between 4 and 24

Question 13.
Complete the following table:

Solution:
(i) Always acute angles
(ii) Acute angle
(iii) Obtuse angle

Students can download Maths Chapter 4 Geometry Ex 4.2 Questions and Answers, Notes, Samacheer Kalvi 6th Maths Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus, helps students complete homework assignments and to score high marks in board exams.

Tamilnadu Samacheer Kalvi 6th Maths Solutions Term 2 Chapter 4 Geometry Ex 4.2

Question 1.
Draw a line segment AB = 7 cm and mark a point P on it. Draw a line perpendicular to the given line segment at P.
Solution:

Step 1 : Draw a line AB = 7 cm and take a point P anywhere on the line.
Step 2 : Place the set square on the line in such a way that the vertex which forms right angle coincides with P and one arm of the right angle coincides with the line AB.
Step 3 : Draw a line PQ through P along the other arm of the right angle of the set square.
Step 4 : The line PQ is perpendicular to the line AB at P. That is, PQ ⊥ AB
∠APQ = ∠BPQ = 90°

Question 2.
Draw a line segment LM = 6.5 cm and mark a point X not lying on it. Using a set square construct a line perpendicular to LM through X.
Solution:

Step 1 : Draw a line LM = 6.5 cm and take a point X anywhere above the line LM.
Step 2 : Place one of the arms of the right angle of a set square along the line LM and the other arm of its right angle touches the point X.
Step 3 : Draw a line through the point X meeting LM at Y.
Step 4 : The line XY is perpendicular to the line LM at Y. That is, LM ⊥ XY.

Question 3.
Find the distance between the given lines using a set square at two different points on each of the pairs of lines and check whether they are parallel.

Solution:
They are parallel

Question 4.
Draw a line segment measuring 7.8 cm. Mark a point B above it at a distance of 5 cm. Through B draw a line parallel to the given segment.
Solution:

Step 1 : Draw a line. Mark two points M and N on the line such that MN = 7.8 cm. Mark a point B any where above the line.
Step 2 : Place the set square below B in such a way that one of the edges that form a right angle lies along MN Place the scale along the other edge of the set square.
Step 3 : Holding the scale firmly, Slide the set square along the edge of the scale until the other edge of the set square reaches the point B. Through B draw a line.
Step 4 : The line MN is parallel to AB. That is, MN || AB.

Question 5.
Draw a line and mark a point R above it at a distance of 5.4 cm Through R draw a line parallel to the given line.
Solution:

Step 1 : Using a scale draw a line AB and mark a point Q on the line.
Step 2 : Place the set square in such a way that the vertex of the right angle coincides with Q and one of the edges of right angle lies along AB. Mark the point R such that QR = 5.4 cm
Step 3 : Place the scale and the set square as shown in the figure.
Step 4 : Hold the scale firmly and slide the set square along the edge of the scale until the other edge touches the point R. Draw a line RS through R.
Step 5 : The line RS is parallel to AB. That is, RS || AB.

Students can download Maths Chapter 2 Integers Ex 2.2 Questions and Answers, Notes, Samacheer Kalvi 6th Maths Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus, helps students complete homework assignments and to score high marks in board exams.

Tamilnadu Samacheer Kalvi 6th Maths Solutions Term 3 Chapter 2 Integers Ex 2.2

Miscellaneous Practice Problems

Question 1.
Write two different real-life situations that represent the integer -3.
Solution:
(i) A sapling planted at a depth of 3m
(ii) Sheela lost ₹ 3 on selling an apple.

Question 2.
Mark the following numbers on a number line.
(i) All integers which are greater than -7 but less than 7.
(ii) The opposite of 3.
(iii) 5 units to the left of -1.
Solution:

Question 3.
Construct a number line that shows the depth of 10 feet from the ground level and its opposite.
Solution:

Question 4.
identify the integers and mark on the number line that are at a distance of 8 units from – 6.
Solution:

Question 5.
Answer the following questions from the number line given below.

(i) Which integer is greater: G or K? Why?
(ii) Find the integer that represents C
(iii) How many integers are there between G and H?
(iv) Find the pairs of letters which are opposite of a number,
(v) Say True or False: 6 units to the left of D is -6.
Solution:
(i) K is greater. K represents -1 and G represents -3. Because it is to the right of G in the negative side of the number line.
(ii) C represents -4
(iii) G represents -3 and H represents 4.
∴ -2, -1, 0, 1, 2, 3 are the 6 numbers between G and H.
(iv) (C, H) and (E, J) are opposite pairs.
(v) False. 6 units to the left of D is 0. Because D represents +6 on the number line

Question 6.
If G is 3 and C is -1, what numbers are A and K on the number line?

Solution:
A (-3), K (7)

Question 7.
Find the integers that are 4 units to the left of 0 and 2 units to the right of -3?
Solution:
-4, -1

Challenge Problems

Question 8.
Is there the smallest and the largest number in the set of integers? Give reason.
Solution:
No, we cannot find the smallest (-) and largest (+) number in the set of integers, as the numbers on the number line extend on both sides without an end.

Question 9.
Look at the Celsius Thermometer and answer the following questions.

(i) What is the temperature that is shown in the Thermometer?
(ii) Where will you mark the temperature 5°C below 0° C in the Thermometer?
(iii) What will be the temperature, if 10° C is reduced from the temperature shown in the Thermometer?
(iv) Mark the opposite of 15° C in the Thermometer.
Solution:
(i) – 10°C
(ii) – 5°C
(iii) -20°C
(iv) -15°C

Question 10.
P, Q, R, and S are four different integers on a number line. From the following clues, find these integers and write them in ascending order.
(i) S is the least of the given integers.
(ii) R is the smallest positive integer.
(iii) The integers P and S are at the same distance from 0.
(iv) Q is 2 units to the left of integer R.
Solution:
S < Q < 0 < R < P

Question 11.
Assuming that the home to be the starting point, mark the following places in order on the number line as per instruction given below and write their corresponding integers.

Places: Home, School, library, Playground, Park, Departmental Store, Bus stand, Railway Station, Post Office, Electricity Board.
Instructions:

1. The bus stand is 3 units to the right of the Home.
2. The library is 2 units to the left of Home.
3. Departmental Store is 6 units to the left of Home.
4. The post office is 1 unit to the right of the Library.
5. Park is 1 unit right of Departmental Store.
6. Railway Station is 3 units left of Post Office.
7. Bus Stand is 8 units to the right of Railway Station.
8. School is next to the right of the Bus Stand.
9. Playground and Library are opposite to each other.
10. Electricity Board and Departmental Store are at equal distance from Home.

Solution:

1. 3
2. -2
3. -6
4. -1
5. -5
6. -4
7. 4
8. 4
9. 5
10. 2

Question 12.
Complete the table using the following hints.

(i) C1 : the first non-negative integer.
(ii) C3 : the opposite to the second negative integer.
(iii) C5 : the additive identity in whole numbers.
(iv) C6 : the successor of the integer in C2.
(v) C8 : the predecessor of the integer in C7.
(vi) C9 : the opposite to the integer in C5.
Solution:
(i) C1 : (0)
(ii) C3 : (2)
(iii) C5 : (0)
(iv) C6 : (-4)
(v) C8 : (-8)
(vi) C9 : (0)

Question 13.
The following bar graph shows the profit (+) and loss (-) of a small scale company (in crores) between the year 2011 to 2017.

(i) Write the integer that represents a profit or a loss for the company in 2014?
(ii) Denote by an integer on the profit or loss in 2016.
(iii) Denote by integers on the loss for the company in 2011 and 2012.
(iv) Say True or False: The loss is minimum in 2012.
(v) Fill in: The amount of loss in 2011 is _____ as profit in 2013.
Solution:
(i) Profit ₹ 45 crores. ∴ Ans : + 45
(ii) In 2016 neither profit nor loss happened. ∴ Ans : 0
(iii) In 2011 loss is 10 crores and in 2012 loss is 20 crores.
∴ -10 and-20.
(iv) False. In 2011 the company’s loss is minimum.
(v) The same. Because in 2013 the profit is 10 crores and in 2011 the loss is 10 crores.